Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1809

Question Number 27853 Answers: 0 Comments: 1

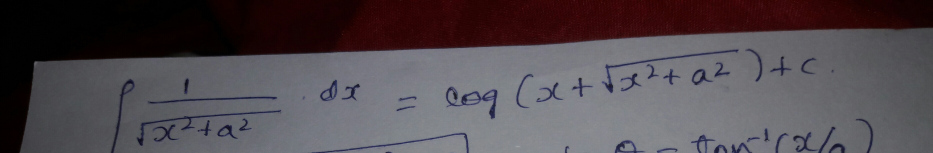
Question Number 27851 Answers: 0 Comments: 2

Question Number 27830 Answers: 0 Comments: 1

lim_(x→∝) (x−lnx)

$$\underset{{x}\rightarrow\propto} {\mathrm{lim}}\:\left({x}−\mathrm{ln}{x}\right) \\ $$

Question Number 27828 Answers: 1 Comments: 2

find the value of ∫_0 ^(π/2) (√(tanx))dx .

$${find}\:{the}\:{value}\:{of}\:\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \sqrt{{tanx}}{dx}\:. \\ $$

Question Number 27821 Answers: 1 Comments: 0

If α and β satisfy sinαcosβ= −(1/2) then the greatest value of 2cosαsinβ

$${If}\:\alpha\:{and}\:\beta\:{satisfy}\:{sin}\alpha{cos}\beta=\:−\frac{\mathrm{1}}{\mathrm{2}}\:{then} \\ $$$${the}\:{greatest}\:{value}\:{of}\:\mathrm{2}{cos}\alpha{sin}\beta \\ $$

Question Number 27820 Answers: 1 Comments: 0

If A,Bε(0,(π/2)) such that 3sin^2 A+2sin^2 B=1 and 3sin2A−2sin2B=0, find the value of A+B.

$${If}\:{A},{B}\epsilon\left(\mathrm{0},\frac{\pi}{\mathrm{2}}\right)\:{such}\:{that}\:\mathrm{3}{sin}^{\mathrm{2}} {A}+\mathrm{2}{sin}^{\mathrm{2}} {B}=\mathrm{1} \\ $$$${and}\:\mathrm{3}{sin}\mathrm{2}{A}−\mathrm{2}{sin}\mathrm{2}{B}=\mathrm{0},\:{find}\:{the}\:{value}\:{of}\:{A}+{B}. \\ $$

Question Number 27818 Answers: 0 Comments: 7

Question Number 27816 Answers: 1 Comments: 1

If N is perfect nth power, prove that n ∣ (d(N)−1) [Where d(N) denotes number of divisors of N] Also show by an example that its vice versa is not necessarily correct.

$$\mathrm{If}\:\mathrm{N}\:\mathrm{is}\:\boldsymbol{\mathrm{perfect}}\:\boldsymbol{\mathrm{nth}}\:\boldsymbol{\mathrm{power}},\:\mathrm{prove}\:\mathrm{that} \\ $$$$\:\:\:\:\:\:\:\mathrm{n}\:\mid\:\left(\mathrm{d}\left(\mathrm{N}\right)−\mathrm{1}\right)\: \\ $$$$\left[{Where}\:\mathrm{d}\left(\mathrm{N}\right)\:{denotes}\:\boldsymbol{{number}}\right. \\ $$$$\left.\boldsymbol{{of}}\:\boldsymbol{{divisors}}\:\boldsymbol{{of}}\:\mathrm{N}\right] \\ $$$$\mathrm{Also}\:\mathrm{show}\:\mathrm{by}\:\mathrm{an}\:\mathrm{example}\:\mathrm{that}\:\mathrm{its} \\ $$$$\mathrm{vice}\:\mathrm{versa}\:\mathrm{is}\:\mathrm{not}\:\mathrm{necessarily}\:\mathrm{correct}. \\ $$

Question Number 27815 Answers: 1 Comments: 0

∫((cos x−cos 2x)/(1−cos x))dx

$$\int\frac{\mathrm{cos}\:\mathrm{x}−\mathrm{cos}\:\mathrm{2x}}{\mathrm{1}−\mathrm{cos}\:\mathrm{x}}\mathrm{dx} \\ $$

Question Number 27809 Answers: 2 Comments: 0

(1) Find the term independent of x in the expansion of (x−(2/x))^(10)

$$\left(\mathrm{1}\right)\:\boldsymbol{\mathrm{Find}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{term}}\:\boldsymbol{\mathrm{independent}} \\ $$$$\boldsymbol{\mathrm{of}}\:\:\:\boldsymbol{\mathrm{x}}\:\:\:\boldsymbol{\mathrm{in}}\:\boldsymbol{\mathrm{the}}\:\:\boldsymbol{\mathrm{expansion}}\:\boldsymbol{\mathrm{of}}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\boldsymbol{\mathrm{x}}−\frac{\mathrm{2}}{\boldsymbol{\mathrm{x}}}\right)^{\mathrm{10}} \\ $$

Question Number 27805 Answers: 0 Comments: 1

find ∫_1 ^∝ ((arctan(αx))/x^2 ) .

$${find}\:\:\int_{\mathrm{1}} ^{\propto} \:\:\frac{{arctan}\left(\alpha{x}\right)}{{x}^{\mathrm{2}} }\:. \\ $$

Question Number 27804 Answers: 0 Comments: 1

calculate ∫_0 ^∝ ((e^(−ax) − e^(−bx) )/x^2 )dx with a>0 b>o

$${calculate}\:\:\int_{\mathrm{0}} ^{\propto} \:\:\frac{{e}^{−{ax}} \:−\:{e}^{−{bx}} }{{x}^{\mathrm{2}} }{dx}\:\:{with}\:{a}>\mathrm{0} \\ $$$${b}>{o} \\ $$

Question Number 27803 Answers: 0 Comments: 0

find the value of ∫_0 ^1 ((arctan(x +x^(−1) ))/(1+x^2 )) dx

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctan}\left({x}\:+{x}^{−\mathrm{1}} \right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx} \\ $$

Question Number 27802 Answers: 0 Comments: 1

find the value of ∫_0 ^∞ (e^(−2x^2 ) /((3+x^2 )^2 ))dx .

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−\mathrm{2}{x}^{\mathrm{2}} } }{\left(\mathrm{3}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx}\:. \\ $$

Question Number 27801 Answers: 0 Comments: 1

solve the d.e. y^′ −2xy =sinx e^x^2 with initial condition y(o)=1.

$${solve}\:{the}\:{d}.{e}.\:\:{y}^{'} \:−\mathrm{2}{xy}\:={sinx}\:{e}^{{x}^{\mathrm{2}} } \:{with}\:{initial}\:{condition} \\ $$$${y}\left({o}\right)=\mathrm{1}. \\ $$

Question Number 27912 Answers: 0 Comments: 2

find the sum of Σ_(n=1) ^∝ (1/n)( ((√2)/(1+i)))^n .

$${find}\:{the}\:{sum}\:{of}\:\sum_{{n}=\mathrm{1}} ^{\propto} \frac{\mathrm{1}}{{n}}\left(\:\:\frac{\sqrt{\mathrm{2}}}{\mathrm{1}+{i}}\right)^{{n}} . \\ $$

Question Number 27797 Answers: 0 Comments: 1

find ∫ (√(2+tan^2 t)) dt.

$${find}\:\:\:\int\:\sqrt{\mathrm{2}+{tan}^{\mathrm{2}} {t}}\:\:{dt}. \\ $$

Question Number 27796 Answers: 0 Comments: 0

find ∫ (x^2 /((cosx +x sinx)^2 )) .

$${find}\:\:\int\:\:\:\frac{{x}^{\mathrm{2}} }{\left({cosx}\:+{x}\:{sinx}\right)^{\mathrm{2}} }\:\:. \\ $$

Question Number 27794 Answers: 0 Comments: 0

let give I(x)= ∫_0 ^π ln (1−2x cost +x^2 )dt by using the polynomial p(x)= (z+x)^(2n) −1 find the value of I(x).

$${let}\:{give}\:\:{I}\left({x}\right)=\:\int_{\mathrm{0}} ^{\pi} {ln}\:\left(\mathrm{1}−\mathrm{2}{x}\:{cost}\:+{x}^{\mathrm{2}} \right){dt}\:{by}\:{using}\:{the} \\ $$$${polynomial}\:{p}\left({x}\right)=\:\left({z}+{x}\right)^{\mathrm{2}{n}} −\mathrm{1}\:\:{find}\:{the}\:{value}\:{of}\:{I}\left({x}\right). \\ $$

Question Number 27792 Answers: 0 Comments: 3

find lim_(x−>1) ∫_x ^x^2 (dt/(ln(t))) .

$${find}\:{lim}_{{x}−>\mathrm{1}} \int_{{x}} ^{{x}^{\mathrm{2}} } \:\:\frac{{dt}}{{ln}\left({t}\right)}\:\:. \\ $$

Question Number 27790 Answers: 0 Comments: 2

let give f(x)= x^(n−1) ln(1+x) with n fromN^∗ find f^((n)) (x)

$${let}\:{give}\:\:{f}\left({x}\right)=\:{x}^{{n}−\mathrm{1}} \:{ln}\left(\mathrm{1}+{x}\right)\:\:{with}\:{n}\:{fromN}^{\ast} \:\:\:{find}\:{f}^{\left({n}\right)} \left({x}\right) \\ $$

Question Number 27789 Answers: 0 Comments: 3

find lim_(x−>0) (1+sinx)^(1/x) .

$${find}\:\:{lim}_{{x}−>\mathrm{0}} \left(\mathrm{1}+{sinx}\right)^{\frac{\mathrm{1}}{{x}}} \:\:. \\ $$

Question Number 27788 Answers: 0 Comments: 0

find the value of A_n = ∫_0 ^π ((sin(nt))/(sint))dt with n∈N^∗ .

$${find}\:{the}\:{value}\:{of}\:\:\:{A}_{{n}} =\:\int_{\mathrm{0}} ^{\pi} \:\:\frac{{sin}\left({nt}\right)}{{sint}}{dt}\:\:{with}\:{n}\in{N}^{\ast} \:. \\ $$

Question Number 27787 Answers: 1 Comments: 0

find lim_(x−>0) ((e^x −x−1)/x^2 ) .

$${find}\:\:{lim}_{{x}−>\mathrm{0}} \frac{{e}^{{x}} \:\:−{x}−\mathrm{1}}{{x}^{\mathrm{2}} }\:. \\ $$

Question Number 27786 Answers: 0 Comments: 1

find lim_(x−>0) (((tanx)/x))^(1/x^2 ) .

$${find}\:{lim}_{{x}−>\mathrm{0}} \:\left(\frac{{tanx}}{{x}}\right)^{\frac{\mathrm{1}}{{x}^{\mathrm{2}} }} . \\ $$

Question Number 27785 Answers: 0 Comments: 1

find nature of the serie Σ_(n=1) ^∝ ξ(n) x^n with ξ(x)= Σ_(n=1) ^∝ (1/n^x ) and x>1 .

$${find}\:{nature}\:{of}\:{the}\:{serie}\:\sum_{{n}=\mathrm{1}} ^{\propto} \:\:\xi\left({n}\right)\:{x}^{{n}} \\ $$$${with}\:\xi\left({x}\right)=\:\sum_{{n}=\mathrm{1}} ^{\propto} \:\:\:\frac{\mathrm{1}}{{n}^{{x}} }\:\:\:\:\:{and}\:{x}>\mathrm{1}\:. \\ $$

Pg 1804 Pg 1805 Pg 1806 Pg 1807 Pg 1808 Pg 1809 Pg 1810 Pg 1811 Pg 1812 Pg 1813

Contact: info@tinkutara.com