Question and Answers Forum

AllQuestion and Answers: Page 1807

Question Number 28067 Answers: 0 Comments: 0

let give f(x)= (1/(2+cosx)) fonction 2π periodic even. developp f at fourier series.

$${let}\:{give}\:{f}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{2}+{cosx}}\:\:\:{fonction}\:\mathrm{2}\pi\:{periodic}\:{even}. \\ $$$${developp}\:{f}\:\:{at}\:{fourier}\:{series}. \\ $$

Question Number 28050 Answers: 0 Comments: 14

Question Number 28044 Answers: 0 Comments: 8

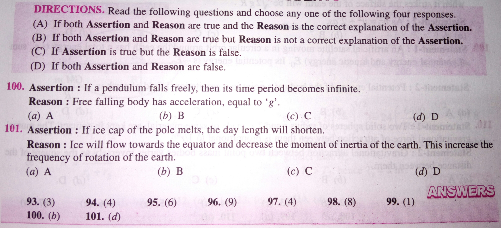
Question Number 28041 Answers: 0 Comments: 0

∫(ϰ^2 /((ϰsinϰ+cosϰ)^2 ))d(ϰ)

$$\int\frac{\varkappa^{\mathrm{2}} }{\left(\varkappa\mathrm{sin}\varkappa+\mathrm{cos}\varkappa\right)^{\mathrm{2}} }\mathrm{d}\left(\varkappa\right) \\ $$

Question Number 28035 Answers: 0 Comments: 2

find the value of ∫_0 ^∞ x((arctan(2x))/((2+x^2 )^2 ))dx .

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:{x}\frac{{arctan}\left(\mathrm{2}{x}\right)}{\left(\mathrm{2}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx}\:. \\ $$

Question Number 28034 Answers: 0 Comments: 0

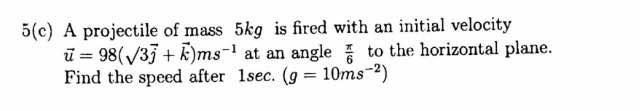
Question Number 28033 Answers: 0 Comments: 1

1) find the value of ∫_0 ^∞ ((ln(x))/(1+x^2 )) dx 2) find the value of ∫_0 ^∞ ((xln(x))/((1+x^2 )^2 ))dx .

$$\left.\mathrm{1}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{ln}\left({x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx} \\ $$$$\left.\mathrm{2}\right)\:\:{find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{xln}\left({x}\right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx}\:. \\ $$

Question Number 28027 Answers: 1 Comments: 0

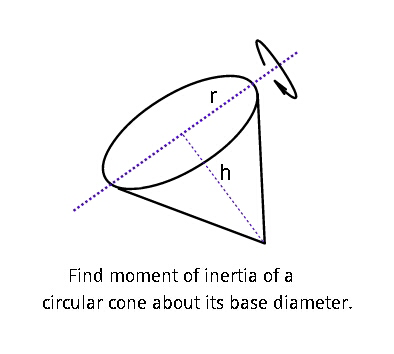
Question Number 36365 Answers: 1 Comments: 2

Question Number 36366 Answers: 3 Comments: 0

Question Number 28015 Answers: 1 Comments: 1

Question Number 28006 Answers: 0 Comments: 1

∫_0 ^∞ (ln x)^(−3) dx

$$\int_{\mathrm{0}} ^{\infty} \left(\mathrm{ln}\:{x}\right)^{−\mathrm{3}} {dx} \\ $$

Question Number 27999 Answers: 0 Comments: 1

find I_(n,m) = ∫_0 ^1 x^n (1−x)^m dx with (n,m)∈N^★^2 and calculate Σ_(n=0) ^∝ I_(n,m) .

$${find}\:\:{I}_{{n},{m}} \:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{{n}} \:\left(\mathrm{1}−{x}\right)^{{m}} \:{dx}\:{with} \\ $$$$\left({n},{m}\right)\in{N}^{\bigstar^{\mathrm{2}} } \:{and}\:{calculate}\:\:\sum_{{n}=\mathrm{0}} ^{\propto} \:{I}_{{n},{m}} . \\ $$

Question Number 27997 Answers: 1 Comments: 3

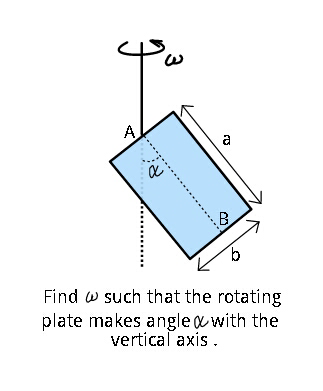
Question Number 27996 Answers: 1 Comments: 0

p,q are two natural number and ((p^6 +2p^4 +4p^2 )/(p^9 −8p^3 ))−(1/(4q))=(5/(6q)), then find the minimum possible value of p+q

$$\mathrm{p},\mathrm{q}\:\mathrm{are}\:\mathrm{two}\:\mathrm{natural}\:\mathrm{number}\:\mathrm{and}\: \\ $$$$\:\frac{\mathrm{p}^{\mathrm{6}} +\mathrm{2p}^{\mathrm{4}} +\mathrm{4p}^{\mathrm{2}} }{\mathrm{p}^{\mathrm{9}} −\mathrm{8p}^{\mathrm{3}} }−\frac{\mathrm{1}}{\mathrm{4q}}=\frac{\mathrm{5}}{\mathrm{6q}}, \\ $$$$\mathrm{then}\:\mathrm{find}\:\mathrm{the}\:\mathrm{minimum} \\ $$$$\mathrm{possible}\:\mathrm{value}\:\mathrm{of}\:\mathrm{p}+\mathrm{q} \\ $$

Question Number 27986 Answers: 0 Comments: 1

Prove that the angular momentum H_G ^ of a rigid body about its mass center is given by : H_x =I_x ^ ω_x −I_(xy) ^ ω_y −I_(xz) ^ ω_z H_y =−I_(yx) ^ ω_x +I_y ^ ω_y −I_(yz) ^ ω_z H_z =−I_(zx) ^ ω_x −I_(zy) ^ ω_y +I_z ^ ω_z where I_x ^ =∫(y^2 +z^2 )dm I_(xy) ^ =∫xy dm ...and so on..

$${Prove}\:{that}\:{the}\:{angular}\:{momentum} \\ $$$$\bar {\boldsymbol{{H}}}_{{G}} \:{of}\:{a}\:{rigid}\:{body}\:{about}\:{its}\:{mass} \\ $$$${center}\:{is}\:{given}\:{by}\:: \\ $$$${H}_{{x}} =\bar {{I}}_{{x}} \omega_{{x}} −\bar {{I}}_{{xy}} \omega_{{y}} −\bar {{I}}_{{xz}} \omega_{{z}} \\ $$$${H}_{{y}} =−\bar {{I}}_{{yx}} \omega_{{x}} +\bar {{I}}_{{y}} \omega_{{y}} −\bar {{I}}_{{yz}} \omega_{{z}} \\ $$$${H}_{{z}} =−\bar {{I}}_{{zx}} \omega_{{x}} −\bar {{I}}_{{zy}} \omega_{{y}} +\bar {{I}}_{{z}} \omega_{{z}} \\ $$$$\:\:\:\:{where}\:\:\bar {{I}}_{{x}} =\int\left({y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right){dm} \\ $$$$\:\:\:\:\:\:\bar {{I}}_{{xy}} =\int{xy}\:{dm}\:...{and}\:{so}\:{on}.. \\ $$

Question Number 27983 Answers: 2 Comments: 0

1) find two factors of 1000001 other than 1 and 1000001 2)(x^2 −5x+5)^((x^2 +2x−24)) =1 what is the value of the product of the solutions?

$$\left.\mathrm{1}\right)\:\mathrm{find}\:\mathrm{two}\:\:\mathrm{factors}\:\mathrm{of}\:\mathrm{1000001}\:\mathrm{other}\:\mathrm{than}\:\mathrm{1}\:\mathrm{and}\:\mathrm{1000001} \\ $$$$\left.\mathrm{2}\right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{5x}+\mathrm{5}\right)^{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2x}−\mathrm{24}\right)} =\mathrm{1}\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{value}\:\:\mathrm{of}\:\mathrm{the}\:\mathrm{product}\:\mathrm{of}\:\mathrm{the}\:\mathrm{solutions}? \\ $$

Question Number 28012 Answers: 1 Comments: 1

1,4,5,16,17,20....... what is the 68th term in this sequnce?

$$\mathrm{1},\mathrm{4},\mathrm{5},\mathrm{16},\mathrm{17},\mathrm{20}.......\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{68th}\:\mathrm{term}\:\mathrm{in}\:\mathrm{this}\:\mathrm{sequnce}? \\ $$

Question Number 27977 Answers: 1 Comments: 0

∣2x+1∣≤2

$$\mid\mathrm{2}{x}+\mathrm{1}\mid\leqslant\mathrm{2} \\ $$

Question Number 27976 Answers: 1 Comments: 1

solve ((2x)/(x^2 +1))<((3x+1)/(2(x^2 +1)))