Question and Answers Forum

AllQuestion and Answers: Page 1806

Question Number 28929 Answers: 1 Comments: 0

If T=2π((L/g))^(1/(2 )) and L=100±0.1 cm(limit standard error) T=2.01±0.01 s (limit standard error) Calculate the value of g and its standard error.

$${If}\:{T}=\mathrm{2}\pi\left(\frac{{L}}{{g}}\right)^{\frac{\mathrm{1}}{\mathrm{2}\:}} \:{and} \\ $$$${L}=\mathrm{100}\pm\mathrm{0}.\mathrm{1}\:{cm}\left({limit}\:{standard}\:\right. \\ $$$$\left.{error}\right) \\ $$$${T}=\mathrm{2}.\mathrm{01}\pm\mathrm{0}.\mathrm{01}\:{s}\:\left({limit}\:{standard}\right. \\ $$$$\left.{error}\right) \\ $$$${Calculate}\:{the}\:{value}\:{of}\:{g}\:{and}\:{its} \\ $$$${standard}\:{error}. \\ $$

Question Number 28930 Answers: 0 Comments: 1

Question Number 28921 Answers: 1 Comments: 0

Question Number 28940 Answers: 1 Comments: 1

Question Number 28911 Answers: 1 Comments: 1

Question Number 28903 Answers: 0 Comments: 1

Question Number 28902 Answers: 0 Comments: 0

Question Number 28894 Answers: 1 Comments: 5

Question Number 28905 Answers: 1 Comments: 0

A body rolls down a slope from a height of 100m. the velocity at the foot of the slope is 20 m/s. What percentage of the P.E is converted in K.E ? Answer: 20%

$$\mathrm{A}\:\mathrm{body}\:\mathrm{rolls}\:\mathrm{down}\:\mathrm{a}\:\mathrm{slope}\:\mathrm{from}\:\mathrm{a}\:\mathrm{height}\:\mathrm{of}\:\:\mathrm{100m}.\:\mathrm{the}\:\mathrm{velocity}\:\mathrm{at}\:\mathrm{the}\:\mathrm{foot}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{slope}\:\mathrm{is}\:\:\mathrm{20}\:\mathrm{m}/\mathrm{s}.\:\:\mathrm{What}\:\mathrm{percentage}\:\mathrm{of}\:\mathrm{the}\:\boldsymbol{\mathrm{P}}.\boldsymbol{\mathrm{E}}\:\:\mathrm{is}\:\mathrm{converted}\:\mathrm{in}\:\:\boldsymbol{\mathrm{K}}.\boldsymbol{\mathrm{E}}\:\:? \\ $$$$ \\ $$$$\boldsymbol{\mathrm{A}}\mathrm{nswer}:\:\:\:\:\:\mathrm{20\%} \\ $$

Question Number 28892 Answers: 2 Comments: 0

find lim_(x→0) (1/x)ln(((e^x −1)/x)) .

$${find}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:\frac{\mathrm{1}}{{x}}{ln}\left(\frac{{e}^{{x}} −\mathrm{1}}{{x}}\right)\:. \\ $$

Question Number 28891 Answers: 1 Comments: 0

let give u_(n,k) = (1/(n+1)) +(1/(n+2)) +.... (1/(kn)) k integr fixed ≥2 find lim_(n→+ ∞) u_(n,k) .

$${let}\:{give}\:{u}_{{n},{k}} =\:\frac{\mathrm{1}}{{n}+\mathrm{1}}\:+\frac{\mathrm{1}}{{n}+\mathrm{2}}\:+....\:\frac{\mathrm{1}}{{kn}}\:\:\:{k}\:{integr}\:{fixed}\:\geqslant\mathrm{2} \\ $$$${find}\:{lim}_{{n}\rightarrow+\:\:\infty} {u}_{{n},{k}} . \\ $$

Question Number 28890 Answers: 0 Comments: 0

1) prove that ∀ x≥0 x −(x^2 /2)≤ln(1+x)≤x 2) find lim_(n→+∞) Π_(k=1) ^n (1 + (1/(k^2 +n^2 )))^n .

$$\left.\mathrm{1}\right)\:{prove}\:{that}\:\forall\:{x}\geqslant\mathrm{0}\:\:\:{x}\:−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\leqslant{ln}\left(\mathrm{1}+{x}\right)\leqslant{x} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{lim}_{{n}\rightarrow+\infty} \:\:\:\prod_{{k}=\mathrm{1}} ^{{n}} \left(\mathrm{1}\:+\:\frac{\mathrm{1}}{{k}^{\mathrm{2}} +{n}^{\mathrm{2}} }\right)^{{n}} . \\ $$

Question Number 28889 Answers: 0 Comments: 3

find I = ∫_0 ^(2π) ln(x−e^(iθ) )dθ and xfromR and x^2 ≠1.

$${find}\:\:{I}\:\:=\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} {ln}\left({x}−{e}^{{i}\theta} \right){d}\theta\:\:\:\:{and}\:{xfromR}\:{and}\:{x}^{\mathrm{2}} \neq\mathrm{1}. \\ $$

Question Number 28888 Answers: 0 Comments: 0

find I_n = ∫_0 ^π (dx/(1+cos^2 (nx))) with n∈ N^★ .

$${find}\:\:{I}_{{n}} =\:\int_{\mathrm{0}} ^{\pi} \:\:\:\frac{{dx}}{\mathrm{1}+{cos}^{\mathrm{2}} \left({nx}\right)}\:{with}\:{n}\in\:{N}^{\bigstar} . \\ $$

Question Number 28887 Answers: 0 Comments: 2

find ∫ arcsin((√(x/(x+2))))dx.

$${find}\:\int\:\:{arcsin}\left(\sqrt{\frac{{x}}{{x}+\mathrm{2}}}\right){dx}. \\ $$

Question Number 28886 Answers: 1 Comments: 0

find ∫ (x/(cos^2 x))dx.

$${find}\:\int\:\:\:\frac{{x}}{{cos}^{\mathrm{2}} {x}}{dx}. \\ $$

Question Number 28885 Answers: 0 Comments: 1

find ∫_(−1) ^1 (dt/(t +(√(1+t^2 )))) .

$${find}\:\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\:\:\:\frac{{dt}}{{t}\:+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}\:. \\ $$

Question Number 28884 Answers: 0 Comments: 0

find ∫_0 ^(π/2) cost ln(tant)dt.

$${find}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:{cost}\:{ln}\left({tant}\right){dt}. \\ $$

Question Number 28883 Answers: 0 Comments: 1

find ∫_0 ^∞ (dt/((1+t^2 )^4 ))

$${find}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{4}} } \\ $$

Question Number 28882 Answers: 0 Comments: 2

find ∫_(−π) ^π ((2dt)/(2+sint +cost)) .

$${find}\:\int_{−\pi} ^{\pi} \:\:\:\frac{\mathrm{2}{dt}}{\mathrm{2}+{sint}\:+{cost}}\:. \\ $$

Question Number 28881 Answers: 1 Comments: 0

find ∫_(−∞) ^(+∞) (dt/(t^2 +2t+2))

$${find}\:\int_{−\infty} ^{+\infty} \:\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{2}{t}+\mathrm{2}} \\ $$

Question Number 28879 Answers: 0 Comments: 0

find the value of Σ_(n=1) ^(+∞) ((cos(nπx))/n^2 ) with 0<x<1.

$${find}\:{the}\:{value}\:{of}\:\sum_{{n}=\mathrm{1}} ^{+\infty} \:\:\frac{{cos}\left({n}\pi{x}\right)}{{n}^{\mathrm{2}} }\:{with}\:\:\mathrm{0}<{x}<\mathrm{1}. \\ $$

Question Number 28876 Answers: 0 Comments: 1

Evaluate (i) lim_(x→−∞) ((2−3x)/(√(3+4x^2 ))) (ii) lim_(x→+∞) ((2−3x)/(√(3+4x^2 )))

$$\mathcal{E}{valuate} \\ $$$$\left(\mathrm{i}\right)\:\:\underset{{x}\rightarrow−\infty} {{lim}}\:\:\frac{\mathrm{2}−\mathrm{3x}}{\sqrt{\mathrm{3}+\mathrm{4x}^{\mathrm{2}} }} \\ $$$$\left(\mathrm{ii}\right)\:\:\underset{{x}\rightarrow+\infty} {{lim}}\:\:\frac{\mathrm{2}−\mathrm{3x}}{\sqrt{\mathrm{3}+\mathrm{4x}^{\mathrm{2}} }} \\ $$

Question Number 28858 Answers: 0 Comments: 1

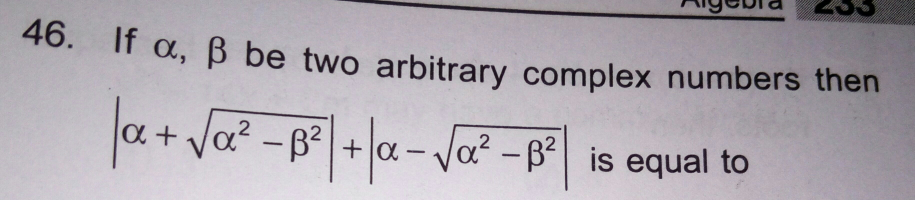
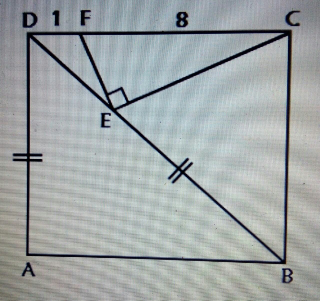
Question Number 28857 Answers: 1 Comments: 0

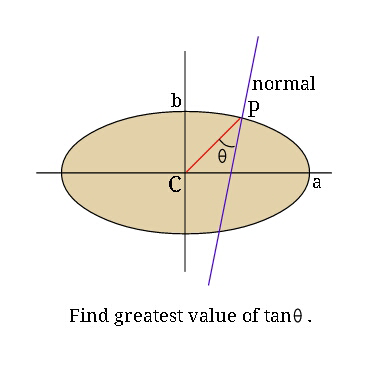
Question Number 28856 Answers: 0 Comments: 0

Pg 1801 Pg 1802 Pg 1803 Pg 1804 Pg 1805 Pg 1806 Pg 1807 Pg 1808 Pg 1809 Pg 1810

Contact: info@tinkutara.com