
AllQuestion and Answers: Page 1802
Question Number 28449 Answers: 2 Comments: 0

Question Number 28446 Answers: 0 Comments: 0
Question Number 28445 Answers: 0 Comments: 0
Question Number 28444 Answers: 0 Comments: 0
Question Number 28442 Answers: 0 Comments: 0
Question Number 28688 Answers: 0 Comments: 0

Question Number 28439 Answers: 0 Comments: 0
$${find}\:\:\int\:\:\frac{\mathrm{1}+{tanx}}{\mathrm{1}+{sin}^{\mathrm{2}} {x}}{dx} \\ $$
Question Number 28438 Answers: 0 Comments: 0
Question Number 28437 Answers: 0 Comments: 0
Question Number 28436 Answers: 0 Comments: 0
Question Number 28435 Answers: 0 Comments: 0
Question Number 28434 Answers: 0 Comments: 0
Question Number 28433 Answers: 0 Comments: 0
Question Number 28432 Answers: 0 Comments: 1
Question Number 28431 Answers: 0 Comments: 0
Question Number 28430 Answers: 0 Comments: 1
Question Number 28429 Answers: 0 Comments: 1
Question Number 28428 Answers: 0 Comments: 1
Question Number 28427 Answers: 1 Comments: 1
Question Number 28426 Answers: 0 Comments: 0
Question Number 28417 Answers: 0 Comments: 0
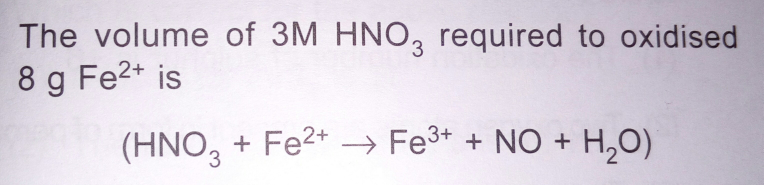
Question Number 28413 Answers: 0 Comments: 0
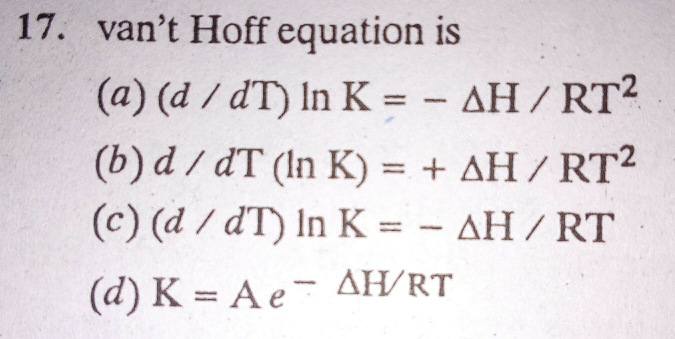
Question Number 28408 Answers: 0 Comments: 0
Question Number 28406 Answers: 1 Comments: 1
$${Image}\:{not}\:{getting}\:{attached}.\:{Please} \\ $$$${see}\:{the}\:{link}\:{below}. \\ $$
Question Number 28399 Answers: 1 Comments: 0

Question Number 28411 Answers: 2 Comments: 1

Pg 1797 Pg 1798 Pg 1799 Pg 1800 Pg 1801 Pg 1802 Pg 1803 Pg 1804 Pg 1805 Pg 1806
