
AllQuestion and Answers: Page 1801
Question Number 28533 Answers: 0 Comments: 1
Question Number 28532 Answers: 0 Comments: 1
Question Number 28531 Answers: 0 Comments: 0
Question Number 28530 Answers: 0 Comments: 0
Question Number 28529 Answers: 0 Comments: 2
Question Number 28540 Answers: 0 Comments: 0
Question Number 28518 Answers: 1 Comments: 2

Question Number 28516 Answers: 2 Comments: 1

Question Number 28515 Answers: 1 Comments: 0

Question Number 28512 Answers: 1 Comments: 1

Question Number 28508 Answers: 1 Comments: 1

Question Number 28506 Answers: 0 Comments: 3
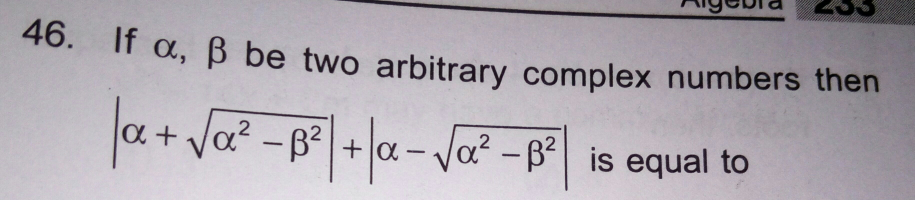
Question Number 28505 Answers: 2 Comments: 0

Question Number 28501 Answers: 2 Comments: 1
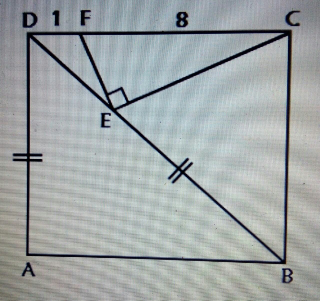
Question Number 28504 Answers: 0 Comments: 0
Question Number 28490 Answers: 2 Comments: 0
Question Number 28483 Answers: 0 Comments: 0

Question Number 28481 Answers: 0 Comments: 1

Question Number 28480 Answers: 1 Comments: 1

Question Number 28479 Answers: 1 Comments: 0

Question Number 28475 Answers: 0 Comments: 0

Question Number 28468 Answers: 0 Comments: 0
Question Number 28467 Answers: 2 Comments: 1

Question Number 28464 Answers: 1 Comments: 0
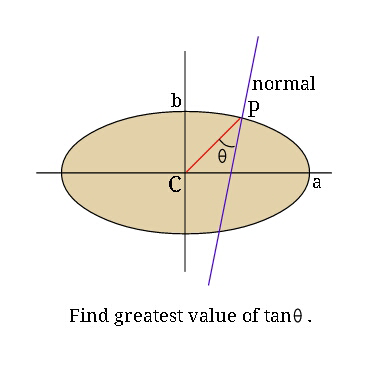
Question Number 28461 Answers: 0 Comments: 2

Question Number 28456 Answers: 1 Comments: 1

Pg 1796 Pg 1797 Pg 1798 Pg 1799 Pg 1800 Pg 1801 Pg 1802 Pg 1803 Pg 1804 Pg 1805
