Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1800

Question Number 28600 Answers: 0 Comments: 4

Question Number 28597 Answers: 1 Comments: 1

Question Number 28591 Answers: 1 Comments: 0

What is the difference between angular frequency and angular velocity?

$${What}\:{is}\:{the}\:{difference}\:{between} \\ $$$${angular}\:{frequency}\:{and}\:{angular} \\ $$$${velocity}? \\ $$

Question Number 28583 Answers: 1 Comments: 1

Question Number 28574 Answers: 1 Comments: 2

Question Number 28586 Answers: 0 Comments: 0

When dry chlorine is passed thru silver chlorate heated to 90°C then which of the oxide of chlorine is formed and why?

$$\mathrm{When}\:\mathrm{dry}\:\mathrm{chlorine}\:\mathrm{is}\:\mathrm{passed}\:\mathrm{thru} \\ $$$$\mathrm{silver}\:\mathrm{chlorate}\:\mathrm{heated}\:\mathrm{to}\:\mathrm{90}°\mathrm{C} \\ $$$$\mathrm{then}\:\mathrm{which}\:\mathrm{of}\:\mathrm{the}\:\mathrm{oxide}\:\mathrm{of}\:\mathrm{chlorine} \\ $$$$\mathrm{is}\:\mathrm{formed}\:\mathrm{and}\:\mathrm{why}? \\ $$

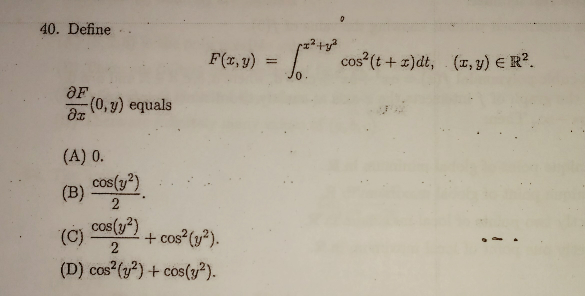
Question Number 28585 Answers: 1 Comments: 0

Question Number 28565 Answers: 1 Comments: 0

Question Number 28554 Answers: 2 Comments: 0

(((a − b))/((c − d))) = 3 (((a − c))/((b − d))) = 4 (((a − d))/((b − c))) = ?

$$\frac{\left({a}\:−\:{b}\right)}{\left({c}\:−\:{d}\right)}\:\:=\:\:\mathrm{3} \\ $$$$\frac{\left({a}\:−\:{c}\right)}{\left({b}\:−\:{d}\right)}\:\:=\:\:\mathrm{4} \\ $$$$\frac{\left({a}\:−\:{d}\right)}{\left({b}\:−\:{c}\right)}\:\:=\:\:? \\ $$$$ \\ $$

Question Number 28547 Answers: 1 Comments: 0

Question Number 28546 Answers: 0 Comments: 0

let give w=e^(i((2π)/n)) and S= Σ_(k=0) ^(n−1) w^k^2 1) prove that S= Σ_(k=0) ^(n−1) w^((q+k)^2 ) 2) find ∣S∣.

$${let}\:{give}\:{w}={e}^{{i}\frac{\mathrm{2}\pi}{{n}}} \:\:\:{and}\:\:{S}=\:\sum_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \:\:{w}^{{k}^{\mathrm{2}} } \\ $$$$\left.\mathrm{1}\right)\:{prove}\:{that}\:\:\:{S}=\:\sum_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \:\:{w}^{\left({q}+{k}\right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{2}\right)\:{find}\:\mid{S}\mid. \\ $$

Question Number 28544 Answers: 0 Comments: 2

if a_1 ,a_2 ,...a_(14 ) are roots of the polynomial p(x)=x^(14) +x^8 2x+1 calculate Σ_(i=1) ^(14) (1/((a_i −1)^2 )) .

$${if}\:\:{a}_{\mathrm{1}} \:,{a}_{\mathrm{2}} ,...{a}_{\mathrm{14}\:} {are}\:{roots}\:{of}\:{the}\:{polynomial} \\ $$$${p}\left({x}\right)={x}^{\mathrm{14}} +{x}^{\mathrm{8}} \:\mathrm{2}{x}+\mathrm{1}\:\:\:{calculate}\:\:\sum_{{i}=\mathrm{1}} ^{\mathrm{14}} \:\:\frac{\mathrm{1}}{\left({a}_{{i}} −\mathrm{1}\right)^{\mathrm{2}} }\:\:. \\ $$

Question Number 28543 Answers: 0 Comments: 0

find the value of ∫_(−∞) ^(+∞) (dt/((t^2 +t +h^2 )^2 +h^2 )) .

$${find}\:{the}\:{value}\:{of}\:\:\int_{−\infty} ^{+\infty} \:\:\:\:\:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} +{t}\:+{h}^{\mathrm{2}} \right)^{\mathrm{2}} \:+{h}^{\mathrm{2}} }\:\:\:. \\ $$

Question Number 28542 Answers: 0 Comments: 0

if (1/(1−x−y−xy)) = Σ_(n=0) ^∞ g_n (y) x^n find g_n .

$${if}\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{1}−{x}−{y}−{xy}}\:=\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\:{g}_{{n}} \left({y}\right)\:{x}^{{n}} \:\:{find}\:{g}_{{n}} \:\:. \\ $$

Question Number 28541 Answers: 0 Comments: 0

prove that ∫_0 ^∞ ((sinx)/(e^(ax) −1))dx= Σ_(p=1) ^∞ (1/(1+p^2 a^2 )) with a>0

$${prove}\:{that}\:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{sinx}}{{e}^{{ax}} −\mathrm{1}}{dx}=\:\sum_{{p}=\mathrm{1}} ^{\infty} \:\:\frac{\mathrm{1}}{\mathrm{1}+{p}^{\mathrm{2}} {a}^{\mathrm{2}} }\:\:\:\:\:{with}\:{a}>\mathrm{0} \\ $$

Question Number 28539 Answers: 0 Comments: 0

find the value of ∫_0 ^∞ ((1−cos(xt))/t^2 ) e^(−t) dt .

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{1}−{cos}\left({xt}\right)}{{t}^{\mathrm{2}} }\:{e}^{−{t}} {dt}\:. \\ $$

Question Number 28538 Answers: 0 Comments: 0

study the convergence of u_(n ) =Σ_(k=0) ^n (1/(√(1+k^2 ))) −argsh(n) and v_n = Σ_(k=0) ^n (1/(√(1+k^2 ))) .

$${study}\:{the}\:{convergence}\:{of} \\ $$$${u}_{{n}\:} \:\:=\sum_{{k}=\mathrm{0}} ^{{n}} \:\:\frac{\mathrm{1}}{\sqrt{\mathrm{1}+{k}^{\mathrm{2}} }}\:\:−{argsh}\left({n}\right)\:\:{and} \\ $$$${v}_{{n}} =\:\sum_{{k}=\mathrm{0}} ^{{n}} \:\:\frac{\mathrm{1}}{\sqrt{\mathrm{1}+{k}^{\mathrm{2}} }}\:\:. \\ $$$$ \\ $$

Question Number 28537 Answers: 0 Comments: 0

prove that ∀ n∈ N (1/(√(1+(n+1)^2 ))) ≤ argsh(n+1) −argsh(n)≤ (1/(√(1+n^2 ))) .

$${prove}\:{that}\:\:\:\forall\:{n}\in\:\mathbb{N} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{1}+\left({n}+\mathrm{1}\right)^{\mathrm{2}} }}\:\leqslant\:{argsh}\left({n}+\mathrm{1}\right)\:−{argsh}\left({n}\right)\leqslant\:\frac{\mathrm{1}}{\sqrt{\mathrm{1}+{n}^{\mathrm{2}} }}\:. \\ $$

Question Number 28536 Answers: 0 Comments: 0

study the convergence of V_n = Π_(1≤p≤n) (1 +(i/p)) i∈ C and i^2 =−1 .

$${study}\:{the}\:{convergence}\:{of}\:\:{V}_{{n}} =\:\prod_{\mathrm{1}\leqslant{p}\leqslant{n}} \left(\mathrm{1}\:+\frac{{i}}{{p}}\right) \\ $$$${i}\in\:{C}\:{and}\:{i}^{\mathrm{2}} =−\mathrm{1}\:. \\ $$

Question Number 28535 Answers: 0 Comments: 0

study the convergence of U_n = (1+(z/n))^n with z∈C .

$${study}\:{the}\:{convergence}\:{of}\:\:{U}_{{n}} =\:\left(\mathrm{1}+\frac{{z}}{{n}}\right)^{{n}} \:{with}\:{z}\in{C}\:. \\ $$

Question Number 28534 Answers: 0 Comments: 1

find n from N in ordre tohave x^2 +x+1 divide (x+1)^n −x^n −1.

$${find}\:{n}\:{from}\:{N}\:\:{in}\:{ordre}\:{tohave}\:{x}^{\mathrm{2}} +{x}+\mathrm{1}\:{divide} \\ $$$$\left({x}+\mathrm{1}\right)^{{n}} −{x}^{{n}} −\mathrm{1}. \\ $$

Question Number 28533 Answers: 0 Comments: 1

let give the matrice A= (((1 2 )),((2 1)) ) calculate A^n then find e^A .

$${let}\:{give}\:{the}\:{matrice}\:\:{A}=\:\begin{pmatrix}{\mathrm{1}\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}}\end{pmatrix} \\ $$$${calculate}\:\:{A}^{{n}} \:\:{then}\:{find}\:\:{e}^{{A}} \:. \\ $$

Question Number 28532 Answers: 0 Comments: 1

let give A_n = ( C_n ^0 .C_n ^1 ....C_n ^n )^(1/(n+1)) find^n (√A) _n .

$${let}\:{give}\:\:{A}_{{n}} =\:\left(\:{C}_{{n}} ^{\mathrm{0}} \:.{C}_{{n}} ^{\mathrm{1}} \:....{C}_{{n}} ^{{n}} \right)^{\frac{\mathrm{1}}{{n}+\mathrm{1}}} \:\:\:{find}\:^{{n}} \sqrt{{A}}\:_{{n}} . \\ $$

Question Number 28531 Answers: 0 Comments: 0

let give S_n = Σ_(k=1) ^n k^p , p∈N and n≥1 find the radius of convergence of the serie Σ_(n≥1) (x^n /S_n ) .

$${let}\:{give}\:\:{S}_{{n}} =\:\sum_{{k}=\mathrm{1}} ^{{n}} \:{k}^{{p}} \:\:\:\:,\:{p}\in{N}\:\:{and}\:{n}\geqslant\mathrm{1} \\ $$$${find}\:{the}\:{radius}\:{of}\:{convergence}\:{of}\:{the}\:{serie}\:\:\sum_{{n}\geqslant\mathrm{1}} \:\frac{{x}^{{n}} }{{S}_{{n}} }\:. \\ $$

Question Number 28530 Answers: 0 Comments: 0

prove that ∣sinx∣= (8/π) Σ_(n=1) ^(+∞) ((sin^2 (nx))/(4n^2 −1)) .

$${prove}\:{that}\:\mid{sinx}\mid=\:\frac{\mathrm{8}}{\pi}\:\sum_{{n}=\mathrm{1}} ^{+\infty} \:\frac{{sin}^{\mathrm{2}} \left({nx}\right)}{\mathrm{4}{n}^{\mathrm{2}} −\mathrm{1}}\:. \\ $$

Question Number 28529 Answers: 0 Comments: 2

solve the d.e. (x^2 −1)y^′ +xy= x^2 −e^x .

$${solve}\:{the}\:{d}.{e}.\:\left({x}^{\mathrm{2}} −\mathrm{1}\right){y}^{'} +{xy}=\:{x}^{\mathrm{2}} −{e}^{{x}} \:. \\ $$

Pg 1795 Pg 1796 Pg 1797 Pg 1798 Pg 1799 Pg 1800 Pg 1801 Pg 1802 Pg 1803 Pg 1804

Contact: info@tinkutara.com