Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1799

Question Number 29007 Answers: 0 Comments: 1

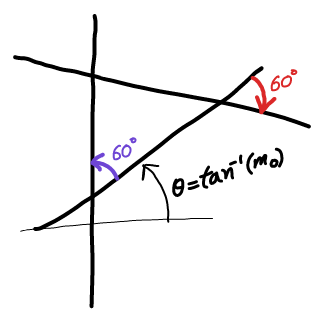
Question Number 29003 Answers: 1 Comments: 1

find ∫_0 ^∞ (dx/(1+x^3 )) .

$${find}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{3}} }\:. \\ $$

Question Number 29002 Answers: 0 Comments: 0

let give 0<p<1 calculate K(p)= ∫_(−∞) ^(+∞) (e^(pt) /(1+e^t ))dt.

$${let}\:{give}\:\mathrm{0}<{p}<\mathrm{1}\:{calculate}\:\:{K}\left({p}\right)=\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{e}^{{pt}} }{\mathrm{1}+{e}^{{t}} }{dt}. \\ $$

Question Number 29001 Answers: 0 Comments: 0

find the value of ∫_0 ^∞ ((cos(ξt))/(1+t^4 ))dt.

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{cos}\left(\xi{t}\right)}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}. \\ $$

Question Number 29000 Answers: 0 Comments: 1

prove thst ∫_R (e^(iξx) /(1+x^2 ))dx= π e^(−∣ξ∣) .

$${prove}\:{thst}\:\:\:\:\int_{\mathbb{R}} \:\:\:\:\frac{{e}^{{i}\xi{x}} }{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\:\pi\:{e}^{−\mid\xi\mid} \:\:. \\ $$

Question Number 28999 Answers: 0 Comments: 1

prove that ∫_0 ^∞ (e^(−t) /(√t))dt= e^(i(π/4)) ∫_0 ^∞ (e^(−ix) /(√x))dx.

$${prove}\:{that}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{t}} }{\sqrt{{t}}}{dt}=\:{e}^{{i}\frac{\pi}{\mathrm{4}}} \:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{ix}} }{\sqrt{{x}}}{dx}. \\ $$

Question Number 28998 Answers: 0 Comments: 0

find ∫_γ (e^z /(z(z+1)))dz with γ={z∈C/ ∣z−1∣=2}

$${find}\:\int_{\gamma} \:\:\:\:\frac{{e}^{{z}} }{{z}\left({z}+\mathrm{1}\right)}{dz}\:{with}\:\gamma=\left\{{z}\in{C}/\:\mid{z}−\mathrm{1}\mid=\mathrm{2}\right\} \\ $$

Question Number 28997 Answers: 0 Comments: 1

find ∫_(−∞) ^(+∞) (dx/((1+x^2 )( 2+e^(ix) ))) .

$${find}\:\int_{−\infty} ^{+\infty} \:\:\:\:\:\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left(\:\mathrm{2}+{e}^{{ix}} \right)}\:. \\ $$

Question Number 28996 Answers: 0 Comments: 0

find ∫_(−∞) ^(+∞) (x^2 /((x^2 +1)^2 (x^2 +2x+2)))dx.

$${find}\:\:\int_{−\infty} ^{+\infty} \:\:\:\:\:\:\:\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{2}{x}+\mathrm{2}\right)}{dx}. \\ $$

Question Number 28995 Answers: 0 Comments: 0

find ∫_0 ^(2π) ((cos(2t))/(3−cost)) dt.

$${find}\:\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\:\frac{{cos}\left(\mathrm{2}{t}\right)}{\mathrm{3}−{cost}}\:{dt}. \\ $$

Question Number 28994 Answers: 0 Comments: 0

find A_n = ∫_(−∞) ^(+∞) (dx/((1+x^2 )^n )) with n from N and n≥1.

$${find}\:\:{A}_{{n}} =\:\:\int_{−\infty} ^{+\infty} \:\:\:\:\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{{n}} }\:\:{with}\:{n}\:{from}\:{N}\:{and}\:{n}\geqslant\mathrm{1}. \\ $$

Question Number 28993 Answers: 1 Comments: 0

L means laplacr trsnsform find L (sin(at)) and L(cos(at)).

$${L}\:{means}\:{laplacr}\:{trsnsform}\:{find}\:{L}\:\left({sin}\left({at}\right)\right) \\ $$$${and}\:{L}\left({cos}\left({at}\right)\right). \\ $$

Question Number 28992 Answers: 0 Comments: 0

L means laplace transform find L(e^(at) )(s).

$${L}\:{means}\:{laplace}\:{transform}\:{find}\:\:{L}\left({e}^{{at}} \right)\left({s}\right). \\ $$

Question Number 28991 Answers: 1 Comments: 1

prove that L(1)(s)= (1/s) and L(t^n )(s)= ((n!)/s^(n+1) ) .L means laplace transform.

$${prove}\:{that}\:{L}\left(\mathrm{1}\right)\left({s}\right)=\:\frac{\mathrm{1}}{{s}}\:\:{and}\:{L}\left({t}^{{n}} \right)\left({s}\right)=\:\frac{{n}!}{{s}^{{n}+\mathrm{1}} }\:.{L}\:{means} \\ $$$${laplace}\:{transform}. \\ $$

Question Number 28990 Answers: 0 Comments: 0

calculate ∫_γ (e^z /((z−1)(z+3)^2 ))dz with γ id the positif circle γ={z∈C/ ∣z∣=(3/2)}.

$${calculate}\:\int_{\gamma} \:\:\:\frac{{e}^{{z}} }{\left({z}−\mathrm{1}\right)\left({z}+\mathrm{3}\right)^{\mathrm{2}} }{dz}\:{with}\:\gamma\:{id}\:{the}\:{positif} \\ $$$${circle}\:\gamma=\left\{{z}\in{C}/\:\mid{z}\mid=\frac{\mathrm{3}}{\mathrm{2}}\right\}. \\ $$

Question Number 28989 Answers: 0 Comments: 1

find ∫_0 ^∞ ((sin^2 (3x))/x^2 )dx.

$${find}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{sin}^{\mathrm{2}} \left(\mathrm{3}{x}\right)}{{x}^{\mathrm{2}} }{dx}. \\ $$

Question Number 28988 Answers: 0 Comments: 0

let give 0<α<1 find in terms of α the value of integral ∫_0 ^∞ (dx/(x^α (1+x))) .

$${let}\:{give}\:\mathrm{0}<\alpha<\mathrm{1}\:{find}\:{in}\:{terms}\:{of}\:\alpha\:{the}\:{value}\:{of}\:{integral} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{{x}^{\alpha} \left(\mathrm{1}+{x}\right)}\:. \\ $$

Question Number 28987 Answers: 0 Comments: 0

find ∫_0 ^(2π) (dt/((a+bcost)^2 )).with a>b>0 .

$${find}\:\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\:\:\:\frac{{dt}}{\left({a}+{bcost}\right)^{\mathrm{2}} }.{with}\:\:{a}>{b}>\mathrm{0}\:. \\ $$

Question Number 28986 Answers: 0 Comments: 0

let give a>1 find ∫_0 ^(2π) (dt/(a+cost)) .

$${let}\:{give}\:{a}>\mathrm{1}\:\:{find}\:\:\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\frac{{dt}}{{a}+{cost}}\:. \\ $$

Question Number 28985 Answers: 0 Comments: 0

let give I_(m,a) =∫_0 ^∞ ((cos(mx))/((1+x^2 )(x^2 +a^2 )))dx 1)verify that I_(m,1) =lim_(a→1) I_(m,a) 2) find the value of ∫_0 ^∞ ((x sin(mx))/((1+x^2 )^2 ))dx

$${let}\:{give}\:{I}_{{m},{a}} =\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{cos}\left({mx}\right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)}{dx} \\ $$$$\left.\mathrm{1}\right){verify}\:{that}\:{I}_{{m},\mathrm{1}} ={lim}_{{a}\rightarrow\mathrm{1}} \:{I}_{{m},{a}} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{x}\:{sin}\left({mx}\right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx} \\ $$

Question Number 28984 Answers: 0 Comments: 0

find F( (1/(1+x^4 ))) F means fourier transform.

$${find}\:{F}\left(\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{4}} }\right)\:{F}\:{means}\:{fourier}\:{transform}. \\ $$

Question Number 28983 Answers: 0 Comments: 0

find the value of∫_(−∞) ^(+∞) ((x^2 −1)/(x^2 +1)) ((sinx)/x)dx.

$${find}\:{the}\:{value}\:{of}\int_{−\infty} ^{+\infty} \:\frac{{x}^{\mathrm{2}} −\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\:\frac{{sinx}}{{x}}{dx}. \\ $$

Question Number 28982 Answers: 0 Comments: 0

fnd the value of Π_(n=1) ^∞ ((n^2 +1)/n^2 ) .

$${fnd}\:{the}\:{value}\:{of}\:\prod_{{n}=\mathrm{1}} ^{\infty} \:\:\:\frac{{n}^{\mathrm{2}} +\mathrm{1}}{{n}^{\mathrm{2}} }\:\:. \\ $$

Question Number 28981 Answers: 1 Comments: 1

find the values of Π_(n=2) ^∞ (1−(2/(n(n+1)))) .

$${find}\:{the}\:{values}\:{of}\:\prod_{{n}=\mathrm{2}} ^{\infty} \left(\mathrm{1}−\frac{\mathrm{2}}{{n}\left({n}+\mathrm{1}\right)}\right)\:. \\ $$

Question Number 28980 Answers: 0 Comments: 0

prove that sin(πz)=πz Π_(k=1) ^∞ (1−(z^2 /k^2 )) zfromC.

$${prove}\:{that}\:{sin}\left(\pi{z}\right)=\pi{z}\:\prod_{{k}=\mathrm{1}} ^{\infty} \left(\mathrm{1}−\frac{{z}^{\mathrm{2}} }{{k}^{\mathrm{2}} }\right)\:\:{zfromC}. \\ $$

Question Number 29012 Answers: 0 Comments: 1

Pg 1794 Pg 1795 Pg 1796 Pg 1797 Pg 1798 Pg 1799 Pg 1800 Pg 1801 Pg 1802 Pg 1803

Terms of Service

Contact: info@tinkutara.com