Question and Answers Forum

AllQuestion and Answers: Page 1798

Question Number 29134 Answers: 0 Comments: 3

Question Number 29131 Answers: 1 Comments: 2

If tan^2 atan^2 b+tan^2 btan^2 c+tan^2 ctan^2 a +2tan^2 atan^2 btan^2 c=1 then find the value of: sin^2 a+sin^2 b+sin^2 c

$${If}\:\mathrm{tan}^{\mathrm{2}} {a}\mathrm{tan}\:^{\mathrm{2}} {b}+\mathrm{tan}^{\mathrm{2}} {b}\mathrm{tan}\:^{\mathrm{2}} {c}+\mathrm{tan}^{\mathrm{2}} {c}\mathrm{tan}\:^{\mathrm{2}} {a} \\ $$$$+\mathrm{2tan}^{\mathrm{2}} {a}\mathrm{tan}^{\mathrm{2}} {b}\mathrm{tan}\:^{\mathrm{2}} {c}=\mathrm{1} \\ $$$${then}\:{find}\:{the}\:{value}\:{of}: \\ $$$$\mathrm{sin}\:^{\mathrm{2}} {a}+\mathrm{sin}\:^{\mathrm{2}} {b}+\mathrm{sin}\:^{\mathrm{2}} {c} \\ $$

Question Number 29136 Answers: 0 Comments: 1

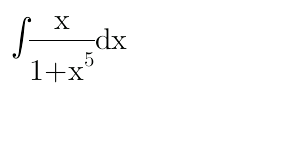
Question Number 29144 Answers: 1 Comments: 0

A body moves in a circular orbit of radius 4R round the earth. Express the acceleration of the free fall due to gravity of the body in terms of g R = radius if the earth g = acceleration due to gravity

$$\mathrm{A}\:\mathrm{body}\:\mathrm{moves}\:\mathrm{in}\:\mathrm{a}\:\mathrm{circular}\:\mathrm{orbit}\:\mathrm{of}\:\mathrm{radius}\:\mathrm{4R}\:\mathrm{round}\:\mathrm{the}\:\mathrm{earth}.\:\:\mathrm{Express}\:\mathrm{the}\:\mathrm{acceleration} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{free}\:\mathrm{fall}\:\mathrm{due}\:\mathrm{to}\:\mathrm{gravity}\:\mathrm{of}\:\mathrm{the}\:\mathrm{body}\:\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:\mathrm{g} \\ $$$$\mathrm{R}\:=\:\mathrm{radius}\:\mathrm{if}\:\mathrm{the}\:\mathrm{earth} \\ $$$$\mathrm{g}\:=\:\mathrm{acceleration}\:\mathrm{due}\:\mathrm{to}\:\mathrm{gravity} \\ $$

Question Number 29159 Answers: 0 Comments: 1

let give f(x)=2(√(x−1)) +3x find f^(−1) (x) and (f^(−1) )^′ (x) .

$${let}\:{give}\:{f}\left({x}\right)=\mathrm{2}\sqrt{{x}−\mathrm{1}}\:+\mathrm{3}{x}\:\:\:{find}\:{f}^{−\mathrm{1}} \left({x}\right)\:{and}\:\left({f}^{−\mathrm{1}} \right)^{'} \left({x}\right)\:. \\ $$

Question Number 29158 Answers: 0 Comments: 1

find lim_(x→1) (((√(3+(√(2x−1)))) −2)/((√(2+(√(3x+1)))) −(√(x+3)))) .

$${find}\:{lim}_{{x}\rightarrow\mathrm{1}} \frac{\sqrt{\mathrm{3}+\sqrt{\mathrm{2}{x}−\mathrm{1}}}\:−\mathrm{2}}{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}{x}+\mathrm{1}}}\:\:−\sqrt{{x}+\mathrm{3}}}\:\:. \\ $$

Question Number 29157 Answers: 1 Comments: 1

find lim_(x→1) (((x−2)^(1/3) +(1−x+x^2 )^(1/3) )/(x^2 −1)) .

$${find}\:{lim}_{{x}\rightarrow\mathrm{1}} \:\:\:\frac{\left({x}−\mathrm{2}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\:+\left(\mathrm{1}−{x}+{x}^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} }{{x}^{\mathrm{2}} −\mathrm{1}}\:. \\ $$

Question Number 29156 Answers: 0 Comments: 2

find lim_(x→0^+ ) x[(1/x)] and lim_(x→0^+ ) x^2 [ (1/x)] . [α] is the greatest integr inferior or equal to α.

$${find}\:{lim}_{{x}\rightarrow\mathrm{0}^{+} } \:\:\:{x}\left[\frac{\mathrm{1}}{{x}}\right]\:\:{and}\:\:{lim}_{{x}\rightarrow\mathrm{0}^{+} } \:\:\:\:{x}^{\mathrm{2}} \:\left[\:\frac{\mathrm{1}}{{x}}\right]\:\:. \\ $$$$\left[\alpha\right]\:{is}\:{the}\:{greatest}\:{integr}\:{inferior}\:{or}\:{equal}\:{to}\:\alpha. \\ $$

Question Number 29123 Answers: 0 Comments: 0

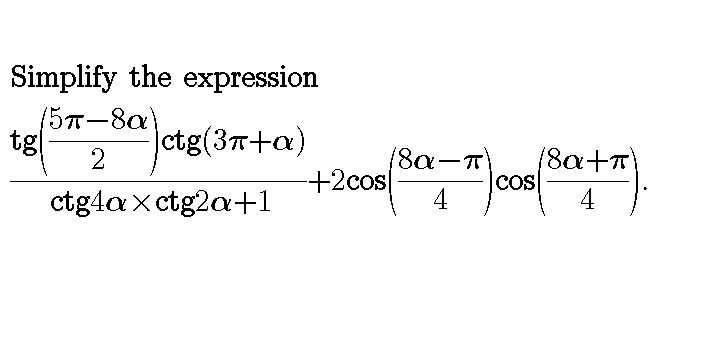
Question Number 29118 Answers: 1 Comments: 6

Question Number 29116 Answers: 1 Comments: 0

Find the area of the region R bounded by the curve y = cosh(x), the line x = log_e (2) and the coordinate axis . Find also the volume obtained when R is rotated completely about the x − axis.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{region}\:\boldsymbol{\mathrm{R}}\:\mathrm{bounded}\:\mathrm{by}\:\mathrm{the}\:\mathrm{curve}\:\:\mathrm{y}\:=\:\mathrm{cosh}\left(\mathrm{x}\right),\:\:\mathrm{the}\:\mathrm{line}\:\:\mathrm{x}\:=\:\mathrm{log}_{\mathrm{e}} \left(\mathrm{2}\right) \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{coordinate}\:\mathrm{axis}\:.\:\:\mathrm{Find}\:\mathrm{also}\:\mathrm{the}\:\mathrm{volume}\:\mathrm{obtained}\:\mathrm{when}\:\boldsymbol{\mathrm{R}}\:\mathrm{is}\:\mathrm{rotated}\: \\ $$$$\mathrm{completely}\:\mathrm{about}\:\mathrm{the}\:\:\mathrm{x}\:−\:\mathrm{axis}. \\ $$

Question Number 29111 Answers: 1 Comments: 1

cos^3 x.sin^2 x=1/16(2cos x−cos 3x−cos 5x)

$$\mathrm{cos}^{\mathrm{3}} {x}.\mathrm{sin}\:^{\mathrm{2}} {x}=\mathrm{1}/\mathrm{16}\left(\mathrm{2cos}\:{x}−\mathrm{cos}\:\mathrm{3}{x}−\mathrm{cos}\:\mathrm{5}{x}\right) \\ $$

Question Number 29107 Answers: 1 Comments: 1

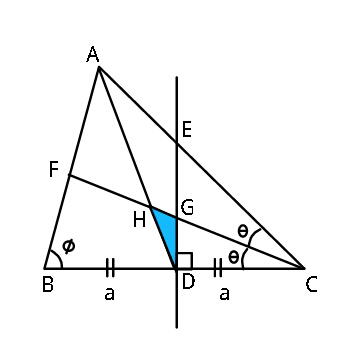
Question Number 29105 Answers: 0 Comments: 2

Show that: ∫_(−1) ^( 1) (dx/(5 cosh(x) + 13 sinh(x))) = (1/2) log_e (((15e − 10)/(3e + 2)))

$$\mathrm{Show}\:\mathrm{that}:\:\:\:\int_{−\mathrm{1}} ^{\:\:\:\mathrm{1}} \:\:\:\:\:\:\:\frac{\mathrm{dx}}{\mathrm{5}\:\mathrm{cosh}\left(\mathrm{x}\right)\:+\:\mathrm{13}\:\mathrm{sinh}\left(\mathrm{x}\right)}\:\:=\:\:\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{log}_{\mathrm{e}} \left(\frac{\mathrm{15e}\:−\:\mathrm{10}}{\mathrm{3e}\:+\:\mathrm{2}}\right)\: \\ $$

Question Number 29080 Answers: 2 Comments: 0

let give a>0 study the convergence of Σ_(n=1) ^∞ a^H_n with H_n = Σ_(k=1) ^n (1/k).

$${let}\:{give}\:{a}>\mathrm{0}\:{study}\:{the}\:{convergence}\:{of}\:\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:{a}^{{H}_{{n}} } \:\: \\ $$$${with}\:{H}_{{n}} =\:\sum_{{k}=\mathrm{1}} ^{{n}} \:\:\frac{\mathrm{1}}{{k}}. \\ $$

Question Number 29079 Answers: 0 Comments: 0

let give w(x)= ∫_0 ^1 ((arcsin(x(1+t^2 )))/(1+t^2 ))dt find w(x).

$${let}\:{give}\:{w}\left({x}\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{arcsin}\left({x}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:{find}\:{w}\left({x}\right). \\ $$

Question Number 29078 Answers: 0 Comments: 2

let give h(x)= ∫_0 ^1 ((arctan(xt))/(1+t^2 )) find h(x) .

$${let}\:{give}\:\:{h}\left({x}\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{arctan}\left({xt}\right)}{\mathrm{1}+{t}^{\mathrm{2}} }\:\:{find}\:{h}\left({x}\right)\:. \\ $$

Question Number 29077 Answers: 0 Comments: 1

let give g(x)=∫_0 ^∞ ((arctan(x(1+t^2 )))/(1+t^2 ))dt find a simple form of g^′ (x) without integral.

$${let}\:{give}\:{g}\left({x}\right)=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left({x}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:{find}\:{a}\:{simple} \\ $$$${form}\:{of}\:\:{g}^{'} \left({x}\right)\:{without}\:{integral}. \\ $$

Question Number 29076 Answers: 0 Comments: 1

let give f(x)= ∫_0 ^1 ((arctan(x(1+t^2 )))/(1+t^2 ))dt find asimple form of f(x) without integral.

$${let}\:{give}\:{f}\left({x}\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctan}\left({x}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:{find}\:{asimple} \\ $$$${form}\:{of}\:{f}\left({x}\right)\:{without}\:{integral}. \\ $$

Question Number 29063 Answers: 0 Comments: 0

Question Number 29049 Answers: 1 Comments: 0

Prove that e^(iπ) +1=0

$${Prove}\:{that} \\ $$$$\:\:\:\:\:{e}^{{i}\pi} +\mathrm{1}=\mathrm{0} \\ $$

Question Number 29048 Answers: 1 Comments: 19

Question Number 29043 Answers: 0 Comments: 1

∫tan^− (1−sinx/1+sinx) dx

$$\int\mathrm{tan}^{−} \left(\mathrm{1}−\mathrm{sinx}/\mathrm{1}+\mathrm{sinx}\right)\:\mathrm{dx} \\ $$

Question Number 29038 Answers: 0 Comments: 1

find ∫_(−∞) ^(+∞) ((cos(at))/(1+t^4 ))dt.

$${find}\:\:\int_{−\infty} ^{+\infty} \:\:\:\:\frac{{cos}\left({at}\right)}{\mathrm{1}+{t}^{\mathrm{4}} }{dt}. \\ $$

Question Number 29037 Answers: 0 Comments: 0

let give the sequence (y_n ) /y_0 (x)=1 and y_n (x)= 1+ ∫_0 ^x (y_(n−1) (t))^2 dt , let suppose x∈[0,1] prove that (y_n ) is increasing majored by (1/(1−x)) if y=lim_(n→+∞) y_n prove that y is solution of differencial equation y^, =y^2 and y(o)=1.

$${let}\:{give}\:{the}\:{sequence}\:\:\left({y}_{{n}} \right)\:/{y}_{\mathrm{0}} \left({x}\right)=\mathrm{1}\:\:{and} \\ $$$${y}_{{n}} \left({x}\right)=\:\mathrm{1}+\:\int_{\mathrm{0}} ^{{x}} \left({y}_{{n}−\mathrm{1}} \left({t}\right)\right)^{\mathrm{2}} {dt}\:,\:{let}\:{suppose}\:{x}\in\left[\mathrm{0},\mathrm{1}\right]\:{prove} \\ $$$${that}\:\left({y}_{{n}} \right)\:{is}\:{increasing}\:{majored}\:{by}\:\frac{\mathrm{1}}{\mathrm{1}−{x}}\:{if}\:{y}={lim}_{{n}\rightarrow+\infty} {y}_{{n}} \\ $$$${prove}\:{that}\:{y}\:{is}\:{solution}\:{of}\:{differencial}\:{equation} \\ $$$${y}^{,} ={y}^{\mathrm{2}} \:{and}\:{y}\left({o}\right)=\mathrm{1}. \\ $$

Question Number 29036 Answers: 0 Comments: 0

p=2m+1 is a prime number prove that 1) (p−1)!≡ −1[p] 2) (m!)^2 ≡ (−1)^(m+1) [p]

$${p}=\mathrm{2}{m}+\mathrm{1}\:{is}\:{a}\:{prime}\:{number}\:{prove}\:{that} \\ $$$$\left.\mathrm{1}\right)\:\left({p}−\mathrm{1}\right)!\equiv\:−\mathrm{1}\left[{p}\right] \\ $$$$\left.\mathrm{2}\right)\:\left({m}!\right)^{\mathrm{2}} \equiv\:\left(−\mathrm{1}\right)^{{m}+\mathrm{1}} \:\left[{p}\right] \\ $$

Pg 1793 Pg 1794 Pg 1795 Pg 1796 Pg 1797 Pg 1798 Pg 1799 Pg 1800 Pg 1801 Pg 1802

Contact: info@tinkutara.com