
AllQuestion and Answers: Page 1789
Question Number 29505 Answers: 0 Comments: 3
Question Number 29503 Answers: 0 Comments: 0
Question Number 29501 Answers: 0 Comments: 0
Question Number 29500 Answers: 0 Comments: 1
Question Number 29498 Answers: 1 Comments: 1

Question Number 29499 Answers: 0 Comments: 0
Question Number 29491 Answers: 1 Comments: 0
Question Number 29490 Answers: 1 Comments: 0
Question Number 29488 Answers: 1 Comments: 4
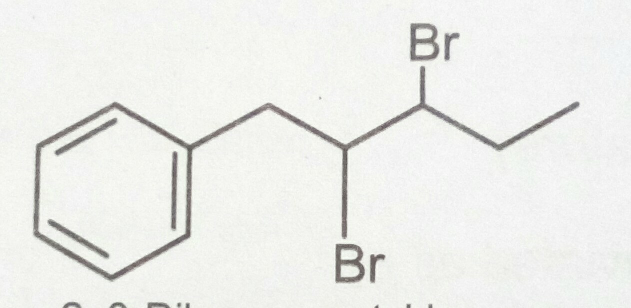
Question Number 29478 Answers: 1 Comments: 0
Question Number 29464 Answers: 1 Comments: 0
Question Number 29463 Answers: 1 Comments: 0
Question Number 29461 Answers: 0 Comments: 1
Question Number 29460 Answers: 2 Comments: 0
Question Number 29459 Answers: 0 Comments: 6
Question Number 29458 Answers: 0 Comments: 1
Question Number 29457 Answers: 0 Comments: 0
Question Number 29456 Answers: 0 Comments: 1
Question Number 29455 Answers: 1 Comments: 1
$${find}\:\int\:\:\mathrm{3}^{\sqrt{\mathrm{2}{x}+\mathrm{1}}} \:{dx}\:. \\ $$
Question Number 29454 Answers: 0 Comments: 1
Question Number 29453 Answers: 0 Comments: 0
Question Number 29452 Answers: 0 Comments: 1
Question Number 29451 Answers: 0 Comments: 1
Question Number 29450 Answers: 0 Comments: 1
Question Number 29449 Answers: 0 Comments: 0
Question Number 29448 Answers: 0 Comments: 0
Pg 1784 Pg 1785 Pg 1786 Pg 1787 Pg 1788 Pg 1789 Pg 1790 Pg 1791 Pg 1792 Pg 1793
