
AllQuestion and Answers: Page 1765
Question Number 31772 Answers: 0 Comments: 0
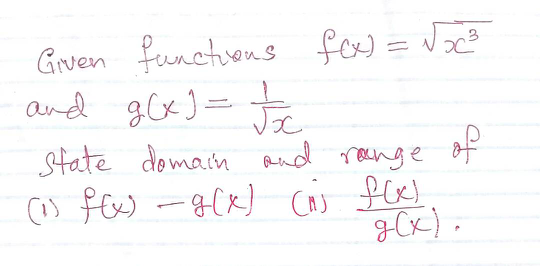
Question Number 31771 Answers: 1 Comments: 1
Question Number 31768 Answers: 0 Comments: 0
Question Number 31767 Answers: 0 Comments: 0
Question Number 31766 Answers: 0 Comments: 1
Question Number 31763 Answers: 3 Comments: 0
Question Number 31749 Answers: 0 Comments: 2
Question Number 31748 Answers: 0 Comments: 3
Question Number 31747 Answers: 0 Comments: 1
Question Number 31743 Answers: 1 Comments: 0
Question Number 31740 Answers: 1 Comments: 0
Question Number 31734 Answers: 1 Comments: 0
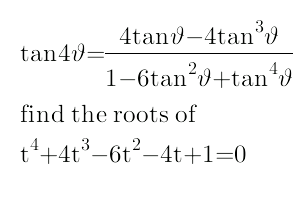
Question Number 31745 Answers: 1 Comments: 0
Question Number 31726 Answers: 1 Comments: 3
Question Number 31719 Answers: 0 Comments: 3

Question Number 31717 Answers: 1 Comments: 0

Question Number 31715 Answers: 0 Comments: 0

Question Number 31714 Answers: 0 Comments: 0
Question Number 31713 Answers: 0 Comments: 3
Question Number 31712 Answers: 0 Comments: 0
Question Number 31711 Answers: 0 Comments: 0
Question Number 31709 Answers: 0 Comments: 0
Question Number 31708 Answers: 0 Comments: 0
Question Number 31707 Answers: 0 Comments: 1
Question Number 31706 Answers: 0 Comments: 1
Question Number 31705 Answers: 0 Comments: 0
Pg 1760 Pg 1761 Pg 1762 Pg 1763 Pg 1764 Pg 1765 Pg 1766 Pg 1767 Pg 1768 Pg 1769
