
AllQuestion and Answers: Page 1763
Question Number 31671 Answers: 0 Comments: 0
Question Number 31670 Answers: 1 Comments: 0
Question Number 31669 Answers: 0 Comments: 0
Question Number 31666 Answers: 1 Comments: 0
Question Number 32371 Answers: 2 Comments: 1
Question Number 31648 Answers: 0 Comments: 0

Question Number 31642 Answers: 0 Comments: 1
Question Number 31639 Answers: 1 Comments: 0

Question Number 31628 Answers: 0 Comments: 5
Question Number 31626 Answers: 1 Comments: 0
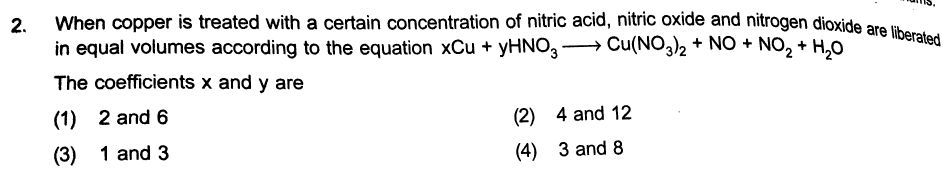
Question Number 31620 Answers: 0 Comments: 0

Question Number 31619 Answers: 1 Comments: 0

Question Number 31611 Answers: 2 Comments: 1
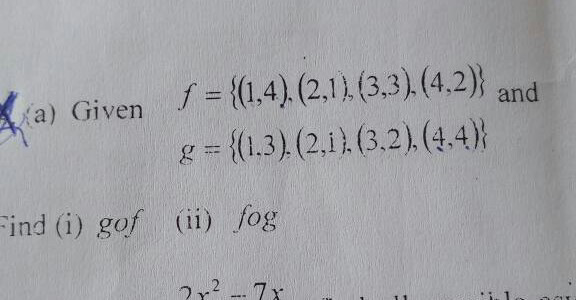
Question Number 31596 Answers: 2 Comments: 2

Question Number 31595 Answers: 2 Comments: 2

Question Number 31594 Answers: 1 Comments: 0
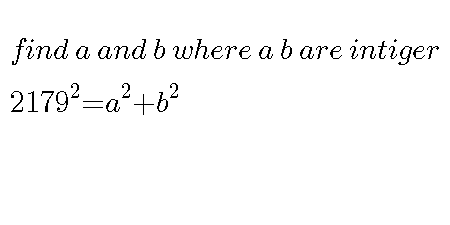
Question Number 31591 Answers: 1 Comments: 4
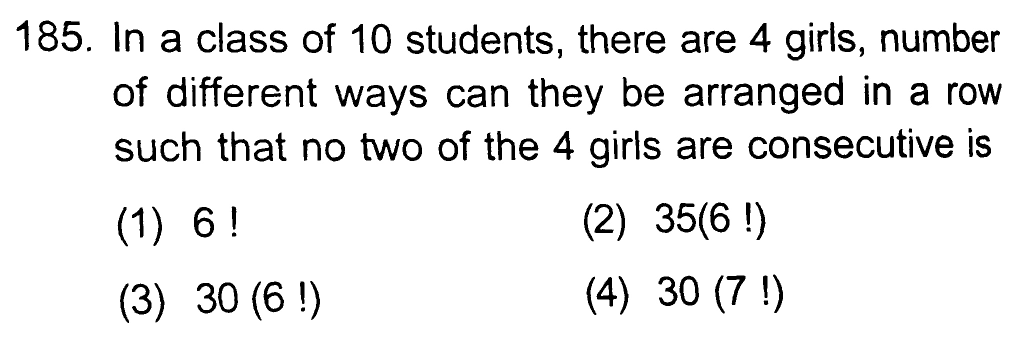
Question Number 31584 Answers: 1 Comments: 0

Question Number 31583 Answers: 0 Comments: 0
$${how}\:{to}\:{read}\:{this}\:\langle{x}\rangle.\:{p} \\ $$
Question Number 31579 Answers: 1 Comments: 0
Question Number 31573 Answers: 0 Comments: 1
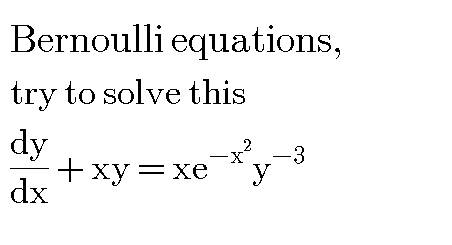
Question Number 31572 Answers: 1 Comments: 0

Question Number 31571 Answers: 2 Comments: 0
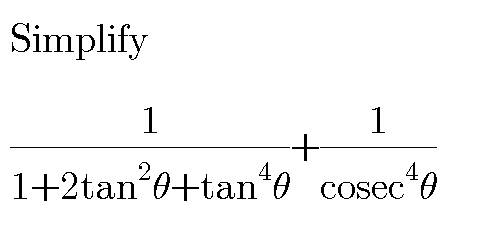
Question Number 31569 Answers: 1 Comments: 0
Question Number 31566 Answers: 1 Comments: 1

Question Number 31564 Answers: 0 Comments: 1
Pg 1758 Pg 1759 Pg 1760 Pg 1761 Pg 1762 Pg 1763 Pg 1764 Pg 1765 Pg 1766 Pg 1767
