
AllQuestion and Answers: Page 1757
Question Number 32237 Answers: 0 Comments: 0

Question Number 32236 Answers: 1 Comments: 0

Question Number 32233 Answers: 0 Comments: 1
Question Number 32220 Answers: 0 Comments: 3
Question Number 32218 Answers: 1 Comments: 0

Question Number 32209 Answers: 1 Comments: 0
Question Number 32208 Answers: 0 Comments: 0

Question Number 32207 Answers: 0 Comments: 0
Question Number 32206 Answers: 0 Comments: 0
Question Number 32203 Answers: 0 Comments: 5
Question Number 32191 Answers: 1 Comments: 1
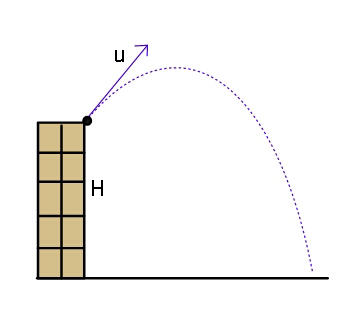
Question Number 32211 Answers: 1 Comments: 1
Question Number 32184 Answers: 1 Comments: 0
Question Number 32181 Answers: 1 Comments: 1
Question Number 32163 Answers: 0 Comments: 0
Question Number 32161 Answers: 1 Comments: 0
Question Number 32160 Answers: 1 Comments: 0
Question Number 32159 Answers: 1 Comments: 2
Question Number 32158 Answers: 0 Comments: 0
Question Number 32164 Answers: 0 Comments: 0
Question Number 32156 Answers: 0 Comments: 2
$${evaluate}\int\frac{\sqrt{{cos}\:\mathrm{2}{x}}}{{sin}\:{x}}{dx} \\ $$
Question Number 32142 Answers: 0 Comments: 0

Question Number 32150 Answers: 0 Comments: 0

Question Number 32139 Answers: 0 Comments: 4
Question Number 32137 Answers: 0 Comments: 0
Question Number 32132 Answers: 0 Comments: 0
$$\int\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right){ln}\left({x}\right)}{dx}=? \\ $$
Pg 1752 Pg 1753 Pg 1754 Pg 1755 Pg 1756 Pg 1757 Pg 1758 Pg 1759 Pg 1760 Pg 1761
