Question and Answers Forum

AllQuestion and Answers: Page 1756

Question Number 32954 Answers: 1 Comments: 0

log_2 x×log_4 x×log_(16) x=7 help

$$\:\boldsymbol{\mathrm{log}}_{\mathrm{2}} \boldsymbol{{x}}×\boldsymbol{\mathrm{log}}_{\mathrm{4}} \boldsymbol{{x}}×\boldsymbol{\mathrm{log}}_{\mathrm{16}} \boldsymbol{{x}}=\mathrm{7} \\ $$$$\:\boldsymbol{{help}} \\ $$

Question Number 32951 Answers: 2 Comments: 1

Evaluate ∫((x^4 +1)/(x^6 +1))dx [W.B.H.S 2018]

$${Evaluate} \\ $$$$\int\frac{{x}^{\mathrm{4}} +\mathrm{1}}{{x}^{\mathrm{6}} +\mathrm{1}}{dx}\:\:\:\:\:\left[{W}.{B}.{H}.{S}\:\mathrm{2018}\right] \\ $$

Question Number 32947 Answers: 1 Comments: 0

Question Number 32946 Answers: 1 Comments: 0

Question Number 32945 Answers: 1 Comments: 0

Question Number 32939 Answers: 1 Comments: 1

1) study the convergence of ∫_0 ^1 (x^p /(1+x)) dx 2) find lim_(p→∞) ∫_0 ^1 (x^p /(1+x))dx .

$$\left.\mathrm{1}\right)\:{study}\:{the}\:{convergence}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{x}^{{p}} }{\mathrm{1}+{x}}\:{dx} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{lim}_{{p}\rightarrow\infty} \:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{x}^{{p}} }{\mathrm{1}+{x}}{dx}\:. \\ $$

Question Number 32938 Answers: 0 Comments: 2

let 0<θ<π find Σ_(n=1) ^∞ ((cos(nθ))/n) 2) find Σ_(n=1) ^∞ ((sin(nθ))/n)

$${let}\:\mathrm{0}<\theta<\pi\:\:{find}\:\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{{cos}\left({n}\theta\right)}{{n}} \\ $$$$\left.\mathrm{2}\right)\:{find}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{{sin}\left({n}\theta\right)}{{n}} \\ $$

Question Number 32937 Answers: 0 Comments: 0

let give ∣x∣<1 prove that Σ_(n=1) ^∞ ((sin(nθ))/n) x^n =arctan( ((xsinθ)/(1−xcosθ))) .

$${let}\:{give}\:\mid{x}\mid<\mathrm{1}\:\:{prove}\:{that} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{{sin}\left({n}\theta\right)}{{n}}\:{x}^{{n}} \:={arctan}\left(\:\frac{{xsin}\theta}{\mathrm{1}−{xcos}\theta}\right)\:. \\ $$

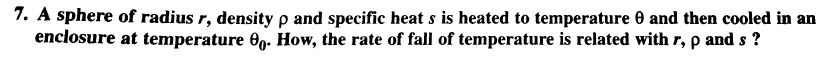
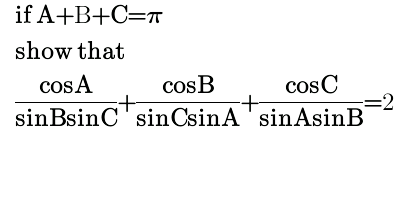
Question Number 32936 Answers: 0 Comments: 0

let give ∣x∣<1 prove that Σ_(n=1) ^∞ ((cos(nθ))/n) x^n =−(1/2)ln(1−2xcosθ+x^2 ) .

$${let}\:{give}\:\mid{x}\mid<\mathrm{1}\:{prove}\:{that} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{{cos}\left({n}\theta\right)}{{n}}\:{x}^{{n}} \:=−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{1}−\mathrm{2}{xcos}\theta+{x}^{\mathrm{2}} \right)\:. \\ $$

Question Number 32935 Answers: 0 Comments: 0

let give ∣x∣<1 prove that Σ_(n=1) ^∞ x^n sin(nθ) = ((x sinθ)/(1−2x cosθ +x^2 )) .

$${let}\:{give}\:\mid{x}\mid<\mathrm{1}\:\:{prove}\:{that} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:{x}^{{n}} {sin}\left({n}\theta\right)\:=\:\:\frac{{x}\:{sin}\theta}{\mathrm{1}−\mathrm{2}{x}\:{cos}\theta\:+{x}^{\mathrm{2}} }\:. \\ $$

Question Number 32934 Answers: 0 Comments: 0

let give ∣x∣<1 prove that Σ_(n=1) ^∞ x^n cos(nθ)= ((xcosθ −x^2 )/(1−2xcosθ +x^2 ))

$${let}\:{give}\:\mid{x}\mid<\mathrm{1}\:{prove}\:{that} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:{x}^{{n}} {cos}\left({n}\theta\right)=\:\frac{{xcos}\theta\:−{x}^{\mathrm{2}} }{\mathrm{1}−\mathrm{2}{xcos}\theta\:+{x}^{\mathrm{2}} } \\ $$

Question Number 32933 Answers: 0 Comments: 0

Σ u_n is a convergent serie with positif terms find the nature of Σ_(n≥1) ((√u_n )/n) and Σ_(n≥o) (u_n /(1+u_n )) .

$$\Sigma\:{u}_{{n}} \:{is}\:{a}\:{convergent}\:{serie}\:{with}\:{positif}\:{terms} \\ $$$${find}\:{the}\:{nature}\:{of}\:\:\sum_{{n}\geqslant\mathrm{1}} \:\frac{\sqrt{{u}_{{n}} }}{{n}}\:\:{and}\:\:\:\sum_{{n}\geqslant{o}} \:\:\frac{{u}_{{n}} }{\mathrm{1}+{u}_{{n}} }\:\:. \\ $$

Question Number 32932 Answers: 0 Comments: 1

find the nature of Σ_(n=1) ^∞ (1/(nΣ_(k=1) ^n (1/k))) .

$${find}\:{the}\:{nature}\:{of}\:\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\mathrm{1}}{{n}\sum_{{k}=\mathrm{1}} ^{{n}} \:\frac{\mathrm{1}}{{k}}}\:. \\ $$

Question Number 32928 Answers: 2 Comments: 2

plz help Evalute ∫_(π/3 ) ^(π/4) ((sin^2 x)/(√(1−cosx)))dx

$$\boldsymbol{{plz}}\:\boldsymbol{{help}} \\ $$$${Evalute} \\ $$$$ \\ $$$$\underset{\pi/\mathrm{3}\:} {\overset{\pi/\mathrm{4}} {\int}}\:\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\sqrt{\mathrm{1}−{cosx}}}{dx} \\ $$

Question Number 32918 Answers: 0 Comments: 1

Question Number 32914 Answers: 0 Comments: 4

Question Number 32913 Answers: 0 Comments: 0

2∧6

$$\mathrm{2}\wedge\mathrm{6} \\ $$

Question Number 32912 Answers: 1 Comments: 0

find x log(√x)=(√(logx))

$$\:\boldsymbol{\mathrm{find}}\:\boldsymbol{\mathrm{x}} \\ $$$$\:\boldsymbol{\mathrm{log}}\sqrt{\boldsymbol{{x}}}=\sqrt{\boldsymbol{\mathrm{log}{x}}} \\ $$

Question Number 32908 Answers: 2 Comments: 2

2018^(2017) > 2017^(2018) or 2018^(2017) < 2017^(2018) ?

$$\mathrm{2018}^{\mathrm{2017}} \:>\:\mathrm{2017}^{\mathrm{2018}} \:{or} \\ $$$$\mathrm{2018}^{\mathrm{2017}} \:<\:\mathrm{2017}^{\mathrm{2018}} \:\:? \\ $$

Question Number 32900 Answers: 0 Comments: 0

Question Number 32897 Answers: 3 Comments: 0

e^π > π^e or e^π <π^e ?

$$ \\ $$$${e}^{\pi} \:>\:\pi^{{e}} \:\:{or}\:\:\:{e}^{\pi} <\pi^{{e}} \:? \\ $$$$ \\ $$

Question Number 32891 Answers: 0 Comments: 3

Question Number 32889 Answers: 0 Comments: 1

Question Number 32885 Answers: 1 Comments: 0

Question Number 32878 Answers: 1 Comments: 0

A= [(α,1,1,1),(1,α,1,1),(1,1,β,1),(1,1,1,β) ]with α^2 ≠1≠β^2 det(A)=....???

$${A}=\begin{bmatrix}{\alpha}&{\mathrm{1}}&{\mathrm{1}}&{\mathrm{1}}\\{\mathrm{1}}&{\alpha}&{\mathrm{1}}&{\mathrm{1}}\\{\mathrm{1}}&{\mathrm{1}}&{\beta}&{\mathrm{1}}\\{\mathrm{1}}&{\mathrm{1}}&{\mathrm{1}}&{\beta}\end{bmatrix}{with}\:\alpha^{\mathrm{2}} \neq\mathrm{1}\neq\beta^{\mathrm{2}} \\ $$$${det}\left({A}\right)=....??? \\ $$

Question Number 32877 Answers: 1 Comments: 0

[(2,1),(0,2) ]^(2018) =.....???

$$\begin{bmatrix}{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{0}}&{\mathrm{2}}\end{bmatrix}^{\mathrm{2018}} =.....??? \\ $$

Pg 1751 Pg 1752 Pg 1753 Pg 1754 Pg 1755 Pg 1756 Pg 1757 Pg 1758 Pg 1759 Pg 1760

Contact: info@tinkutara.com