Question and Answers Forum

AllQuestion and Answers: Page 1753

Question Number 32935 Answers: 0 Comments: 0

let give ∣x∣<1 prove that Σ_(n=1) ^∞ x^n sin(nθ) = ((x sinθ)/(1−2x cosθ +x^2 )) .

$${let}\:{give}\:\mid{x}\mid<\mathrm{1}\:\:{prove}\:{that} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:{x}^{{n}} {sin}\left({n}\theta\right)\:=\:\:\frac{{x}\:{sin}\theta}{\mathrm{1}−\mathrm{2}{x}\:{cos}\theta\:+{x}^{\mathrm{2}} }\:. \\ $$

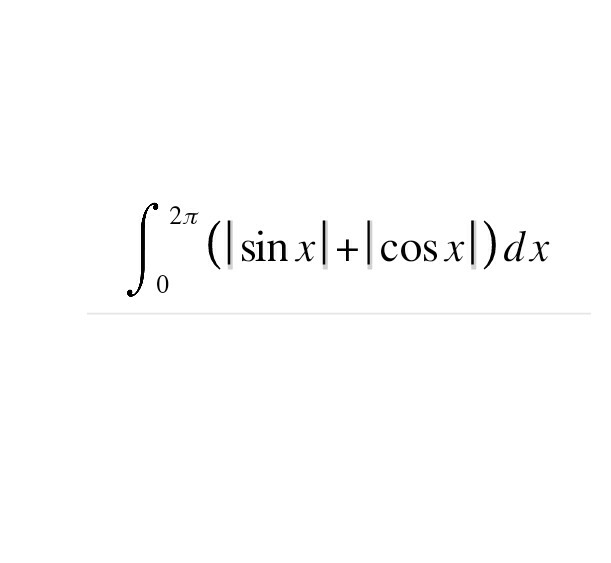
Question Number 32934 Answers: 0 Comments: 0

let give ∣x∣<1 prove that Σ_(n=1) ^∞ x^n cos(nθ)= ((xcosθ −x^2 )/(1−2xcosθ +x^2 ))

$${let}\:{give}\:\mid{x}\mid<\mathrm{1}\:{prove}\:{that} \\ $$$$\sum_{{n}=\mathrm{1}} ^{\infty} \:{x}^{{n}} {cos}\left({n}\theta\right)=\:\frac{{xcos}\theta\:−{x}^{\mathrm{2}} }{\mathrm{1}−\mathrm{2}{xcos}\theta\:+{x}^{\mathrm{2}} } \\ $$

Question Number 32933 Answers: 0 Comments: 0

Σ u_n is a convergent serie with positif terms find the nature of Σ_(n≥1) ((√u_n )/n) and Σ_(n≥o) (u_n /(1+u_n )) .

$$\Sigma\:{u}_{{n}} \:{is}\:{a}\:{convergent}\:{serie}\:{with}\:{positif}\:{terms} \\ $$$${find}\:{the}\:{nature}\:{of}\:\:\sum_{{n}\geqslant\mathrm{1}} \:\frac{\sqrt{{u}_{{n}} }}{{n}}\:\:{and}\:\:\:\sum_{{n}\geqslant{o}} \:\:\frac{{u}_{{n}} }{\mathrm{1}+{u}_{{n}} }\:\:. \\ $$

Question Number 32932 Answers: 0 Comments: 1

find the nature of Σ_(n=1) ^∞ (1/(nΣ_(k=1) ^n (1/k))) .

$${find}\:{the}\:{nature}\:{of}\:\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\mathrm{1}}{{n}\sum_{{k}=\mathrm{1}} ^{{n}} \:\frac{\mathrm{1}}{{k}}}\:. \\ $$

Question Number 32928 Answers: 2 Comments: 2

plz help Evalute ∫_(π/3 ) ^(π/4) ((sin^2 x)/(√(1−cosx)))dx

$$\boldsymbol{{plz}}\:\boldsymbol{{help}} \\ $$$${Evalute} \\ $$$$ \\ $$$$\underset{\pi/\mathrm{3}\:} {\overset{\pi/\mathrm{4}} {\int}}\:\frac{\mathrm{sin}^{\mathrm{2}} {x}}{\sqrt{\mathrm{1}−{cosx}}}{dx} \\ $$

Question Number 32918 Answers: 0 Comments: 1

Question Number 32914 Answers: 0 Comments: 4

Question Number 32913 Answers: 0 Comments: 0

2∧6

$$\mathrm{2}\wedge\mathrm{6} \\ $$

Question Number 32912 Answers: 1 Comments: 0

find x log(√x)=(√(logx))

$$\:\boldsymbol{\mathrm{find}}\:\boldsymbol{\mathrm{x}} \\ $$$$\:\boldsymbol{\mathrm{log}}\sqrt{\boldsymbol{{x}}}=\sqrt{\boldsymbol{\mathrm{log}{x}}} \\ $$

Question Number 32908 Answers: 2 Comments: 2

2018^(2017) > 2017^(2018) or 2018^(2017) < 2017^(2018) ?

$$\mathrm{2018}^{\mathrm{2017}} \:>\:\mathrm{2017}^{\mathrm{2018}} \:{or} \\ $$$$\mathrm{2018}^{\mathrm{2017}} \:<\:\mathrm{2017}^{\mathrm{2018}} \:\:? \\ $$

Question Number 32900 Answers: 0 Comments: 0

Question Number 32897 Answers: 3 Comments: 0

e^π > π^e or e^π <π^e ?

$$ \\ $$$${e}^{\pi} \:>\:\pi^{{e}} \:\:{or}\:\:\:{e}^{\pi} <\pi^{{e}} \:? \\ $$$$ \\ $$

Question Number 32891 Answers: 0 Comments: 3

Question Number 32889 Answers: 0 Comments: 1

Question Number 32885 Answers: 1 Comments: 0

Question Number 32878 Answers: 1 Comments: 0

A= [(α,1,1,1),(1,α,1,1),(1,1,β,1),(1,1,1,β) ]with α^2 ≠1≠β^2 det(A)=....???

$${A}=\begin{bmatrix}{\alpha}&{\mathrm{1}}&{\mathrm{1}}&{\mathrm{1}}\\{\mathrm{1}}&{\alpha}&{\mathrm{1}}&{\mathrm{1}}\\{\mathrm{1}}&{\mathrm{1}}&{\beta}&{\mathrm{1}}\\{\mathrm{1}}&{\mathrm{1}}&{\mathrm{1}}&{\beta}\end{bmatrix}{with}\:\alpha^{\mathrm{2}} \neq\mathrm{1}\neq\beta^{\mathrm{2}} \\ $$$${det}\left({A}\right)=....??? \\ $$

Question Number 32877 Answers: 1 Comments: 0

[(2,1),(0,2) ]^(2018) =.....???

$$\begin{bmatrix}{\mathrm{2}}&{\mathrm{1}}\\{\mathrm{0}}&{\mathrm{2}}\end{bmatrix}^{\mathrm{2018}} =.....??? \\ $$

Question Number 32872 Answers: 1 Comments: 0

Question Number 32870 Answers: 0 Comments: 0

Question Number 32869 Answers: 1 Comments: 1

A= (((2 0)),((1 2)) ) ;h and k are numbers so that A^2 =hA + kI,where I= (((1 0)),((0 1)) ). find the value of h and k.

$${A}=\begin{pmatrix}{\mathrm{2}\:\:\:\:\:\:\:\mathrm{0}}\\{\mathrm{1}\:\:\:\:\:\:\:\mathrm{2}}\end{pmatrix}\:;{h}\:\mathrm{and}\:{k}\:\mathrm{are}\:\mathrm{numbers}\:\mathrm{so} \\ $$$$\mathrm{that}\:\mathrm{A}^{\mathrm{2}} ={hA}\:+\:{kI},\mathrm{where}\:\mathrm{I}=\:\begin{pmatrix}{\mathrm{1}\:\:\:\:\:\:\:\:\mathrm{0}}\\{\mathrm{0}\:\:\:\:\:\:\:\:\mathrm{1}}\end{pmatrix}. \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{h}\:\mathrm{and}\:{k}. \\ $$

Question Number 32850 Answers: 0 Comments: 1

evaluate ∫_0 ^π 42(2)dx

$${evaluate} \\ $$$$\int_{\mathrm{0}} ^{\pi} \mathrm{42}\left(\mathrm{2}\right){dx} \\ $$

Question Number 32868 Answers: 0 Comments: 0

Question Number 32845 Answers: 1 Comments: 0

how many moles are there in 3g of Na_2_ CO_3

$${how}\:{many}\:{moles}\:{are}\:{there}\:{in}\:\mathrm{3}{g}\:{of} \\ $$$${Na}_{\mathrm{2}_{} } {CO}_{\mathrm{3}} \\ $$

Question Number 32844 Answers: 0 Comments: 1

sketch on the x−y plain the locus of a point,P which moves such that its x and y coordinates are same. state the locus.

$${sketch}\:{on}\:{the}\:{x}−{y}\:{plain}\:{the}\:{locus} \\ $$$${of}\:{a}\:{point},{P}\:{which}\:{moves}\:{such}\:{that} \\ $$$${its}\:{x}\:{and}\:\:{y}\:{coordinates}\:{are}\:{same}. \\ $$$${state}\:{the}\:{locus}. \\ $$

Question Number 32841 Answers: 1 Comments: 0

A+B+C=180^0 3sin A +4cosB =6 3cosA + 4sin B =(√(13)) sin C=....

$${A}+{B}+{C}=\mathrm{180}^{\mathrm{0}} \\ $$$$\mathrm{3}{sin}\:{A}\:+\mathrm{4}{cosB}\:=\mathrm{6} \\ $$$$\mathrm{3}{cosA}\:+\:\mathrm{4}{sin}\:{B}\:=\sqrt{\mathrm{13}} \\ $$$${sin}\:{C}=.... \\ $$

Question Number 32837 Answers: 0 Comments: 1

the length of the line segment joining A and B is (√(10)) .Given that its double the line segment joining the points (7,n) and (6,2) find the possible values of n.

$${the}\:{length}\:{of}\:{the}\:{line}\:{segment}\:{joining} \\ $$$${A}\:{and}\:{B}\:{is}\:\sqrt{\mathrm{10}}\:.{Given}\:{that}\:{its}\:{double} \\ $$$${the}\:{line}\:{segment}\:{joining}\:{the}\:{points} \\ $$$$\left(\mathrm{7},{n}\right)\:{and}\:\left(\mathrm{6},\mathrm{2}\right)\:{find}\:{the}\:{possible}\:{values}\:{of}\:{n}. \\ $$$$ \\ $$

Pg 1748 Pg 1749 Pg 1750 Pg 1751 Pg 1752 Pg 1753 Pg 1754 Pg 1755 Pg 1756 Pg 1757

Contact: info@tinkutara.com