
AllQuestion and Answers: Page 1752
Question Number 32889 Answers: 0 Comments: 1

Question Number 32885 Answers: 1 Comments: 0

Question Number 32878 Answers: 1 Comments: 0
Question Number 32877 Answers: 1 Comments: 0
Question Number 32872 Answers: 1 Comments: 0

Question Number 32870 Answers: 0 Comments: 0
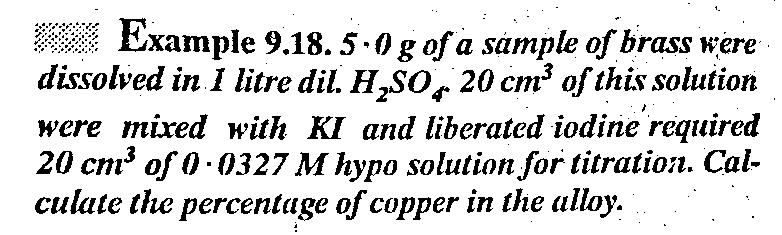
Question Number 32869 Answers: 1 Comments: 1
Question Number 32850 Answers: 0 Comments: 1
$${evaluate} \\ $$$$\int_{\mathrm{0}} ^{\pi} \mathrm{42}\left(\mathrm{2}\right){dx} \\ $$
Question Number 32868 Answers: 0 Comments: 0
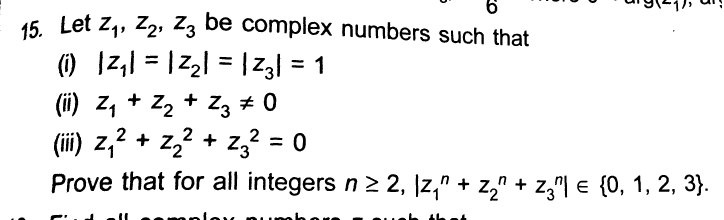
Question Number 32845 Answers: 1 Comments: 0
Question Number 32844 Answers: 0 Comments: 1
Question Number 32841 Answers: 1 Comments: 0
Question Number 32837 Answers: 0 Comments: 1
Question Number 32838 Answers: 2 Comments: 2
Question Number 32834 Answers: 1 Comments: 0
Question Number 32832 Answers: 0 Comments: 0
Question Number 32830 Answers: 0 Comments: 0
Question Number 32810 Answers: 1 Comments: 2
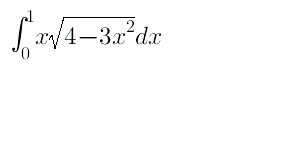
Question Number 33004 Answers: 0 Comments: 3
Question Number 32965 Answers: 0 Comments: 2

Question Number 32960 Answers: 1 Comments: 0
Question Number 32789 Answers: 0 Comments: 0
Question Number 32788 Answers: 0 Comments: 1
Question Number 32785 Answers: 1 Comments: 0
Question Number 32780 Answers: 2 Comments: 0
Question Number 32776 Answers: 1 Comments: 0
Pg 1747 Pg 1748 Pg 1749 Pg 1750 Pg 1751 Pg 1752 Pg 1753 Pg 1754 Pg 1755 Pg 1756
