Question and Answers Forum

AllQuestion and Answers: Page 1739

Question Number 33466 Answers: 1 Comments: 2

if tan𝛃=(r/(√s)) and sin𝛃=((√s)/r) show that cos𝛃=(√(r^2 +s))

$$\:\boldsymbol{\mathrm{if}}\:\boldsymbol{\mathrm{tan}\beta}=\frac{\boldsymbol{\mathrm{r}}}{\sqrt{\boldsymbol{\mathrm{s}}}}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{sin}\beta}=\frac{\sqrt{\boldsymbol{\mathrm{s}}}}{\boldsymbol{\mathrm{r}}} \\ $$$$\:\boldsymbol{\mathrm{show}}\:\boldsymbol{\mathrm{that}}\:\boldsymbol{\mathrm{cos}\beta}=\sqrt{\boldsymbol{\mathrm{r}}^{\mathrm{2}} +\boldsymbol{\mathrm{s}}} \\ $$

Question Number 33463 Answers: 0 Comments: 1

Find the pricipal and ordinary argument of z=(i/(−2−2i))

$${Find}\:{the}\:{pricipal}\:{and}\:{ordinary} \\ $$$${argument}\:{of}\:{z}=\frac{{i}}{−\mathrm{2}−\mathrm{2}{i}} \\ $$

Question Number 33462 Answers: 1 Comments: 0

using PMI show that ∀ n≥2 the number 5^n ends with the digits 25

$${using}\:{PMI}\:{show}\:{that}\:\forall\:{n}\geqslant\mathrm{2}\:{the} \\ $$$${number}\:\mathrm{5}^{{n}} \:{ends}\:{with}\:{the}\:{digits}\:\mathrm{25} \\ $$

Question Number 33460 Answers: 1 Comments: 0

why do we use greek letters like α,β,θ,π,Ω,ρ in mathematics

$$\:\:{why}\:{do}\:{we}\:{use}\:{greek}\:{letters}\:{like}\: \\ $$$$\alpha,\beta,\theta,\pi,\Omega,\rho\:\:\:{in}\:{mathematics} \\ $$

Question Number 33455 Answers: 1 Comments: 2

Please can someone help with a simplier method of solving this question Q1; Given that the expression x^3 +x^2 −4x +5 and x^3 +3x−7 leave same remainder when divided by (x−a) find the possible values of a

$$\:\:\:\:\:{Please}\:{can}\:{someone}\:{help}\:{with}\:{a} \\ $$$${simplier}\:{method}\:{of}\:{solving}\:{this}\:{question} \\ $$$${Q}\mathrm{1};\:\:\: \\ $$$$\:\:\:{Given}\:{that}\:{the}\:{expression}\:{x}^{\mathrm{3}} +{x}^{\mathrm{2}} −\mathrm{4}{x}\:+\mathrm{5} \\ $$$${and}\:{x}^{\mathrm{3}} +\mathrm{3}{x}−\mathrm{7}\:{leave}\:{same}\:{remainder} \\ $$$${when}\:{divided}\:{by}\:\left({x}−{a}\right)\:{find}\:{the}\:{possible} \\ $$$${values}\:{of}\:{a} \\ $$

Question Number 33452 Answers: 1 Comments: 2

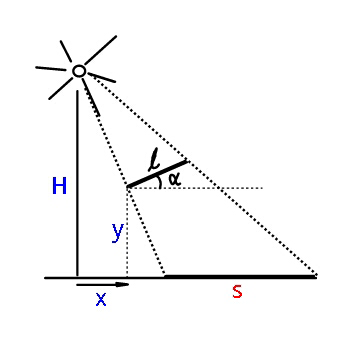
Question Number 33435 Answers: 1 Comments: 0

In a region an electric field exist in a given direction and it passes through a circle of radius R normally. The magnitude of electric field is given as : E = E_0 (1− (r/R)). where r is the distance from centre of circle .Find electric flux through plane of circle within it.

$$\boldsymbol{{I}}{n}\:{a}\:{region}\:{an}\:{electric}\:{field}\:{exist}\:{in}\:{a}\:{given} \\ $$$${direction}\:{and}\:{it}\:{passes}\:{through}\:{a}\:{circle} \\ $$$${of}\:{radius}\:{R}\:{normally}.\:{The}\:{magnitude} \\ $$$${of}\:{electric}\:{field}\:{is}\:{given}\:{as}\:: \\ $$$$\boldsymbol{{E}}\:=\:{E}_{\mathrm{0}} \:\left(\mathrm{1}−\:\frac{{r}}{\boldsymbol{{R}}}\right).\:{where}\:{r}\:{is}\:{the}\:{distance} \\ $$$${from}\:{centre}\:{of}\:{circle}\:.{Find}\:{electric}\: \\ $$$${flux}\:{through}\:{plane}\:{of}\:{circle}\:{within}\:{it}. \\ $$

Question Number 33430 Answers: 0 Comments: 3

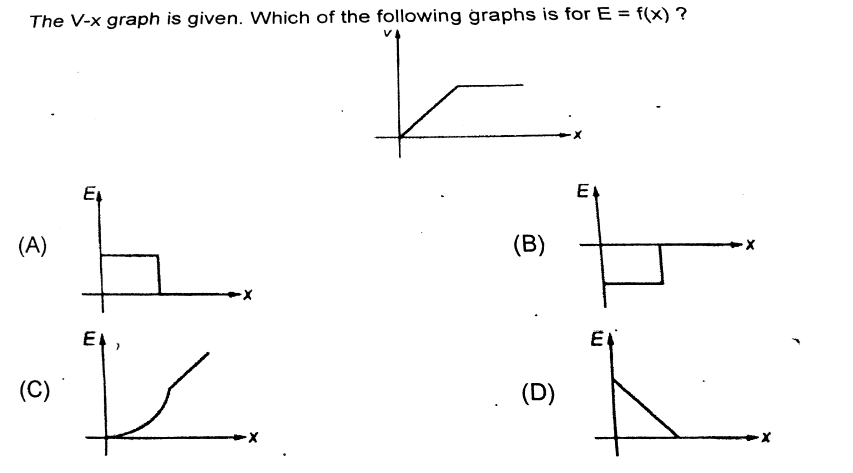
Question Number 33425 Answers: 1 Comments: 3

find k if the deteminant of (((3 k)),((2 3)) ) is 2 can someone please teach me how to find the deteminant of a 3×3 matrix ?

$${find}\:{k}\:{if}\:{the}\:{deteminant}\:{of}\: \\ $$$$\:\:\:\begin{pmatrix}{\mathrm{3}\:\:\:\:\:\:\:\:\:{k}}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:\:\mathrm{3}}\end{pmatrix}\:\:\:{is}\:\mathrm{2}\: \\ $$$${can}\:{someone}\:{please}\:{teach}\:{me}\:{how} \\ $$$${to}\:{find}\:{the}\:{deteminant}\:{of}\:{a}\:\mathrm{3}×\mathrm{3} \\ $$$${matrix}\:? \\ $$$$\: \\ $$$$\:\: \\ $$

Question Number 33413 Answers: 0 Comments: 2

the value of θ,in the range 0° ≤ θ≤90° for which sinθ = cos θ is...?

$$ \\ $$$${the}\:{value}\:{of}\:\theta,{in}\:{the}\:{range}\:\mathrm{0}°\:\leqslant\:\:\theta\leqslant\mathrm{90}° \\ $$$${for}\:{which}\:{sin}\theta\:=\:{cos}\:\theta\:{is}...? \\ $$

Question Number 33411 Answers: 0 Comments: 1

Given that u and v are real valued functions in x ,then (d/dx)((u/v)) is equal to?

$$\:\mathrm{Given}\:\mathrm{that}\:{u}\:\mathrm{and}\:{v}\:\mathrm{are}\:\mathrm{real}\:\mathrm{valued} \\ $$$$\mathrm{functions}\:\mathrm{in}\:{x}\:,\mathrm{then}\:\frac{{d}}{{dx}}\left(\frac{{u}}{{v}}\right)\:{is}\:{equal}\:{to}? \\ $$

Question Number 33410 Answers: 1 Comments: 1

please is there any general way for calculating the error or uncertainty in g when m=((4π^2 )/g) where m=slope and g=acceleration due to gravity please help

$${please}\:{is}\:{there}\:{any}\:{general}\:{way}\:{for} \\ $$$${calculating}\:{the}\:{error}\:{or}\:{uncertainty} \\ $$$${in}\:{g}\:{when} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:{m}=\frac{\mathrm{4}\pi^{\mathrm{2}} }{{g}}\:{where}\:{m}={slope}\:{and} \\ $$$${g}={acceleration}\:{due}\:{to}\:{gravity} \\ $$$$ \\ $$$$ \\ $$$${please}\:{help} \\ $$

Question Number 33407 Answers: 1 Comments: 0

if y=x! find dy/dx

$${if}\:{y}={x}!\:{find}\:{dy}/{dx} \\ $$

Question Number 33406 Answers: 0 Comments: 4

Find the half derivative of y=ln x

$${Find}\:{the}\:{half}\:{derivative}\:{of}\:{y}=\mathrm{ln}\:{x} \\ $$

Question Number 33400 Answers: 0 Comments: 4

Find out electric field on an axial position due to a ring having linear charge density 𝛌= λ_0 cos θ .

$$\boldsymbol{{Find}}\:{out}\:{electric}\:{field}\:{on}\:{an}\:{axial}\: \\ $$$${position}\:{due}\:{to}\:{a}\:{ring}\:{having}\:{linear} \\ $$$${charge}\:{density}\:\boldsymbol{\lambda}=\:\lambda_{\mathrm{0}} \:\mathrm{cos}\:\theta\:. \\ $$

Question Number 33375 Answers: 0 Comments: 2

If f:R → R is an odd function such that : a) f(1+x) = 1+f(x) . b) x^2 f((1/x)) = f(x) , x≠0. Then find f(x) ?

$${If}\:{f}:{R}\:\rightarrow\:{R}\:{is}\:{an}\:\boldsymbol{{odd}}\:{function}\:{such} \\ $$$${that}\:: \\ $$$$\left.{a}\right)\:{f}\left(\mathrm{1}+{x}\right)\:=\:\mathrm{1}+{f}\left({x}\right)\:. \\ $$$$\left.{b}\right)\:{x}^{\mathrm{2}} \:{f}\left(\frac{\mathrm{1}}{{x}}\right)\:=\:{f}\left({x}\right)\:,\:{x}\neq\mathrm{0}. \\ $$$${Then}\:{find}\:\boldsymbol{{f}}\left(\boldsymbol{{x}}\right)\:? \\ $$

Question Number 33369 Answers: 1 Comments: 0

Prove that gcd( gcd(A,B),gcd(B,C),gcd(C,A) ) =gcd(A,B,C)

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\:\:\mathrm{gcd}\left(\:\:\mathrm{gcd}\left(\mathrm{A},\mathrm{B}\right),\mathrm{gcd}\left(\mathrm{B},\mathrm{C}\right),\mathrm{gcd}\left(\mathrm{C},\mathrm{A}\right)\:\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{gcd}\left(\mathrm{A},\mathrm{B},\mathrm{C}\right) \\ $$

Question Number 33363 Answers: 0 Comments: 3

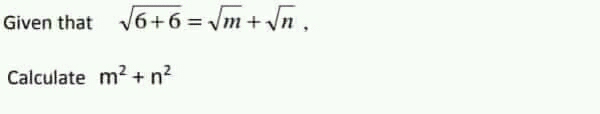
Question Number 33362 Answers: 0 Comments: 1

calculate by residus theorem I = ∫_(−∞) ^(+∞) ((cos(πx))/((1+x +x^2 )))dx .

$${calculate}\:{by}\:{residus}\:{theorem} \\ $$$${I}\:=\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{cos}\left(\pi{x}\right)}{\left(\mathrm{1}+{x}\:+{x}^{\mathrm{2}} \right)}{dx}\:. \\ $$

Question Number 33359 Answers: 0 Comments: 0

let consider the serie Σ_(n≥1) sin((1/(√n)))x^n 1) find the radius of convergence 2)study the convergence at −R and R 3) let S(x)its sum study the continuity of S 4) prove that (1−x)_(x→1^− ) S(x)→0

$${let}\:{consider}\:{the}\:{serie}\:\sum_{{n}\geqslant\mathrm{1}} {sin}\left(\frac{\mathrm{1}}{\sqrt{{n}}}\right){x}^{{n}} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{the}\:{radius}\:{of}\:{convergence} \\ $$$$\left.\mathrm{2}\right){study}\:{the}\:{convergence}\:{at}\:−{R}\:{and}\:{R} \\ $$$$\left.\mathrm{3}\right)\:{let}\:{S}\left({x}\right){its}\:{sum}\:{study}\:{the}\:{continuity} \\ $$$${of}\:{S} \\ $$$$\left.\mathrm{4}\right)\:{prove}\:{that}\:\left(\mathrm{1}−{x}\right)_{{x}\rightarrow\mathrm{1}^{−} } {S}\left({x}\right)\rightarrow\mathrm{0} \\ $$

Question Number 33358 Answers: 0 Comments: 0

prove that Σ_(p=1) ^∞ (z^p /(1+z^p )) =Σ_(q=1) ^∞ (−1)^(q−1) (z^q /(1−z^q ))

$${prove}\:{that}\:\sum_{{p}=\mathrm{1}} ^{\infty} \:\frac{{z}^{{p}} }{\mathrm{1}+{z}^{{p}} }\:=\sum_{{q}=\mathrm{1}} ^{\infty} \:\left(−\mathrm{1}\right)^{{q}−\mathrm{1}} \frac{{z}^{{q}} }{\mathrm{1}−{z}^{{q}} } \\ $$

Question Number 33357 Answers: 0 Comments: 0

find the rsdius of convergence for the serie Σ_(n=1) ^∞ (1 +(1/n))^n^2 x^n

$${find}\:{the}\:{rsdius}\:{of}\:{convergence}\:{for} \\ $$$${the}\:{serie}\:\sum_{{n}=\mathrm{1}} ^{\infty} \left(\mathrm{1}\:+\frac{\mathrm{1}}{{n}}\right)^{{n}^{\mathrm{2}} } \:{x}^{{n}} \: \\ $$$$ \\ $$

Question Number 33356 Answers: 0 Comments: 0

find the radius of Σ_(n≥0) ((n^2 +n)/(2^n +n!)) x^n

$${find}\:{the}\:{radius}\:{of}\:\sum_{{n}\geqslant\mathrm{0}} \frac{{n}^{\mathrm{2}} \:+{n}}{\mathrm{2}^{{n}} \:+{n}!}\:{x}^{{n}} \\ $$

Question Number 33355 Answers: 0 Comments: 0

let a≥1 find the radius of Σ_(n≥1) arc cos(1−(1/n^a ))z^n