
AllQuestion and Answers: Page 1707
Question Number 36692 Answers: 2 Comments: 1
Question Number 36691 Answers: 0 Comments: 2

Question Number 36689 Answers: 0 Comments: 1
Question Number 36690 Answers: 0 Comments: 1
Question Number 36677 Answers: 4 Comments: 3
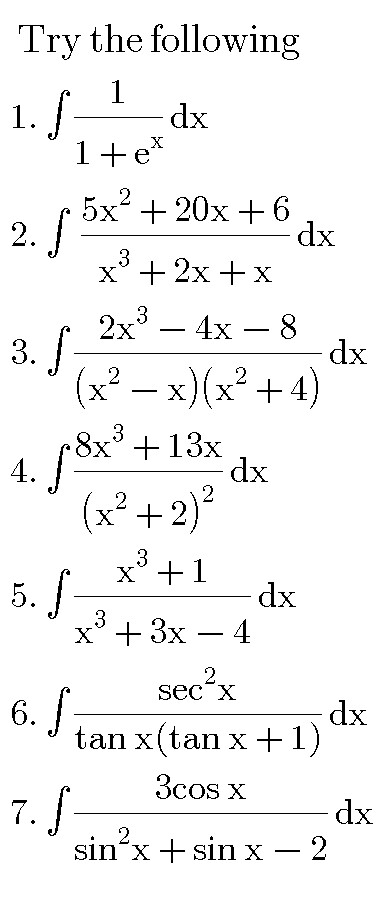
Question Number 36676 Answers: 1 Comments: 3
Question Number 36661 Answers: 0 Comments: 2

Question Number 36660 Answers: 1 Comments: 0
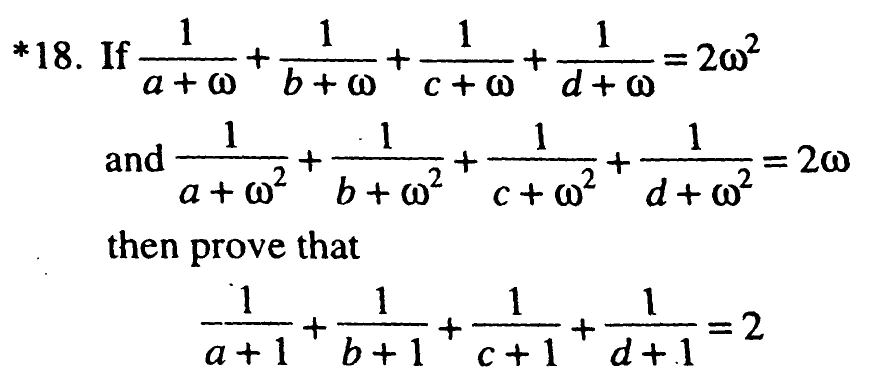
Question Number 36649 Answers: 1 Comments: 4
$$\int\:\frac{\mathrm{1}}{{x}^{\mathrm{4}} +\mathrm{1}}\:{dx} \\ $$
Question Number 36643 Answers: 2 Comments: 1
$$\int\:\frac{{x}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}\:{dx}\:=\:? \\ $$
Question Number 36634 Answers: 0 Comments: 5

Question Number 36633 Answers: 0 Comments: 1
Question Number 36630 Answers: 0 Comments: 1
Question Number 36629 Answers: 0 Comments: 0

Question Number 36626 Answers: 0 Comments: 1

Question Number 36625 Answers: 0 Comments: 0

Question Number 36623 Answers: 0 Comments: 0

Question Number 36622 Answers: 0 Comments: 0

Question Number 36613 Answers: 1 Comments: 5

Question Number 36601 Answers: 0 Comments: 2
Question Number 36597 Answers: 2 Comments: 2
Question Number 36659 Answers: 1 Comments: 3
Question Number 36595 Answers: 2 Comments: 0
Question Number 36590 Answers: 1 Comments: 1

Question Number 36587 Answers: 0 Comments: 0
Question Number 36576 Answers: 1 Comments: 1

Pg 1702 Pg 1703 Pg 1704 Pg 1705 Pg 1706 Pg 1707 Pg 1708 Pg 1709 Pg 1710 Pg 1711
