
AllQuestion and Answers: Page 1704
Question Number 36923 Answers: 0 Comments: 1
Question Number 36922 Answers: 0 Comments: 1
Question Number 36921 Answers: 0 Comments: 0
Question Number 36920 Answers: 0 Comments: 1
Question Number 36919 Answers: 0 Comments: 1
Question Number 36918 Answers: 0 Comments: 0
Question Number 36917 Answers: 0 Comments: 1
Question Number 36916 Answers: 0 Comments: 1
Question Number 36915 Answers: 0 Comments: 1
Question Number 36912 Answers: 0 Comments: 1
Question Number 36911 Answers: 0 Comments: 1
Question Number 36910 Answers: 0 Comments: 0
Question Number 36909 Answers: 0 Comments: 0
Question Number 36908 Answers: 0 Comments: 0
Question Number 36907 Answers: 0 Comments: 0
Question Number 36969 Answers: 1 Comments: 0
Question Number 36905 Answers: 0 Comments: 0
Question Number 36904 Answers: 0 Comments: 1
Question Number 36903 Answers: 0 Comments: 0
Question Number 36892 Answers: 1 Comments: 1
Question Number 36886 Answers: 0 Comments: 1

Question Number 36884 Answers: 0 Comments: 0
Question Number 36880 Answers: 1 Comments: 3

Question Number 36877 Answers: 0 Comments: 0

Question Number 36876 Answers: 0 Comments: 0
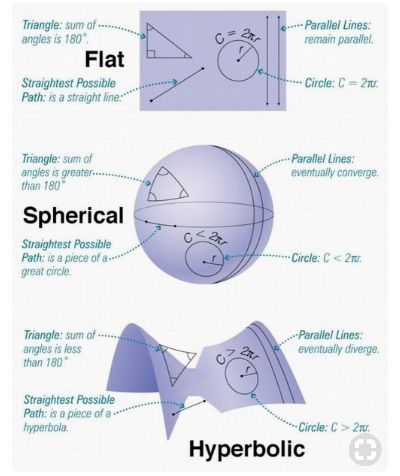
Question Number 36874 Answers: 0 Comments: 0

Pg 1699 Pg 1700 Pg 1701 Pg 1702 Pg 1703 Pg 1704 Pg 1705 Pg 1706 Pg 1707 Pg 1708
