
AllQuestion and Answers: Page 1699
Question Number 37283 Answers: 0 Comments: 1
Question Number 37282 Answers: 0 Comments: 5
Question Number 37281 Answers: 0 Comments: 1
Question Number 37280 Answers: 0 Comments: 1
Question Number 37279 Answers: 1 Comments: 1
Question Number 37278 Answers: 0 Comments: 1
Question Number 37277 Answers: 0 Comments: 3
Question Number 37276 Answers: 0 Comments: 0
Question Number 37275 Answers: 0 Comments: 0
Question Number 37273 Answers: 0 Comments: 0
Question Number 37272 Answers: 0 Comments: 0
Question Number 37271 Answers: 0 Comments: 2
Question Number 37270 Answers: 1 Comments: 0
Question Number 37269 Answers: 0 Comments: 2
Question Number 37263 Answers: 1 Comments: 1

Question Number 37258 Answers: 1 Comments: 0
$$\int\:\frac{{x}^{\mathrm{3}} +\mathrm{1}}{\sqrt{{x}^{\mathrm{2}} +{x}}}\:{dx}\:=\:? \\ $$
Question Number 37252 Answers: 1 Comments: 1
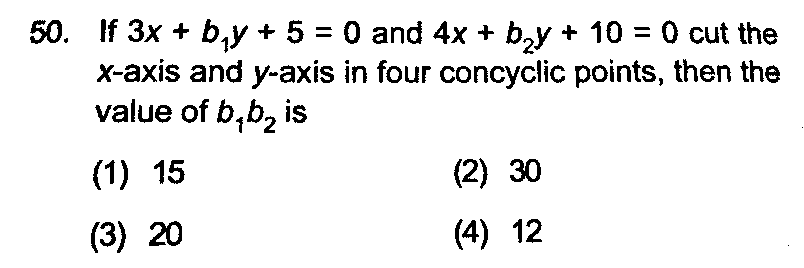
Question Number 37540 Answers: 1 Comments: 0
Question Number 37249 Answers: 0 Comments: 0
Question Number 37244 Answers: 1 Comments: 0
Question Number 37243 Answers: 0 Comments: 0
Question Number 37237 Answers: 1 Comments: 1
Question Number 37236 Answers: 0 Comments: 0
Question Number 37235 Answers: 1 Comments: 0
Question Number 37233 Answers: 0 Comments: 0
Question Number 37232 Answers: 0 Comments: 0
Pg 1694 Pg 1695 Pg 1696 Pg 1697 Pg 1698 Pg 1699 Pg 1700 Pg 1701 Pg 1702 Pg 1703
