Question and Answers Forum

AllQuestion and Answers: Page 1697

Question Number 37139 Answers: 2 Comments: 0

if α , β are the roots of the quadratic equation ax^2 +bx+c =0 then find the quadratic equation whose roots are α^(2 ) , β^2

$${if}\:\alpha\:,\:\beta\:\:{are}\:{the}\:{roots}\:{of}\:{the}\:{quadratic} \\ $$$${equation}\:{ax}^{\mathrm{2}} +{bx}+{c}\:=\mathrm{0}\:{then}\:\:{find} \\ $$$${the}\:{quadratic}\:{equation}\:{whose}\:{roots} \\ $$$${are}\:\:\alpha^{\mathrm{2}\:\:\:} ,\:\beta^{\mathrm{2}} \\ $$$$ \\ $$$$ \\ $$

Question Number 37137 Answers: 2 Comments: 4

Find minimum distance between y^2 =8x and x^2 +(y+6)^2 =1.

$$\mathrm{Find}\:\mathrm{minimum}\:\mathrm{distance}\:\mathrm{between} \\ $$$$\mathrm{y}^{\mathrm{2}} =\mathrm{8}{x}\:{and}\:{x}^{\mathrm{2}} +\left({y}+\mathrm{6}\right)^{\mathrm{2}} =\mathrm{1}. \\ $$

Question Number 37129 Answers: 0 Comments: 0

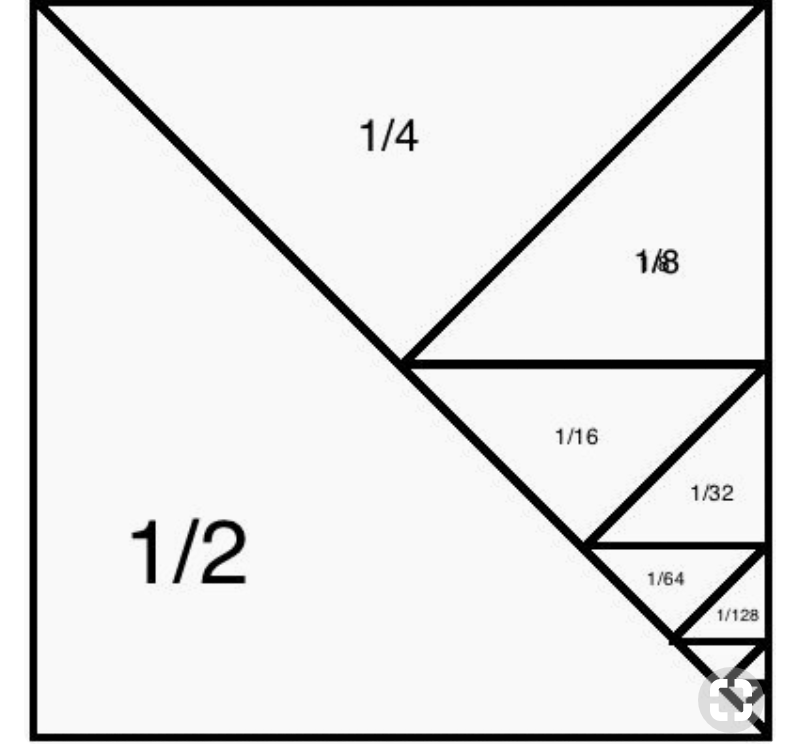
Question Number 37128 Answers: 0 Comments: 0

Question Number 37511 Answers: 0 Comments: 3

To the developer of Tinku Tara: dear sir: for some unknown reasons I don′t get any notification from the app when a post, in which I am involved, has been updated. Where is the problem and how can I solve it? Thank you!

$${To}\:{the}\:{developer}\:{of}\:{Tinku}\:{Tara}: \\ $$$${dear}\:{sir}:\:{for}\:{some}\:{unknown}\:{reasons} \\ $$$${I}\:{don}'{t}\:{get}\:{any}\:{notification}\:{from}\:{the} \\ $$$${app}\:{when}\:{a}\:{post},\:{in}\:{which}\:{I}\:{am}\:{involved}, \\ $$$${has}\:{been}\:{updated}.\:{Where}\:{is}\:{the} \\ $$$${problem}\:{and}\:{how}\:{can}\:{I}\:{solve}\:{it}? \\ $$$${Thank}\:{you}! \\ $$

Question Number 37123 Answers: 0 Comments: 1

draw ΔAB^ C and its image ΔA′B^′ C^′ after a reflection in line y=x if A(0,3),B(3,0),C(3,2).what is the line of symmetry of the two figures?

$${draw}\:\Delta{A}\overset{} {{B}C}\:{and}\:{its}\:{image}\:\:\Delta{A}'{B}^{'} {C}^{'} \: \\ $$$${after}\:{a}\:{reflection}\:{in}\:{line}\:{y}={x} \\ $$$${if}\:{A}\left(\mathrm{0},\mathrm{3}\right),{B}\left(\mathrm{3},\mathrm{0}\right),{C}\left(\mathrm{3},\mathrm{2}\right).{what}\:{is}\:{the} \\ $$$${line}\:{of}\:{symmetry}\:\:{of}\:{the}\:{two}\:{figures}? \\ $$

Question Number 37122 Answers: 0 Comments: 0

draw ΔAB^ C and its image ΔA′B^′ C^′ after a reflection in line y=x if A(0,3),B(3,0),C(3,2).what is the line of symmetry of the two figures?

Question Number 37110 Answers: 1 Comments: 2

prove that (cos𝛝−sin𝛝)^2 +(cosec𝛝+sin𝛝)^2 =2

$$\boldsymbol{\mathrm{prove}}\:\boldsymbol{\mathrm{that}} \\ $$$$\left(\boldsymbol{\mathrm{cos}\vartheta}−\boldsymbol{\mathrm{sin}\vartheta}\right)^{\mathrm{2}} +\left(\boldsymbol{\mathrm{cosec}\vartheta}+\boldsymbol{\mathrm{sin}\vartheta}\right)^{\mathrm{2}} =\mathrm{2} \\ $$

Question Number 37108 Answers: 1 Comments: 0

If 4x+8cos x+tan x−2sec x−4log {cosx(1+sin x)}≥6 ∀ x ε [0,ψ) then largest value of ψ is ?

$$\mathrm{If}\:\mathrm{4}{x}+\mathrm{8cos}\:{x}+\mathrm{tan}\:{x}−\mathrm{2sec}\:{x}−\mathrm{4log}\:\left\{\mathrm{cos}{x}\left(\mathrm{1}+\mathrm{sin}\:{x}\right)\right\}\geqslant\mathrm{6} \\ $$$$\forall\:{x}\:\epsilon\:\left[\mathrm{0},\psi\right)\:\mathrm{then}\:\mathrm{largest}\:\mathrm{value}\:\mathrm{of}\:\psi\:\mathrm{is}\:? \\ $$

Question Number 37089 Answers: 1 Comments: 10

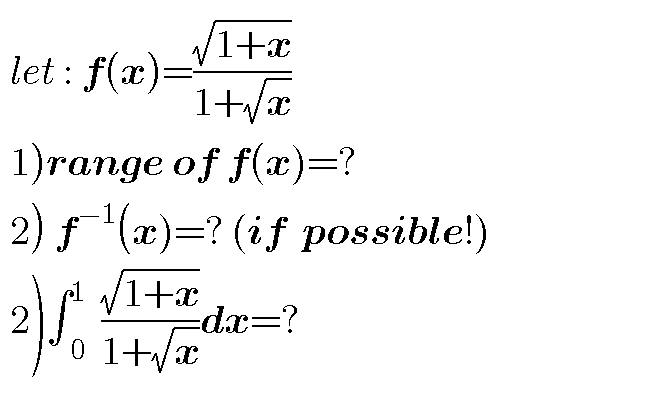
Question Number 37084 Answers: 0 Comments: 2

solve using matrix method x − y= 4 2x − 3y= 5

$$\:\:{solve}\:{using}\:{matrix}\:{method} \\ $$$$\:\:\:\:\:\:{x}\:−\:{y}=\:\mathrm{4} \\ $$$$\:\:\:\:\:\mathrm{2}{x}\:−\:\mathrm{3}{y}=\:\mathrm{5} \\ $$

Question Number 37081 Answers: 0 Comments: 1

Question Number 37079 Answers: 1 Comments: 0

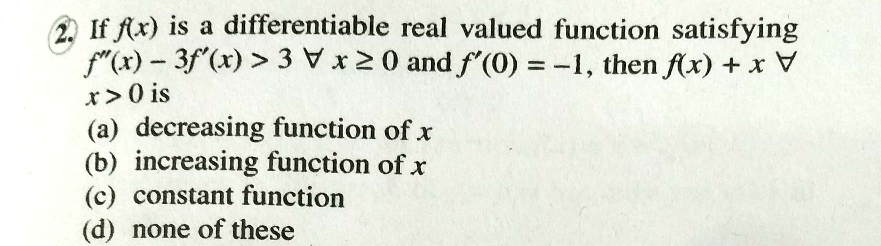
Question Number 37073 Answers: 2 Comments: 2

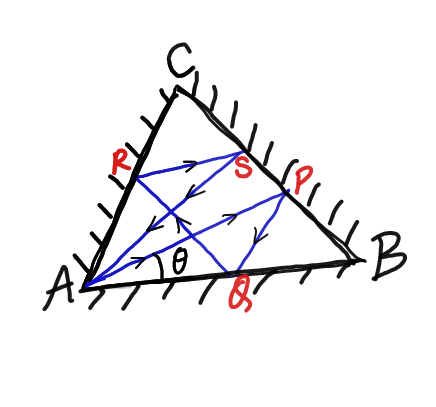
Question Number 37071 Answers: 2 Comments: 1

find the value of ∫_0 ^(π/2) ((xdx)/(1+cosx))

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{xdx}}{\mathrm{1}+{cosx}} \\ $$

Question Number 37067 Answers: 2 Comments: 1

find ∫ (dx/((x+1)(√(1+x^2 ))))

$${find}\:\int\:\:\:\:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$

Question Number 37059 Answers: 0 Comments: 0

Question Number 37058 Answers: 1 Comments: 0

Question Number 37048 Answers: 0 Comments: 2

to everybody: if you solved an integral, please test your solution by differentiating. I′m doing the same. thank you so much! MJS

$$\mathrm{to}\:\mathrm{everybody}: \\ $$$$\mathrm{if}\:\mathrm{you}\:\mathrm{solved}\:\mathrm{an}\:\mathrm{integral},\:\mathrm{please}\:\mathrm{test}\:\mathrm{your} \\ $$$$\mathrm{solution}\:\mathrm{by}\:\mathrm{differentiating}.\:\mathrm{I}'\mathrm{m}\:\mathrm{doing}\:\mathrm{the} \\ $$$$\mathrm{same}.\:\mathrm{thank}\:\mathrm{you}\:\mathrm{so}\:\mathrm{much}! \\ $$$${MJS} \\ $$

Question Number 37045 Answers: 1 Comments: 1

Question Number 37037 Answers: 4 Comments: 0

If ((a+b−c)/(a+b)) = ((b+c−a)/(b+c)) =((c+a−b)/(c+a)) and a+b+c ≠ 0 then which of the following is true 1) a=−b=c 2)−a=−b=c 3) a=b=c 4) a=b≠c

$${If}\:\frac{{a}+{b}−{c}}{{a}+{b}}\:=\:\frac{{b}+{c}−{a}}{{b}+{c}}\:=\frac{{c}+{a}−{b}}{{c}+{a}}\:{and}\: \\ $$$$\mathrm{a}+\mathrm{b}+\mathrm{c}\:\neq\:\mathrm{0}\:{then}\:{which}\:{of}\:{the}\:{following} \\ $$$$\left.{i}\left.{s}\:{true}\:\mathrm{1}\right)\:{a}=−{b}={c}\:\mathrm{2}\right)−{a}=−{b}={c}\: \\ $$$$\left.\mathrm{3}\left.\right)\:\mathrm{a}=\mathrm{b}=\mathrm{c}\:\mathrm{4}\right)\:\mathrm{a}=\mathrm{b}\neq{c} \\ $$

Question Number 37036 Answers: 0 Comments: 3

∫_0 ^( a) (1−((b−x)/(√((b−x)^2 +cx)))) dx = ?

$$\int_{\mathrm{0}} ^{\:\:{a}} \left(\mathrm{1}−\frac{{b}−{x}}{\sqrt{\left({b}−{x}\right)^{\mathrm{2}} +{cx}}}\right)\:{dx}\:=\:? \\ $$

Question Number 37032 Answers: 1 Comments: 1

Question Number 37028 Answers: 1 Comments: 0

∫((2x−1)/(5x^2 −x+2)) dx = ?

$$\int\frac{\mathrm{2}{x}−\mathrm{1}}{\mathrm{5}{x}^{\mathrm{2}} −{x}+\mathrm{2}}\:{dx}\:\:=\:\:? \\ $$

Question Number 37026 Answers: 0 Comments: 0

Question Number 37023 Answers: 0 Comments: 0

Pg 1692 Pg 1693 Pg 1694 Pg 1695 Pg 1696 Pg 1697 Pg 1698 Pg 1699 Pg 1700 Pg 1701

Contact: info@tinkutara.com