Question and Answers Forum

Qn:(a) given A and B are disjoint sets shade in venn diagram (i)B∩C (ii)A−(B∪C) (iii)(B∪C)−A Qn:(b) Given that ∣A∣=19 ∣B∣=23 ∣C∣=24 ∣A∪B∣=30 ∣(A∩B)−C∣=5 ∣(B∩C)∣=10 ∣(A∪B)^′ ∣=20 and ∣C−(A∪B)∣=10 then find (i)∣(A∩C)∣ (ii)∣(B−′C)∣′ (iii)∣𝛍∣ (iv)∣(B′∪C)∩A′∣

Question Number 33660 Answers: 4 Comments: 0

from sinhu=tan𝛝 prove that (i)tanh(u/2)=tan(𝛝/2) (ii)coshu=sec𝛝 (iii)u=log(sec𝛝+tan𝛝) (iv)tanhu=sin𝛝

Question Number 33659 Answers: 1 Comments: 0

solve for x and y coshy−7sinhx=3 and coshy−3sinh^2 x=2

$$\:\boldsymbol{\mathrm{solve}}\:\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{x}}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{y}} \\ $$$$\:\boldsymbol{\mathrm{cosh}{y}}−\mathrm{7}\boldsymbol{\mathrm{sinh}{x}}=\mathrm{3}\:\boldsymbol{\mathrm{and}} \\ $$$$\:\boldsymbol{\mathrm{cosh}{y}}−\mathrm{3}\boldsymbol{\mathrm{sinh}}^{\mathrm{2}} \boldsymbol{{x}}=\mathrm{2} \\ $$

Question Number 33658 Answers: 1 Comments: 1

If f(x)= x^3 −3x+1 then find number of different real solutions of f(f(x))=0 ?

$$\boldsymbol{{I}}{f}\:{f}\left({x}\right)=\:{x}^{\mathrm{3}} −\mathrm{3}{x}+\mathrm{1} \\ $$$${then}\:{find}\:{number}\:{of}\:{different}\:\:\:{real} \\ $$$${solutions}\:{of}\:{f}\left({f}\left({x}\right)\right)=\mathrm{0}\:? \\ $$

Question Number 33651 Answers: 1 Comments: 0

Let function f(x) be defined as f(x)= x^2 +bx+c , where b,c∈R . And f(1) − 2f(5) +f(9) =32. Find no. of ordered pairs (b,c) such that ∣f(x)∣≤8 ∀ x∈ [1,9] ?

$${Let}\:{function}\:{f}\left({x}\right)\:{be}\:{defined}\:{as}\: \\ $$$${f}\left({x}\right)=\:{x}^{\mathrm{2}} +{bx}+{c}\:,\:{where}\:{b},{c}\in{R}\:. \\ $$$${And}\:{f}\left(\mathrm{1}\right)\:−\:\mathrm{2}{f}\left(\mathrm{5}\right)\:+{f}\left(\mathrm{9}\right)\:=\mathrm{32}. \\ $$$${Find}\:{no}.\:{of}\:{ordered}\:{pairs}\:\left({b},{c}\right) \\ $$$${such}\:{that}\:\mid{f}\left({x}\right)\mid\leqslant\mathrm{8}\:\forall\:{x}\in\:\left[\mathrm{1},\mathrm{9}\right]\:? \\ $$

Question Number 33649 Answers: 1 Comments: 3

Consider f:R^+ →R such that f(3)=1 for a∈R^+ and f(x).f(y) + f((3/x)).f((3/y)) = 2f(xy) ∀ x,y ∈ R^+ . Then find f(x) ?

$${Consider}\:{f}:{R}^{+} \rightarrow{R}\:{such}\:{that} \\ $$$${f}\left(\mathrm{3}\right)=\mathrm{1}\:{for}\:{a}\in{R}^{+} \:{and}\: \\ $$$${f}\left({x}\right).{f}\left({y}\right)\:+\:{f}\left(\frac{\mathrm{3}}{{x}}\right).{f}\left(\frac{\mathrm{3}}{{y}}\right)\:=\:\mathrm{2}{f}\left({xy}\right) \\ $$$$\forall\:{x},{y}\:\in\:{R}^{+} .\:{Then}\:{find}\:{f}\left({x}\right)\:? \\ $$

Question Number 33644 Answers: 1 Comments: 0

∫(sec^2 x)e^(tanx) dx

$$\:\int\left(\boldsymbol{\mathrm{sec}}^{\mathrm{2}} \boldsymbol{{x}}\right)\boldsymbol{{e}}^{\boldsymbol{\mathrm{tan}{x}}} \boldsymbol{{dx}} \\ $$

Question Number 33643 Answers: 1 Comments: 0

∫((2x+3)/(x^2 +4))dx

$$\:\int\frac{\mathrm{2}\boldsymbol{{x}}+\mathrm{3}}{\boldsymbol{{x}}^{\mathrm{2}} +\mathrm{4}}\boldsymbol{{dx}} \\ $$

Question Number 33629 Answers: 1 Comments: 2

Question Number 33628 Answers: 0 Comments: 4

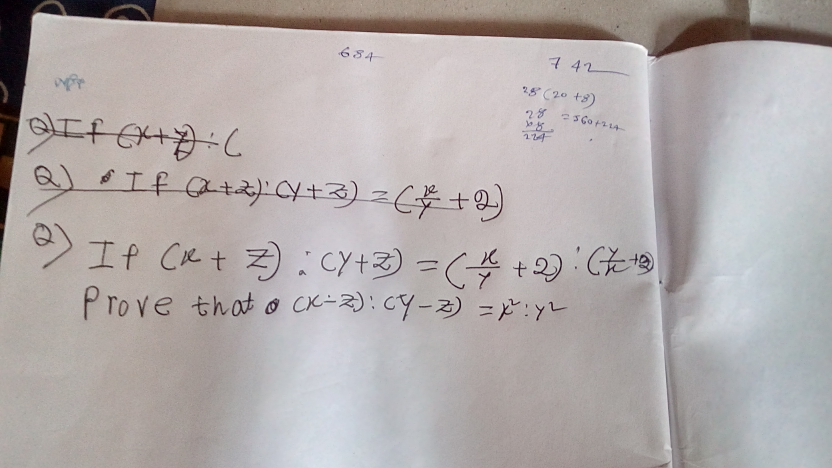
Question Number 33622 Answers: 0 Comments: 0

Question Number 33619 Answers: 1 Comments: 3

∫x^(5/2) (1−x)^(3/2) dx

$$\int{x}^{\mathrm{5}/\mathrm{2}} \left(\mathrm{1}−{x}\right)^{\mathrm{3}/\mathrm{2}} {dx} \\ $$

Question Number 33616 Answers: 1 Comments: 4

please help me please is there any app for practicing calculus for a CBT exam.I mean one that has a timer so I can asses my speed.

$${please}\:{help}\:{me} \\ $$$$ \\ $$$${please}\:{is}\:{there}\:{any}\:{app}\:{for}\:{practicing} \\ $$$${calculus}\:{for}\:\:{a}\:{CBT}\:{exam}.{I}\:{mean} \\ $$$${one}\:{that}\:{has}\:{a}\:{timer}\:{so}\:{I}\:{can}\:{asses} \\ $$$${my}\:{speed}. \\ $$

Question Number 33599 Answers: 1 Comments: 2

calculatef(a)= ∫_(−a) ^a (dx/((t^2 +x^2 )^(3/2) )) with a>0 .

$${calculatef}\left({a}\right)=\:\:\int_{−{a}} ^{{a}} \:\:\:\:\frac{{dx}}{\left({t}^{\mathrm{2}} \:+{x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\:\:{with}\:{a}>\mathrm{0}\:. \\ $$

Question Number 33597 Answers: 0 Comments: 0

study and give the graph of f(x) =e^(2/(lnx)) .

$${study}\:{and}\:{give}\:{the}\:{graph}\:{of}\:{f}\left({x}\right)\:={e}^{\frac{\mathrm{2}}{{lnx}}} \:. \\ $$

Question Number 33596 Answers: 0 Comments: 0

1) prove that ∀(a,b)∈R^2 ∣sinb −sina∣≤∣b−a∣ 2)let give the sequence x_0 =0 and x_(n+1) =a +(1/2)sin(x_n ) prove that for m≥n ∣x_m −x_n ∣ ≤ ((∣a∣)/2^(n−1) ) 3) prove that (x_n ) is convergent and its limit is solution of the equation x = a +(1/2) sinx .

$$\left.\mathrm{1}\right)\:{prove}\:{that}\:\forall\left({a},{b}\right)\in{R}^{\mathrm{2}} \:\:\:\:\mid{sinb}\:−{sina}\mid\leqslant\mid{b}−{a}\mid \\ $$$$\left.\mathrm{2}\right){let}\:{give}\:{the}\:{sequence}\:\:{x}_{\mathrm{0}} =\mathrm{0}\:{and} \\ $$$${x}_{{n}+\mathrm{1}} ={a}\:+\frac{\mathrm{1}}{\mathrm{2}}{sin}\left({x}_{{n}} \right)\:{prove}\:{that}\:{for}\:{m}\geqslant{n} \\ $$$$\mid{x}_{{m}} \:−{x}_{{n}} \mid\:\leqslant\:\:\frac{\mid{a}\mid}{\mathrm{2}^{{n}−\mathrm{1}} } \\ $$$$\left.\mathrm{3}\right)\:{prove}\:{that}\:\left({x}_{{n}} \right)\:{is}\:{convergent}\:{and}\:{its}\:{limit}\:{is}\:{solution} \\ $$$${of}\:{the}\:{equation}\:\:{x}\:=\:{a}\:+\frac{\mathrm{1}}{\mathrm{2}}\:{sinx}\:. \\ $$

Question Number 33595 Answers: 0 Comments: 0

find lim_(x→0) ((ln(1+sinx) −x(√(1−x)))/(sinx −shx)) .

$${find}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:\:\frac{{ln}\left(\mathrm{1}+{sinx}\right)\:−{x}\sqrt{\mathrm{1}−{x}}}{{sinx}\:−{shx}}\:\:. \\ $$

Question Number 33594 Answers: 0 Comments: 1

calculate lim_(x→1^− ) (1/((1−x)^α ))(arcsinx −(π/2)) .

$${calculate}\:\:{lim}_{{x}\rightarrow\mathrm{1}^{−} } \:\:\:\:\:\:\:\:\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)^{\alpha} }\left({arcsinx}\:−\frac{\pi}{\mathrm{2}}\right)\:. \\ $$

Pg 1680 Pg 1681 Pg 1682 Pg 1683 Pg 1684 Pg 1685 Pg 1686 Pg 1687 Pg 1688 Pg 1689

Contact: info@tinkutara.com