Question and Answers Forum

AllQuestion and Answers: Page 1683

Question Number 39023 Answers: 0 Comments: 1

let g(x)= ∫_(−∞) ^(+∞) ((arctan(x(1+t^2 )))/(1+t^2 ))dt with x>0 find a simple form of g(x) .

$${let}\:{g}\left({x}\right)=\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{arctan}\left({x}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:\:{with}\:{x}>\mathrm{0} \\ $$$${find}\:{a}\:{simple}\:{form}\:{of}\:{g}\left({x}\right)\:. \\ $$

Question Number 39022 Answers: 0 Comments: 1

let p(x)= (1+e^(iθ) x)^n −(1−e^(iθ) x)^n with n integr natural 1) find the roots of p(x) 2) fctorize inside C[x] p(x) 3) factorize inside R[x] p(x). θ ∈R

$${let}\:{p}\left({x}\right)=\:\left(\mathrm{1}+{e}^{{i}\theta} {x}\right)^{{n}} \:−\left(\mathrm{1}−{e}^{{i}\theta} {x}\right)^{{n}} \:{with}\:{n}\:{integr}\:{natural} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{the}\:{roots}\:{of}\:{p}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{fctorize}\:{inside}\:{C}\left[{x}\right]\:{p}\left({x}\right) \\ $$$$\left.\mathrm{3}\right)\:{factorize}\:{inside}\:{R}\left[{x}\right]\:{p}\left({x}\right).\:\:\theta\:\in{R} \\ $$

Question Number 39021 Answers: 0 Comments: 0

calculate A_n = ∫_0 ^1 sin(narctanx)dx with n integr natural. 2) find nature of Σ_n A_n

$${calculate}\:\:{A}_{{n}} =\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{sin}\left({narctanx}\right){dx}\:\:{with}\:{n}\:{integr}\:{natural}. \\ $$$$\left.\mathrm{2}\right)\:{find}\:{nature}\:{of}\:\sum_{{n}} \:\:{A}_{{n}} \\ $$

Question Number 39020 Answers: 1 Comments: 0

calculate ∫_0 ^1 ((ln(1+(√(x^2 +1))))/(√(x^2 +1))) dx

$${calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+\sqrt{{x}^{\mathrm{2}} \:+\mathrm{1}}\right)}{\sqrt{{x}^{\mathrm{2}} \:+\mathrm{1}}}\:{dx} \\ $$

Question Number 39019 Answers: 1 Comments: 3

calculate ∫ (dx/((x^2 +1)(x^2 +2)(x^2 +3))) 1) find the value of ∫_0 ^∞ (dx/((x^2 +1)(x^2 +2)(x^2 +3)))

$${calculate}\:\int\:\:\:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{2}\right)\left({x}^{\mathrm{2}} \:+\mathrm{3}\right)} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)\left({x}^{\mathrm{2}} \:+\mathrm{3}\right)} \\ $$

Question Number 39018 Answers: 0 Comments: 0

find nature of Σ_(n=0) ^∞ (((−1)^([x]) )/(2+cos(n[x])))

$${find}\:{nature}\:{of}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\:\:\frac{\left(−\mathrm{1}\right)^{\left[{x}\right]} }{\mathrm{2}+{cos}\left({n}\left[{x}\right]\right)} \\ $$

Question Number 39017 Answers: 0 Comments: 1

find ∫ ((−2x+3)/(x^2 ( x^3 +8)))dx 2) calculate ∫_1 ^(+∞) ((−2x+3)/(x^2 (x^3 +8)))dx

$${find}\:\:\:\int\:\:\frac{−\mathrm{2}{x}+\mathrm{3}}{{x}^{\mathrm{2}} \left(\:{x}^{\mathrm{3}} \:+\mathrm{8}\right)}{dx} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{1}} ^{+\infty} \:\:\:\frac{−\mathrm{2}{x}+\mathrm{3}}{{x}^{\mathrm{2}} \left({x}^{\mathrm{3}} \:+\mathrm{8}\right)}{dx} \\ $$

Question Number 39016 Answers: 0 Comments: 0

calculate ∫_0 ^π ((sin(nx))/(cosx))dx with n from N .

$${calculate}\:\int_{\mathrm{0}} ^{\pi} \:\:\:\:\frac{{sin}\left({nx}\right)}{{cosx}}{dx}\:\:{with}\:{n}\:{from}\:{N}\:. \\ $$

Question Number 39015 Answers: 0 Comments: 2

find ∫ (dx/(x(2x+1)(3x+2))) 2) calculate ∫_1 ^2 (dx/(x(2x+1)(3x+2)))

$${find}\:\:\int\:\:\:\:\:\:\frac{{dx}}{{x}\left(\mathrm{2}{x}+\mathrm{1}\right)\left(\mathrm{3}{x}+\mathrm{2}\right)} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{1}} ^{\mathrm{2}} \:\:\:\:\frac{{dx}}{{x}\left(\mathrm{2}{x}+\mathrm{1}\right)\left(\mathrm{3}{x}+\mathrm{2}\right)} \\ $$

Question Number 39013 Answers: 0 Comments: 1

Given the matrices A = ((3,5),(2,4) ) and I = ((1,0),(0,1) ) find matrix B if BA= I find A′ the reflection on the line y = x and A′′ the enlargement with matrix (((2 0)),((0 2)) ).

$${Given}\:{the}\:{matrices} \\ $$$${A}\:=\:\begin{pmatrix}{\mathrm{3}}&{\mathrm{5}}\\{\mathrm{2}}&{\mathrm{4}}\end{pmatrix}\:{and}\:{I}\:=\:\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}\\{\mathrm{0}}&{\mathrm{1}}\end{pmatrix} \\ $$$${find}\:{matrix}\:{B}\:{if}\: \\ $$$${BA}=\:{I} \\ $$$${find}\:{A}'\:{the}\:{reflection}\:{on}\:{the} \\ $$$${line}\:{y}\:=\:{x}\:{and}\:{A}''\:{the}\:{enlargement} \\ $$$${with}\:{matrix}\:\begin{pmatrix}{\mathrm{2}\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{\mathrm{0}\:\:\:\:\:\:\:\:\:\:\mathrm{2}}\end{pmatrix}. \\ $$

Question Number 39053 Answers: 1 Comments: 0

Question Number 39003 Answers: 2 Comments: 0

Question Number 38982 Answers: 0 Comments: 9

Question Number 38967 Answers: 1 Comments: 8

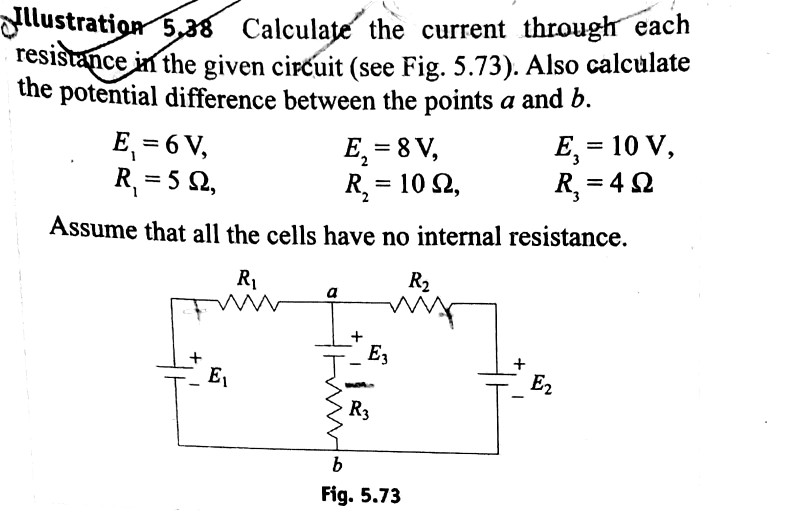
Question Number 38996 Answers: 1 Comments: 1

a line with equation y = 2x + 5.the point (1,7) lie on this line.find the distance between this line and the line joining (2,3) and (6,7).

$${a}\:{line}\:{with}\:{equation} \\ $$$$\:{y}\:=\:\mathrm{2}{x}\:+\:\mathrm{5}.{the}\:{point}\:\left(\mathrm{1},\mathrm{7}\right) \\ $$$${lie}\:{on}\:{this}\:{line}.{find}\:{the}\: \\ $$$${distance}\:{between}\:{this}\:{line}\: \\ $$$${and}\:{the}\:{line}\:{joining}\: \\ $$$$\left(\mathrm{2},\mathrm{3}\right)\:{and}\:\left(\mathrm{6},\mathrm{7}\right). \\ $$

Question Number 38960 Answers: 1 Comments: 0

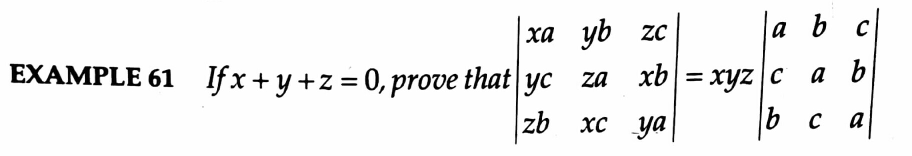
Question Number 38957 Answers: 1 Comments: 1

solve for x e^(2x) + 2e^x + 1 = 0

$${solve}\:{for}\:{x}\: \\ $$$${e}^{\mathrm{2}{x}} \:+\:\mathrm{2}{e}^{{x}} \:+\:\mathrm{1}\:=\:\mathrm{0} \\ $$

Question Number 38946 Answers: 0 Comments: 0

find ∫ arcos(2(√(1−x^2 )))dx .

$${find}\:\int\:{arcos}\left(\mathrm{2}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\right){dx}\:. \\ $$

Question Number 38942 Answers: 0 Comments: 1

find Σ_(n=1) ^∞ (((−1)^n )/n)cos(nx) and Σ_(n=1) ^∞ (((−1)^n )/n)sin(nx)

$${find}\:\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}{cos}\left({nx}\right)\:{and}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}}{sin}\left({nx}\right) \\ $$

Question Number 38939 Answers: 2 Comments: 2

Question Number 38936 Answers: 1 Comments: 1

Question Number 38933 Answers: 0 Comments: 9

[Refreshing an old question] Find the smallest number which is a multiple of 75 and has 75 divisors.

$$\left[{Refreshing}\:{an}\:{old}\:{question}\right] \\ $$$${Find}\:{the}\:{smallest}\:{number}\:{which}\:{is} \\ $$$${a}\:{multiple}\:{of}\:\mathrm{75}\:{and}\:{has}\:\mathrm{75}\:{divisors}. \\ $$

Question Number 38923 Answers: 1 Comments: 0

A glass prism made from a material of refractive index 1.55 has a refracting angle of 60°.The prism is immersed in water of refractive index 1.33.Determine the angle of minimum deviation for a parallel beam of light passing through the prism.

$${A}\:{glass}\:{prism}\:{made}\:{from}\:{a}\:{material} \\ $$$${of}\:{refractive}\:{index}\:\mathrm{1}.\mathrm{55}\:{has}\:{a} \\ $$$${refracting}\:{angle}\:{of}\:\mathrm{60}°.{The}\:{prism} \\ $$$${is}\:{immersed}\:{in}\:{water}\:{of}\:{refractive} \\ $$$${index}\:\mathrm{1}.\mathrm{33}.{Determine}\:{the}\:{angle}\:{of} \\ $$$${minimum}\:{deviation}\:{for}\:{a}\:{parallel} \\ $$$${beam}\:{of}\:{light}\:{passing}\:{through}\:{the} \\ $$$${prism}. \\ $$

Question Number 38925 Answers: 1 Comments: 0

Calculate the deviation of a ray of light,which is incident on a plane mirror at an angle of 40°.

$${Calculate}\:{the}\:{deviation}\:{of}\:{a}\:{ray}\:{of} \\ $$$${light},{which}\:{is}\:{incident}\:{on}\:{a}\:{plane} \\ $$$${mirror}\:{at}\:{an}\:{angle}\:{of}\:\mathrm{40}°. \\ $$

Question Number 38907 Answers: 1 Comments: 5

(√(c^2 +x^2 )) = 1+(c/x) how many complex roots ?

$$\sqrt{{c}^{\mathrm{2}} +{x}^{\mathrm{2}} }\:=\:\mathrm{1}+\frac{{c}}{{x}} \\ $$$${how}\:{many}\:{complex}\:{roots}\:? \\ $$

Question Number 38905 Answers: 1 Comments: 0

Pg 1678 Pg 1679 Pg 1680 Pg 1681 Pg 1682 Pg 1683 Pg 1684 Pg 1685 Pg 1686 Pg 1687

Contact: info@tinkutara.com