
AllQuestion and Answers: Page 1682
Question Number 39142 Answers: 1 Comments: 0
Question Number 39135 Answers: 1 Comments: 2
Question Number 39127 Answers: 0 Comments: 1
Question Number 39144 Answers: 1 Comments: 0
Question Number 39121 Answers: 0 Comments: 5
Question Number 39120 Answers: 1 Comments: 1
Question Number 39119 Answers: 0 Comments: 1
Question Number 39104 Answers: 1 Comments: 1

Question Number 39089 Answers: 2 Comments: 1
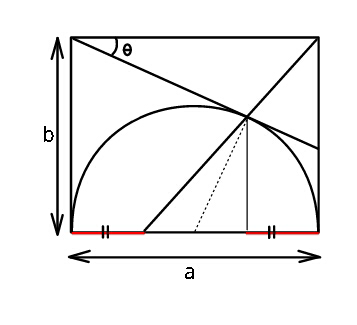
Question Number 39078 Answers: 1 Comments: 2
Question Number 39072 Answers: 2 Comments: 1

Question Number 39067 Answers: 2 Comments: 7

Question Number 39059 Answers: 1 Comments: 0
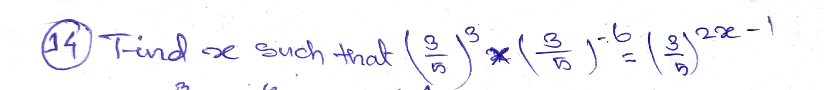
Question Number 39058 Answers: 1 Comments: 1
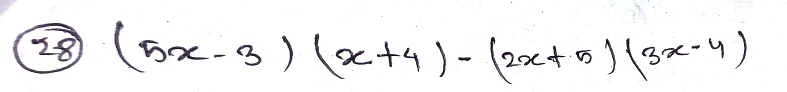
Question Number 39055 Answers: 1 Comments: 0

Question Number 39040 Answers: 0 Comments: 0
Question Number 39039 Answers: 0 Comments: 2
Question Number 39038 Answers: 0 Comments: 2
Question Number 39037 Answers: 0 Comments: 2
Question Number 39035 Answers: 0 Comments: 1
Question Number 39034 Answers: 0 Comments: 1
Question Number 39033 Answers: 0 Comments: 2
Question Number 39032 Answers: 1 Comments: 0
$${x}+{y}=\mathrm{3} \\ $$$${x}=\mathrm{2} \\ $$$${y}=? \\ $$
Question Number 39028 Answers: 1 Comments: 0
Question Number 39026 Answers: 2 Comments: 0
Question Number 39025 Answers: 0 Comments: 1
Pg 1677 Pg 1678 Pg 1679 Pg 1680 Pg 1681 Pg 1682 Pg 1683 Pg 1684 Pg 1685 Pg 1686
