Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1681

Question Number 39475 Answers: 1 Comments: 0

Question Number 39470 Answers: 2 Comments: 2

Question Number 39469 Answers: 1 Comments: 0

Question Number 39466 Answers: 0 Comments: 1

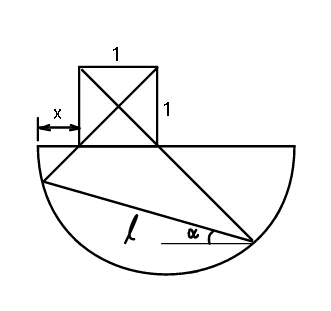
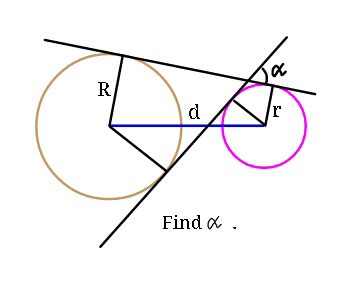
Question Number 39464 Answers: 1 Comments: 0

Domain of the explicit form of the function y represented implicitly by the equation (1+x)cosy−x^2 =0 is (a) (−1,1] (b) (−1, 1−(√)5/2] (c) [1−(√)5/2, 1+(√)5/2] (d) [0, 1+(√)5/2]

$${Domain}\:\:{of}\:\:{the}\:\:{explicit}\:\:{form}\:\:{of} \\ $$$${the}\:\:{function}\:\:\:{y}\:\:\:{represented}\: \\ $$$${implicitly}\:\:\:{by}\:\:{the}\:\:{equation}\: \\ $$$$\left(\mathrm{1}+{x}\right){cosy}−{x}^{\mathrm{2}} =\mathrm{0}\:\:{is} \\ $$$$\left({a}\right)\:\:\left(−\mathrm{1},\mathrm{1}\right]\:\:\:\:\:\:\:\:\:\:\left({b}\right)\:\:\:\:\left(−\mathrm{1},\:\mathrm{1}−\sqrt{}\mathrm{5}/\mathrm{2}\right] \\ $$$$\left({c}\right)\:\:\:\left[\mathrm{1}−\sqrt{}\mathrm{5}/\mathrm{2},\:\mathrm{1}+\sqrt{}\mathrm{5}/\mathrm{2}\right] \\ $$$$\left({d}\right)\:\:\left[\mathrm{0},\:\mathrm{1}+\sqrt{}\mathrm{5}/\mathrm{2}\right] \\ $$

Question Number 39458 Answers: 0 Comments: 4

find the greatest possible square insribed in a triangle with sides a b c

$$\mathrm{find}\:\mathrm{the}\:\mathrm{greatest}\:\mathrm{possible}\:\mathrm{square}\:\mathrm{insribed}\:\mathrm{in} \\ $$$$\mathrm{a}\:\mathrm{triangle}\:\mathrm{with}\:\mathrm{sides}\:{a}\:{b}\:{c} \\ $$

Question Number 39457 Answers: 1 Comments: 0

Question Number 39443 Answers: 1 Comments: 3

lim_(n→∞) [ (1/(n^2 +1))+ (2/(n^2 +2))+ (3/(n^2 +3))+ ....+(1/(n+1))] = ?

$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\:\left[\:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} +\mathrm{1}}+\:\frac{\mathrm{2}}{\mathrm{n}^{\mathrm{2}} +\mathrm{2}}+\:\frac{\mathrm{3}}{\mathrm{n}^{\mathrm{2}} +\mathrm{3}}+\:....+\frac{\mathrm{1}}{\mathrm{n}+\mathrm{1}}\right]\:=\:? \\ $$

Question Number 39441 Answers: 0 Comments: 2

∫_(1/4) ^( 4) (1/x) sin (x−(1/x))dx = ?

$$\int_{\frac{\mathrm{1}}{\mathrm{4}}} ^{\:\mathrm{4}} \:\frac{\mathrm{1}}{{x}}\:\mathrm{sin}\:\left({x}−\frac{\mathrm{1}}{{x}}\right){dx}\:=\:? \\ $$

Question Number 39440 Answers: 1 Comments: 0

f(x)= ∫_0 ^( x_ ) e^(t ) (((1+sin t)/(1+cos t))) dt. Then f((π/3))×f(((2π)/3)) = ?

$$\mathrm{f}\left({x}\right)=\:\int_{\mathrm{0}} ^{\:{x}_{} } \:{e}^{{t}\:} \left(\frac{\mathrm{1}+\mathrm{sin}\:{t}}{\mathrm{1}+\mathrm{cos}\:{t}}\right)\:{dt}. \\ $$$${T}\mathrm{hen}\:\:\mathrm{f}\left(\frac{\pi}{\mathrm{3}}\right)×\mathrm{f}\left(\frac{\mathrm{2}\pi}{\mathrm{3}}\right)\:=\:? \\ $$

Question Number 39436 Answers: 1 Comments: 2

Question Number 39477 Answers: 1 Comments: 3

∫2^x 3^(2x) dx=?

$$\int\mathrm{2}^{\mathrm{x}} \mathrm{3}^{\mathrm{2x}} \mathrm{dx}=? \\ $$

Question Number 39431 Answers: 1 Comments: 1

∫_0 ^(2π) e^(x/2) sin ((x/2)+(π/4))dx = ?

$$\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\mathrm{e}^{\frac{{x}}{\mathrm{2}}} \mathrm{sin}\:\left(\frac{{x}}{\mathrm{2}}+\frac{\pi}{\mathrm{4}}\right)\mathrm{d}{x}\:=\:? \\ $$

Question Number 39402 Answers: 1 Comments: 1

Question Number 39483 Answers: 0 Comments: 3

find f(t)= ∫_0 ^1 ((ln(1+xt))/(1+x^2 )) dx .

$${find}\:{f}\left({t}\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{xt}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx}\:. \\ $$

Question Number 39395 Answers: 0 Comments: 2

Given that θ is an obtuse angle find tan θ if cos θ =(3/5)

$${Given}\:{that}\:\theta\:{is}\:{an}\:{obtuse}\: \\ $$$${angle}\:{find}\:{tan}\:\theta\:{if} \\ $$$${cos}\:\theta\:=\frac{\mathrm{3}}{\mathrm{5}} \\ $$$$ \\ $$

Question Number 39389 Answers: 0 Comments: 2

calculate F(x) = ∫_0 ^∞ (dt/(1+(1+x(1+t^2 ))^2 ))

$${calculate}\:{F}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\:\frac{{dt}}{\mathrm{1}+\left(\mathrm{1}+{x}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\right)^{\mathrm{2}} } \\ $$

Question Number 39388 Answers: 1 Comments: 0

calculate A =tan((π/5)).tan(((2π)/5)).tan(((3π)/5)).tan(((4π)/5))

$${calculate}\:{A}\:={tan}\left(\frac{\pi}{\mathrm{5}}\right).{tan}\left(\frac{\mathrm{2}\pi}{\mathrm{5}}\right).{tan}\left(\frac{\mathrm{3}\pi}{\mathrm{5}}\right).{tan}\left(\frac{\mathrm{4}\pi}{\mathrm{5}}\right) \\ $$

Question Number 39386 Answers: 1 Comments: 1

find the value of ∫_0 ^1 ((ln(1+x))/(1+x^2 ))dx

$${find}\:{the}\:{value}\:{of}\:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$

Question Number 39384 Answers: 2 Comments: 0

The values of a for which y= ax^2 +ax+(1/(24)) and x = ay^2 +ay+(1/(24)) touch each other are 1) (2/3) 2) (3/2) 3) ((13+(√(601)))/(12)) 4) ((13−(√(601)))/(12)).

$$\mathrm{The}\:\mathrm{values}\:\mathrm{of}\:\mathrm{a}\:\mathrm{for}\:\mathrm{which}\:\mathrm{y}=\:\mathrm{a}{x}^{\mathrm{2}} +{ax}+\frac{\mathrm{1}}{\mathrm{24}} \\ $$$${and}\:{x}\:=\:{ay}^{\mathrm{2}} +{ay}+\frac{\mathrm{1}}{\mathrm{24}}\:{touch}\:{each}\:{other} \\ $$$${are} \\ $$$$\left.\mathrm{1}\left.\right)\:\frac{\mathrm{2}}{\mathrm{3}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\right)\:\frac{\mathrm{3}}{\mathrm{2}} \\ $$$$\left.\mathrm{3}\left.\right)\:\frac{\mathrm{13}+\sqrt{\mathrm{601}}}{\mathrm{12}}\:\:\:\:\:\:\:\mathrm{4}\right)\:\frac{\mathrm{13}−\sqrt{\mathrm{601}}}{\mathrm{12}}. \\ $$

Question Number 39383 Answers: 1 Comments: 1

calculate ∫_0 ^(π/3) ((sinxdx)/(cosx(2+ln(cosx))) .

$${calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\:\:\:\frac{{sinxdx}}{{cosx}\left(\mathrm{2}+{ln}\left({cosx}\right)\right.}\:. \\ $$

Question Number 39382 Answers: 1 Comments: 0

Question Number 39381 Answers: 1 Comments: 0

Question Number 39380 Answers: 0 Comments: 0

find the value of Σ_(n=0) ^∞ (((−1)^n )/(4n+1))

$${find}\:{the}\:{value}\:{of}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{4}{n}+\mathrm{1}} \\ $$

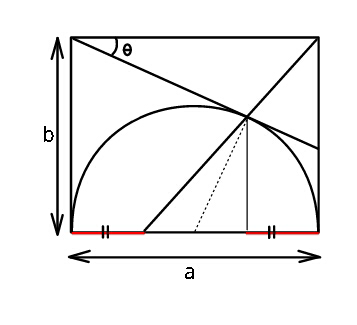
Question Number 39379 Answers: 1 Comments: 6

Question Number 39378 Answers: 0 Comments: 1

study the derivability of f(x)=Σ_(n=0) ^∞ (((−1)^n )/(nx +1))

$${study}\:{the}\:{derivability}\:{of} \\ $$$${f}\left({x}\right)=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{{nx}\:+\mathrm{1}} \\ $$

Pg 1676 Pg 1677 Pg 1678 Pg 1679 Pg 1680 Pg 1681 Pg 1682 Pg 1683 Pg 1684 Pg 1685

Contact: info@tinkutara.com