
AllQuestion and Answers: Page 1678
Question Number 39607 Answers: 3 Comments: 0
Question Number 39591 Answers: 1 Comments: 0
Question Number 39588 Answers: 1 Comments: 0
Question Number 39587 Answers: 4 Comments: 0
Question Number 39586 Answers: 2 Comments: 0
Question Number 39582 Answers: 1 Comments: 0
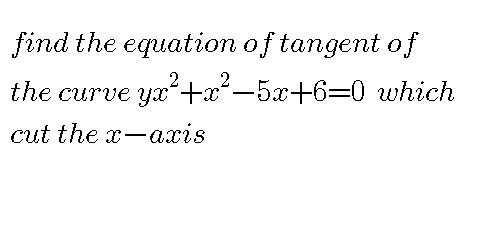
Question Number 39573 Answers: 1 Comments: 1
Question Number 39559 Answers: 2 Comments: 1

Question Number 39529 Answers: 0 Comments: 5

Question Number 39520 Answers: 1 Comments: 1
Question Number 39519 Answers: 1 Comments: 1
Question Number 39517 Answers: 0 Comments: 2
Question Number 39524 Answers: 0 Comments: 0

Question Number 39511 Answers: 0 Comments: 2

Question Number 39508 Answers: 1 Comments: 1
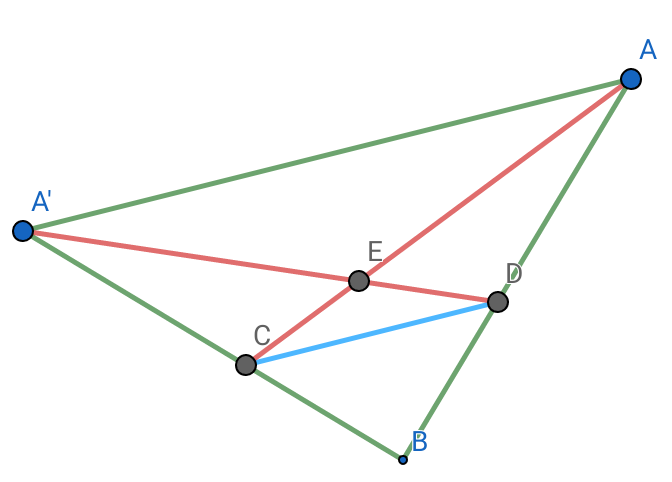
Question Number 39486 Answers: 0 Comments: 7
Question Number 39475 Answers: 1 Comments: 0

Question Number 39470 Answers: 2 Comments: 2

Question Number 39469 Answers: 1 Comments: 0
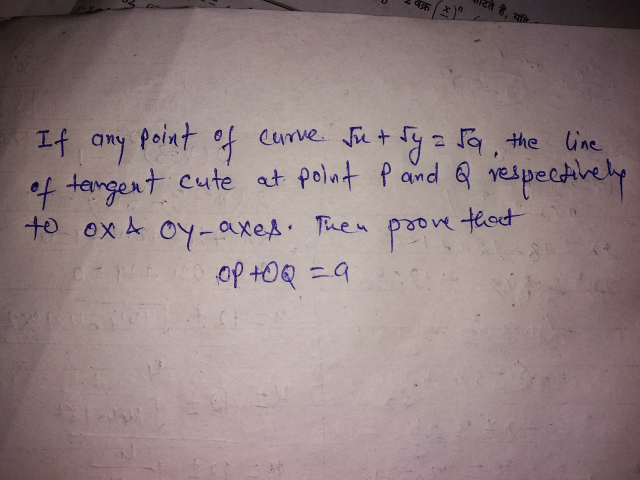
Question Number 39466 Answers: 0 Comments: 1

Question Number 39464 Answers: 1 Comments: 0
Question Number 39458 Answers: 0 Comments: 4
Question Number 39457 Answers: 1 Comments: 0
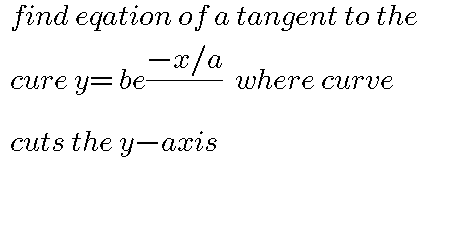
Question Number 39443 Answers: 1 Comments: 3
Question Number 39441 Answers: 0 Comments: 2
Question Number 39440 Answers: 1 Comments: 0
Pg 1673 Pg 1674 Pg 1675 Pg 1676 Pg 1677 Pg 1678 Pg 1679 Pg 1680 Pg 1681 Pg 1682
