
AllQuestion and Answers: Page 1677
Question Number 39695 Answers: 0 Comments: 4
Question Number 39689 Answers: 1 Comments: 2
Question Number 39949 Answers: 2 Comments: 1
Question Number 39678 Answers: 1 Comments: 1
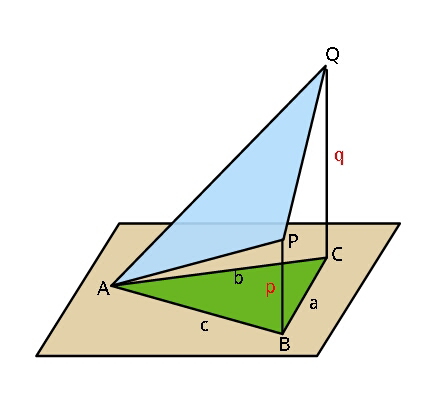
Question Number 39671 Answers: 1 Comments: 0
Question Number 39666 Answers: 1 Comments: 1

Question Number 39669 Answers: 1 Comments: 0

Question Number 39660 Answers: 0 Comments: 2
Question Number 39655 Answers: 1 Comments: 1

Question Number 39727 Answers: 0 Comments: 1
Question Number 39651 Answers: 1 Comments: 0
Question Number 39650 Answers: 1 Comments: 0
Question Number 39649 Answers: 1 Comments: 1
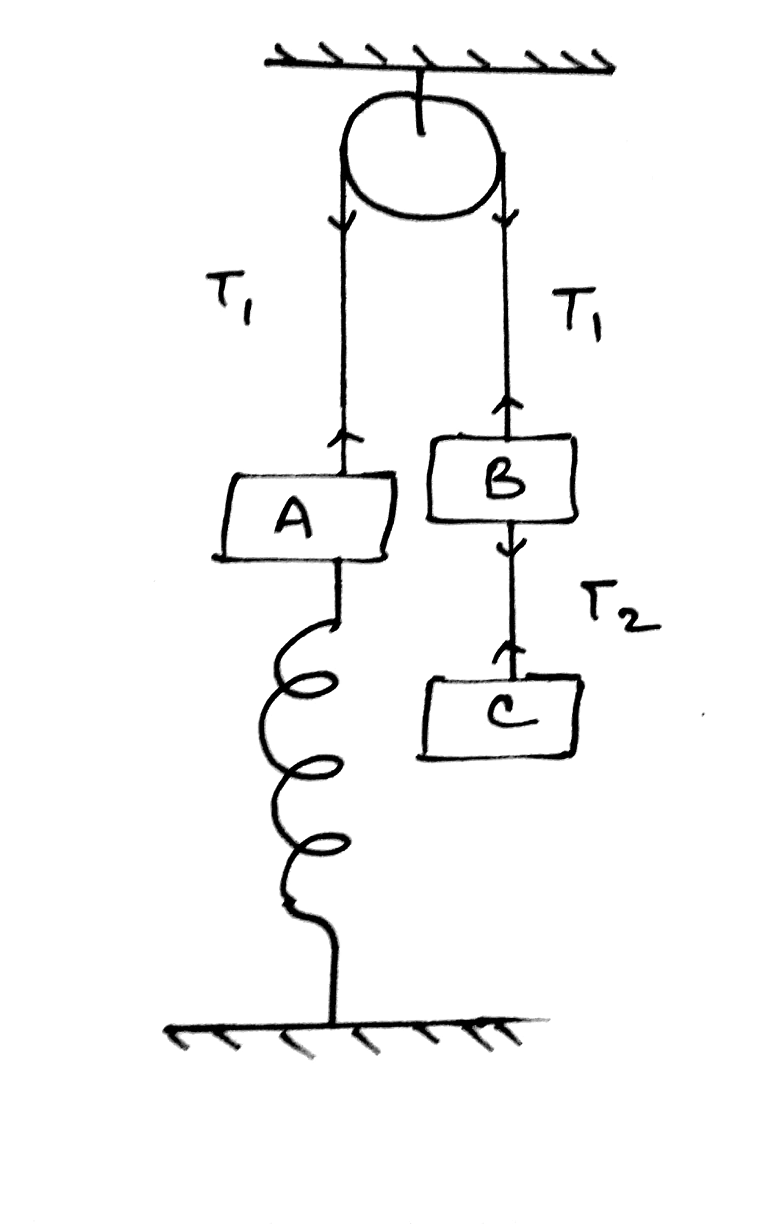
Question Number 39640 Answers: 1 Comments: 0
Question Number 39639 Answers: 1 Comments: 0

Question Number 39638 Answers: 1 Comments: 0

Question Number 39636 Answers: 1 Comments: 0
Question Number 39635 Answers: 1 Comments: 1
Question Number 39633 Answers: 0 Comments: 3
Question Number 39632 Answers: 0 Comments: 0
Question Number 39631 Answers: 0 Comments: 0
Question Number 39626 Answers: 2 Comments: 1
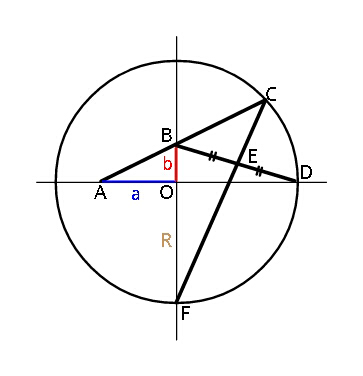
Question Number 39623 Answers: 0 Comments: 0
Question Number 39622 Answers: 0 Comments: 0
Question Number 39612 Answers: 1 Comments: 4

Question Number 39611 Answers: 1 Comments: 0
Pg 1672 Pg 1673 Pg 1674 Pg 1675 Pg 1676 Pg 1677 Pg 1678 Pg 1679 Pg 1680 Pg 1681
