
AllQuestion and Answers: Page 1676
Question Number 39712 Answers: 1 Comments: 3
Question Number 39711 Answers: 0 Comments: 1
Question Number 39709 Answers: 1 Comments: 1

Question Number 39708 Answers: 1 Comments: 0
Question Number 39706 Answers: 0 Comments: 0

Question Number 39703 Answers: 0 Comments: 1
Question Number 39702 Answers: 0 Comments: 6
Question Number 39700 Answers: 0 Comments: 1
Question Number 39699 Answers: 0 Comments: 5
Question Number 39696 Answers: 1 Comments: 3

Question Number 39695 Answers: 0 Comments: 4
Question Number 39689 Answers: 1 Comments: 2
Question Number 39949 Answers: 2 Comments: 1
Question Number 39678 Answers: 1 Comments: 1
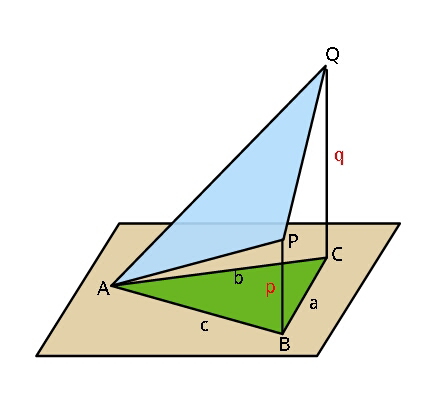
Question Number 39671 Answers: 1 Comments: 0
Question Number 39666 Answers: 1 Comments: 1

Question Number 39669 Answers: 1 Comments: 0

Question Number 39660 Answers: 0 Comments: 2
Question Number 39655 Answers: 1 Comments: 1

Question Number 39727 Answers: 0 Comments: 1
Question Number 39651 Answers: 1 Comments: 0
Question Number 39650 Answers: 1 Comments: 0
Question Number 39649 Answers: 1 Comments: 1
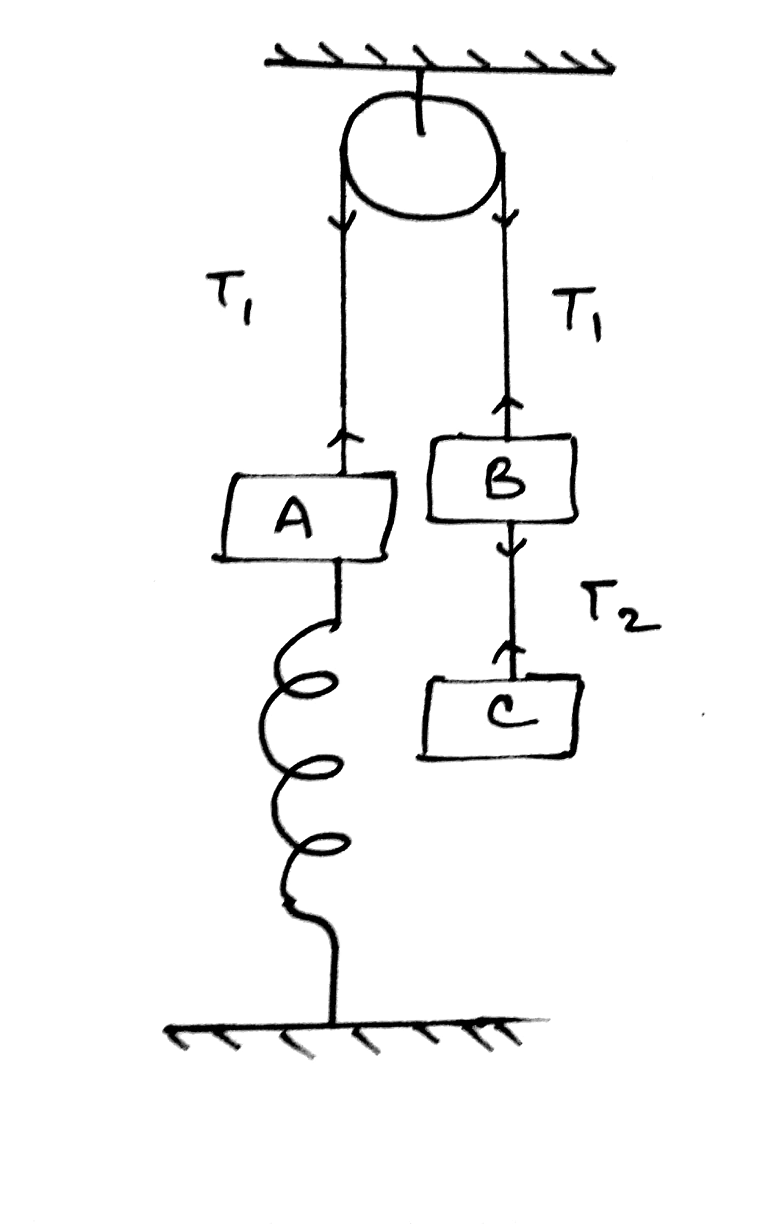
Question Number 39640 Answers: 1 Comments: 0
Question Number 39639 Answers: 1 Comments: 0

Question Number 39638 Answers: 1 Comments: 0

Pg 1671 Pg 1672 Pg 1673 Pg 1674 Pg 1675 Pg 1676 Pg 1677 Pg 1678 Pg 1679 Pg 1680
