Question and Answers Forum

AllQuestion and Answers: Page 1675

Question Number 39921 Answers: 4 Comments: 0

Question Number 39930 Answers: 1 Comments: 0

Question Number 39899 Answers: 0 Comments: 0

Given the lines l_1 ; 3y = 2x ,l_2 ; y = −((3x)/2) + p and l_3 ; y ^ = x + 1 a) find the value of p if the point of intersection between l_1 and l_2 is (3,5) b) find the cosine of the angle between l_2 and l_3 c) which line holds the point (1,2). d)find the line l_4 with gradient ∫_4 ^π [l_1 + l_2 dx] perpendicur to l_2 ,parrallel to l_1 .

$${Given}\:{the}\:{lines}\: \\ $$$${l}_{\mathrm{1}} ;\:\mathrm{3}{y}\:=\:\mathrm{2}{x}\:,{l}_{\mathrm{2}} ;\:{y}\:=\:−\frac{\mathrm{3}{x}}{\mathrm{2}}\:+\:{p} \\ $$$${and}\:{l}_{\mathrm{3}} ;\:{y}\overset{} {\:}=\:{x}\:+\:\mathrm{1} \\ $$$$\left.{a}\right)\:{find}\:{the}\:{value}\:{of}\:{p}\:{if}\: \\ $$$${the}\:{point}\:{of}\:{intersection}\:{between} \\ $$$${l}_{\mathrm{1}} \:{and}\:{l}_{\mathrm{2}} \:{is}\:\left(\mathrm{3},\mathrm{5}\right) \\ $$$$\left.{b}\right)\:{find}\:{the}\:{cosine}\:{of}\:{the}\:{angle} \\ $$$${between}\:{l}_{\mathrm{2}} \:{and}\:{l}_{\mathrm{3}} \\ $$$$\left.{c}\right)\:{which}\:{line}\:{holds}\:{the}\:{point} \\ $$$$\left(\mathrm{1},\mathrm{2}\right). \\ $$$$\left.{d}\right){find}\:{the}\:{line}\:{l}_{\mathrm{4}} \:{with}\:{gradient} \\ $$$$\int_{\mathrm{4}} ^{\pi} \left[{l}_{\mathrm{1}} \:+\:{l}_{\mathrm{2}} \:{dx}\right]\:{perpendicur}\:{to} \\ $$$${l}_{\mathrm{2}} ,{parrallel}\:{to}\:{l}_{\mathrm{1}} . \\ $$

Question Number 39914 Answers: 0 Comments: 1

Question Number 39892 Answers: 0 Comments: 5

let g(x)= e^(−2x) arctan(x+3) developp g at integr serie .

$${let}\:{g}\left({x}\right)=\:{e}^{−\mathrm{2}{x}} \:{arctan}\left({x}+\mathrm{3}\right) \\ $$$${developp}\:{g}\:{at}\:{integr}\:{serie}\:\:. \\ $$

Question Number 39891 Answers: 0 Comments: 5

let f(x)=arctan(2x+1) 1) calculate f^((n)) (x) 2) calculate f^((n)) (0) 3) developp f at integr serie 4) calculate ∫_0 ^1 f(x)dx 5) calculate ∫_0 ^1 ((arctan(2x+1))/(4x^2 +4x +2))dx

$${let}\:{f}\left({x}\right)={arctan}\left(\mathrm{2}{x}+\mathrm{1}\right) \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\:{f}^{\left({n}\right)} \left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:{f}^{\left({n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{3}\right)\:{developp}\:{f}\:{at}\:{integr}\:{serie} \\ $$$$\left.\mathrm{4}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:{f}\left({x}\right){dx} \\ $$$$\left.\mathrm{5}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{arctan}\left(\mathrm{2}{x}+\mathrm{1}\right)}{\mathrm{4}{x}^{\mathrm{2}} \:+\mathrm{4}{x}\:+\mathrm{2}}{dx} \\ $$

Question Number 39879 Answers: 2 Comments: 0

let p(x)= x^3 + 3x − 4 find α+β and αβ

$${let}\: \\ $$$${p}\left({x}\right)=\:{x}^{\mathrm{3}} \:+\:\mathrm{3}{x}\:−\:\mathrm{4} \\ $$$${find}\:\alpha+\beta\:{and}\: \\ $$$$\alpha\beta \\ $$$$ \\ $$$$ \\ $$

Question Number 39868 Answers: 2 Comments: 0

a,b,c>0,if ((8a^2 )/(a^2 +9))=b, ((10b^2 )/(b^2 +16))=c, ((6c^2 )/(c^2 +25))=a, then,what is the minimum value of a+b+c?

$${a},{b},{c}>\mathrm{0},{if} \\ $$$$\frac{\mathrm{8}{a}^{\mathrm{2}} }{{a}^{\mathrm{2}} +\mathrm{9}}={b},\:\:\frac{\mathrm{10}{b}^{\mathrm{2}} }{{b}^{\mathrm{2}} +\mathrm{16}}={c},\:\:\frac{\mathrm{6}{c}^{\mathrm{2}} }{{c}^{\mathrm{2}} +\mathrm{25}}={a}, \\ $$$${then},{what}\:{is}\:{the}\:{minimum}\:{value}\:{of}\:\:{a}+{b}+{c}? \\ $$

Question Number 39860 Answers: 1 Comments: 1

Question Number 39854 Answers: 2 Comments: 2

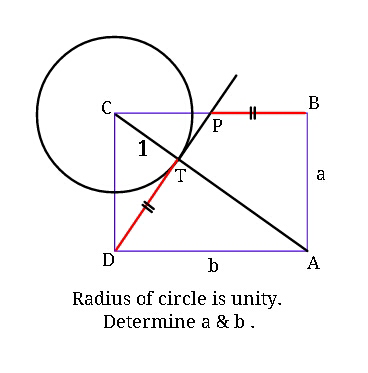
Question Number 39851 Answers: 2 Comments: 1

Question Number 39848 Answers: 2 Comments: 0

Question Number 39846 Answers: 2 Comments: 2

the sum of the four digit even numbers that can be formed with digits 0 3 5 4 without repitation

$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{four}\:\:\mathrm{digit}\:\mathrm{even}\:\mathrm{numbers}\:\mathrm{that}\:\mathrm{can}\:\mathrm{be}\:\mathrm{formed}\:\mathrm{with}\:\mathrm{digits}\:\mathrm{0}\:\mathrm{3}\:\mathrm{5}\:\mathrm{4}\:\mathrm{without}\:\mathrm{repitation} \\ $$

Question Number 39840 Answers: 0 Comments: 1

calculate lim_(x→0) ∫_(x+1) ^(x^2 +1) ln(1+t) e^(−t) dt

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:\:\:\int_{{x}+\mathrm{1}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \:\:{ln}\left(\mathrm{1}+{t}\right)\:{e}^{−{t}} {dt}\: \\ $$

Question Number 39839 Answers: 0 Comments: 1

calculate lim_(x→1) ∫_x ^x^2 ((arctan(2t))/(sin(πt)))dt

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{1}} \:\:\:\int_{{x}} ^{{x}^{\mathrm{2}} } \:\:\:\frac{{arctan}\left(\mathrm{2}{t}\right)}{{sin}\left(\pi{t}\right)}{dt} \\ $$

Question Number 39838 Answers: 0 Comments: 1

find lim_(ξ→0) ∫_0 ^1 (dx/((√(1+ξx^2 ))−(√(1−ξx^2 ))))

$${find}\:{lim}_{\xi\rightarrow\mathrm{0}} \:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{dx}}{\sqrt{\mathrm{1}+\xi{x}^{\mathrm{2}} }−\sqrt{\mathrm{1}−\xi{x}^{\mathrm{2}} }}\: \\ $$

Question Number 39837 Answers: 0 Comments: 0

let S_n =Σ_(n=1) ^∞ e^(−n[(x/n)]) find a equivalent of S_n when n→+∞

$${let}\:{S}_{{n}} =\sum_{{n}=\mathrm{1}} ^{\infty} \:\:{e}^{−{n}\left[\frac{{x}}{{n}}\right]} \\ $$$${find}\:{a}\:{equivalent}\:{of}\:{S}_{{n}} \:{when}\:{n}\rightarrow+\infty \\ $$

Question Number 39836 Answers: 0 Comments: 0

calculate ∫_0 ^(+∞) ((ln(1+ix^2 ))/(2+x^2 ))dx

$${calculate}\:\:\int_{\mathrm{0}} ^{+\infty} \frac{{ln}\left(\mathrm{1}+{ix}^{\mathrm{2}} \right)}{\mathrm{2}+{x}^{\mathrm{2}} }{dx} \\ $$

Question Number 39835 Answers: 0 Comments: 0

simplify [(([nx])/n)] with n natural integr not0 and x real

$${simplify}\:\left[\frac{\left[{nx}\right]}{{n}}\right]\:{with}\:{n}\:{natural}\:{integr}\:{not}\mathrm{0}\:\:{and}\:{x}\:{real} \\ $$

Question Number 39834 Answers: 1 Comments: 0

calculate ∫_0 ^(π/6) ∣ cos(2x)−cos(3x)∣dx

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\mid\:{cos}\left(\mathrm{2}{x}\right)−{cos}\left(\mathrm{3}{x}\right)\mid{dx} \\ $$

Question Number 39833 Answers: 2 Comments: 1

find ∫ ((ln(x+(√(x^2 −1))))/(√(x^2 −1))) dx 2) calculate ∫_2 ^5 ((ln(x+(√(x^2 −1)))/(√(x^2 −1)))dx

$${find}\:\:\int\:\:\:\:\frac{{ln}\left({x}+\sqrt{{x}^{\mathrm{2}} \:−\mathrm{1}}\right)}{\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}\:{dx} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{2}} ^{\mathrm{5}} \:\:\:\frac{{ln}\left({x}+\sqrt{{x}^{\mathrm{2}} \:−\mathrm{1}}\right.}{\sqrt{{x}^{\mathrm{2}} −\mathrm{1}}}{dx} \\ $$

Question Number 39827 Answers: 2 Comments: 0

if f(x) = 3x^3 + px^2 + 4x − 8 and (x − 1) is a factor of f(x). a) find the value of p. with these value of p b) solve the equation f(x) = 0. if α and β are roots of f(x), c) find α + β and αβ d) Evaluate α^2 + β^2 hence α − β

$${if}\:{f}\left({x}\right)\:=\:\mathrm{3}{x}^{\mathrm{3}} \:+\:{px}^{\mathrm{2}} \:+\:\mathrm{4}{x}\:−\:\mathrm{8} \\ $$$${and}\:\left({x}\:−\:\mathrm{1}\right)\:{is}\:{a}\:{factor}\:{of}\: \\ $$$${f}\left({x}\right). \\ $$$$\left.{a}\right)\:{find}\:{the}\:{value}\:{of}\:{p}. \\ $$$${with}\:{these}\:{value}\:{of}\:{p} \\ $$$$\left.{b}\right)\:{solve}\:{the}\:{equation}\:{f}\left({x}\right)\:=\:\mathrm{0}. \\ $$$${if}\:\alpha\:{and}\:\beta\:{are}\:{roots}\:{of}\: \\ $$$${f}\left({x}\right),\: \\ $$$$\left.{c}\right)\:{find}\:\alpha\:+\:\beta\:{and}\:\alpha\beta \\ $$$$\left.{d}\right)\:{Evaluate}\:\alpha^{\mathrm{2}} \:+\:\beta^{\mathrm{2}} \:{hence}\:\alpha\:−\:\beta \\ $$$$ \\ $$

Question Number 40139 Answers: 0 Comments: 3

let I_n = ∫_0 ^1 x^n (√(1−x)) dx 1) calculate I_0 and I_1 2) prove that ∀n∈ N^★ (3+2n) I_n =2n I_(n−1) 3) find I_n interms of n

$${let}\:\:{I}_{{n}} =\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:{x}^{{n}} \sqrt{\mathrm{1}−{x}}\:{dx} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{I}_{\mathrm{0}} \:\:{and}\:{I}_{\mathrm{1}} \\ $$$$\left.\mathrm{2}\right)\:{prove}\:{that}\:\:\forall{n}\in\:{N}^{\bigstar} \:\:\:\:\left(\mathrm{3}+\mathrm{2}{n}\right)\:{I}_{{n}} =\mathrm{2}{n}\:{I}_{{n}−\mathrm{1}} \\ $$$$\left.\mathrm{3}\right)\:{find}\:{I}_{{n}} \:\:{interms}\:{of}\:{n} \\ $$

Question Number 39814 Answers: 2 Comments: 0

kwame, ama and yaw shared an amount of money in the ratio 3:4:5. if ama had $8,400 more than kwame. find the total amount shared.

$$\mathrm{kwame},\:\mathrm{ama}\:\mathrm{and}\:\mathrm{yaw}\:\mathrm{shared}\:\mathrm{an}\:\mathrm{amount}\:\mathrm{of}\:\mathrm{money} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{ratio}\:\mathrm{3}:\mathrm{4}:\mathrm{5}.\:\mathrm{if}\:\mathrm{ama}\:\mathrm{had}\:\$\mathrm{8},\mathrm{400}\:\mathrm{more}\:\mathrm{than}\:\mathrm{kwame}. \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{total}\:\mathrm{amount}\:\mathrm{shared}. \\ $$

Question Number 39811 Answers: 1 Comments: 1

Question Number 39799 Answers: 1 Comments: 0

what will be the number which makes 680621 a perfect square root?

$$\boldsymbol{\mathrm{what}}\:\boldsymbol{\mathrm{will}}\:\boldsymbol{\mathrm{be}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{number}}\:\boldsymbol{\mathrm{which}}\:\boldsymbol{\mathrm{makes}}\:\mathrm{680621} \\ $$$$\boldsymbol{\mathrm{a}}\:\boldsymbol{\mathrm{perfect}}\:\boldsymbol{\mathrm{square}}\:\boldsymbol{\mathrm{root}}? \\ $$

Pg 1670 Pg 1671 Pg 1672 Pg 1673 Pg 1674 Pg 1675 Pg 1676 Pg 1677 Pg 1678 Pg 1679

Contact: info@tinkutara.com