Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1671

Question Number 40745 Answers: 3 Comments: 0

Question Number 40743 Answers: 2 Comments: 0

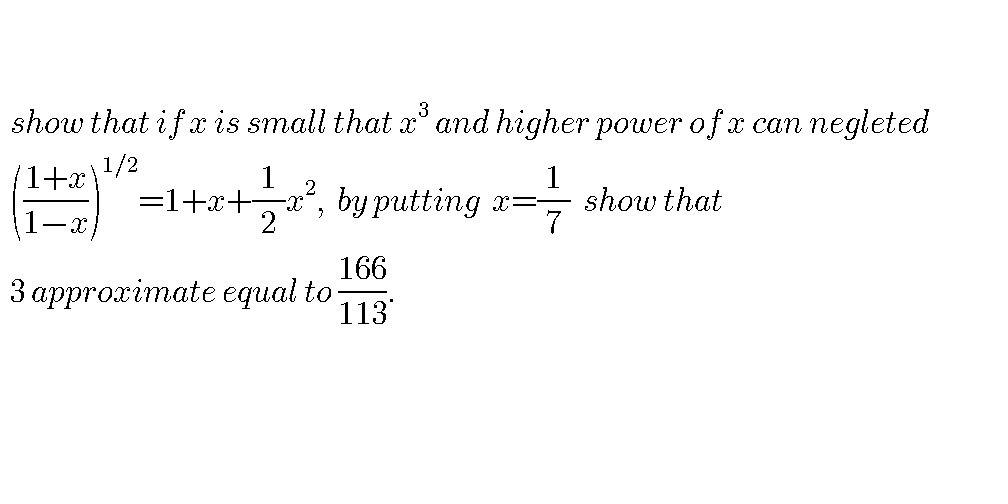
Question Number 40740 Answers: 0 Comments: 5

Question Number 40738 Answers: 2 Comments: 2

Question Number 40732 Answers: 1 Comments: 2

Question Number 40717 Answers: 1 Comments: 1

∫(√(tanx/sinx.cosxdx))

$$\int\sqrt{{tanx}/{sinx}.{cosxdx}} \\ $$

Question Number 40716 Answers: 2 Comments: 0

∫(cosx−cos2x/1−cosx)dx

$$\int\left({cosx}−{cos}\mathrm{2}{x}/\mathrm{1}−{cosx}\right){dx} \\ $$

Question Number 40715 Answers: 1 Comments: 1

for x≥2 ∣x−2∣=

$$\mathrm{for}\:\mathrm{x}\geqslant\mathrm{2}\:\mid\mathrm{x}−\mathrm{2}\mid= \\ $$

Question Number 40711 Answers: 1 Comments: 0

Two point charges,q_1 =0.4μC and q_2 =−0.3μC are placed at 10cm apart.Calculate (a)the potential at point A which is midway between them,and (b)point B which is 6cm from q_1 and 8cm from q_2

$${Two}\:{point}\:{charges},{q}_{\mathrm{1}} =\mathrm{0}.\mathrm{4}\mu{C}\:{and} \\ $$$${q}_{\mathrm{2}} =−\mathrm{0}.\mathrm{3}\mu{C}\:{are}\:{placed}\:{at}\:\mathrm{10}{cm} \\ $$$${apart}.{Calculate}\: \\ $$$$\left({a}\right){the}\:{potential}\:{at}\:{point}\:{A}\:{which}\:{is} \\ $$$${midway}\:{between}\:{them},{and} \\ $$$$\left({b}\right){point}\:{B}\:{which}\:{is}\:\mathrm{6}{cm}\:{from}\:{q}_{\mathrm{1}} \\ $$$${and}\:\mathrm{8}{cm}\:{from}\:{q}_{\mathrm{2}} \\ $$

Question Number 40709 Answers: 1 Comments: 1

calculate lim_(x→0) ((cos(x−sinx)−1)/(x^2 ))

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:\:\frac{{cos}\left({x}−{sinx}\right)−\mathrm{1}}{{x}^{\mathrm{2}} \:} \\ $$

Question Number 40700 Answers: 1 Comments: 0

A charge q_1 =2.0×10^(−9) C is placed at the point,(x=0,y=4cm) and another charge,q_2 =−3.0×10^(−9) C is located at the point,(x=3cm,y=4cm). If a third charge,q_3 =4.0×10^(−9) C is placed at the origin, a)obtain the x and y component of the total force on q_3 b)Calculate the magnitude and direction of the total force on q_(3.)

$${A}\:{charge}\:{q}_{\mathrm{1}} =\mathrm{2}.\mathrm{0}×\mathrm{10}^{−\mathrm{9}} {C}\:{is}\:{placed} \\ $$$${at}\:{the}\:{point},\left({x}=\mathrm{0},{y}=\mathrm{4}{cm}\right)\:{and} \\ $$$${another}\:{charge},{q}_{\mathrm{2}} =−\mathrm{3}.\mathrm{0}×\mathrm{10}^{−\mathrm{9}} {C} \\ $$$${is}\:{located}\:{at}\:{the}\:{point},\left({x}=\mathrm{3}{cm},{y}=\mathrm{4}{cm}\right). \\ $$$${If}\:{a}\:{third}\:{charge},{q}_{\mathrm{3}} =\mathrm{4}.\mathrm{0}×\mathrm{10}^{−\mathrm{9}} {C} \\ $$$${is}\:{placed}\:{at}\:{the}\:{origin}, \\ $$$$\left.{a}\right){obtain}\:{the}\:{x}\:{and}\:{y}\:{component}\:{of} \\ $$$${the}\:{total}\:{force}\:{on}\:{q}_{\mathrm{3}} \\ $$$$\left.{b}\right){Calculate}\:{the}\:{magnitude}\:{and} \\ $$$${direction}\:{of}\:{the}\:{total}\:{force}\:{on}\:{q}_{\mathrm{3}.} \\ $$

Question Number 40699 Answers: 1 Comments: 0

Two point charges q_1 =1.5×10^(−9) C and q_2 =3.0×10^(−9) C are seperated by a distance of 200cm.Calculate the point at which the total electric field is zero.

$${Two}\:{point}\:{charges}\:{q}_{\mathrm{1}} =\mathrm{1}.\mathrm{5}×\mathrm{10}^{−\mathrm{9}} {C} \\ $$$${and}\:{q}_{\mathrm{2}} =\mathrm{3}.\mathrm{0}×\mathrm{10}^{−\mathrm{9}} {C}\:{are}\:{seperated} \\ $$$${by}\:{a}\:{distance}\:{of}\:\mathrm{200}{cm}.{Calculate} \\ $$$${the}\:{point}\:{at}\:{which}\:{the}\:{total}\:{electric} \\ $$$${field}\:{is}\:{zero}. \\ $$

Question Number 40686 Answers: 0 Comments: 6

Question Number 40684 Answers: 0 Comments: 1

∫((x^7 −1)/(logx))dx

$$\int\frac{\mathrm{x}^{\mathrm{7}} −\mathrm{1}}{\mathrm{logx}}\mathrm{dx} \\ $$

Question Number 40675 Answers: 1 Comments: 2

Question Number 40667 Answers: 1 Comments: 2

find the value lim_(x→0) g(x) must have, if g complies the statement about limit. Suppose lim_(x→ −4) [x lim_(x→0) g(x)] = 2

$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{g}\left(\mathrm{x}\right)\:\:\mathrm{must}\:\mathrm{have},\:\mathrm{if}\:\mathrm{g}\:\mathrm{complies}\:\mathrm{the}\:\mathrm{statement}\: \\ $$$$\mathrm{about}\:\mathrm{limit}.\:\mathrm{Suppose}\:\:\:\underset{{x}\rightarrow\:−\mathrm{4}} {\mathrm{lim}}\:\:\left[\mathrm{x}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\:\mathrm{g}\left(\mathrm{x}\right)\right]\:\:=\:\:\mathrm{2} \\ $$

Question Number 40665 Answers: 1 Comments: 0

−5−( )=3 −5−x=3 x=3+5 x=8(give sign of greater number) −5−8=3

$$−\mathrm{5}−\left(\:\:\right)=\mathrm{3} \\ $$$$−\mathrm{5}−{x}=\mathrm{3} \\ $$$${x}=\mathrm{3}+\mathrm{5} \\ $$$${x}=\mathrm{8}\left({give}\:{sign}\:{of}\:{greater}\:{number}\right) \\ $$$$−\mathrm{5}−\mathrm{8}=\mathrm{3} \\ $$

Question Number 40664 Answers: 1 Comments: 0

Factorise: x^(19) − x^(17) + x^(10) + x^8 + 1

$$\mathrm{Factorise}:\:\:\:\:\:\mathrm{x}^{\mathrm{19}} \:−\:\mathrm{x}^{\mathrm{17}} \:+\:\mathrm{x}^{\mathrm{10}} \:+\:\mathrm{x}^{\mathrm{8}} \:+\:\mathrm{1} \\ $$

Question Number 40662 Answers: 1 Comments: 5

Question Number 40661 Answers: 0 Comments: 4

1)find g(x)=∫_0 ^(π/2) ln(1−x^2 cos^2 θ)dθ with x from R 2) find the value of ∫_0 ^(π/2) ln(1−2 cos^2 θ)dθ and 3) find the value of A(α)=∫_0 ^(π/2) ln(1−cos^2 α cos^2 θ)dθ

$$\left.\mathrm{1}\right){find}\:\:{g}\left({x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{1}−{x}^{\mathrm{2}} {cos}^{\mathrm{2}} \theta\right){d}\theta\:\:{with}\:{x}\:{from}\:{R} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{1}−\mathrm{2}\:{cos}^{\mathrm{2}} \theta\right){d}\theta\:{and} \\ $$$$\left.\mathrm{3}\right)\:{find}\:{the}\:{value}\:{of}\:\: \\ $$$${A}\left(\alpha\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\mathrm{1}−{cos}^{\mathrm{2}} \alpha\:{cos}^{\mathrm{2}} \theta\right){d}\theta\: \\ $$

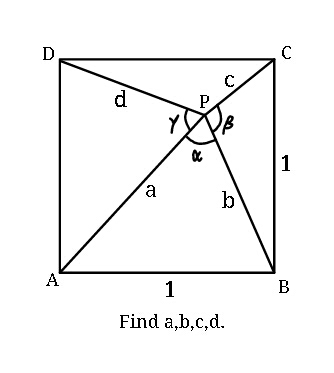
Question Number 40660 Answers: 0 Comments: 0

let f(t) = ∫_0 ^∞ ((arctan(tcosx))/(1+x^2 ))dx 1) find another form of f(t) 2) calculate ∫_0 ^∞ ((arctan(2cosx))/(1+x^2 ))dx .

$${let}\:{f}\left({t}\right)\:=\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left({tcosx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{another}\:{form}\:{of}\:{f}\left({t}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\:\frac{{arctan}\left(\mathrm{2}{cosx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:. \\ $$

Question Number 40658 Answers: 0 Comments: 1

find ∫_0 ^(π/4) ((x−1)/(2+cosx))dx .

$${find}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{{x}−\mathrm{1}}{\mathrm{2}+{cosx}}{dx}\:. \\ $$

Question Number 40657 Answers: 1 Comments: 0