
AllQuestion and Answers: Page 1665
Question Number 40878 Answers: 0 Comments: 0
Question Number 40876 Answers: 0 Comments: 0
Question Number 40875 Answers: 1 Comments: 0
Question Number 40874 Answers: 0 Comments: 0

Question Number 40873 Answers: 1 Comments: 0
Question Number 40872 Answers: 1 Comments: 2
Question Number 40870 Answers: 1 Comments: 1
Question Number 40868 Answers: 0 Comments: 4
$${calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{x}}{{sinx}}{dx}\:\:. \\ $$
Question Number 40867 Answers: 0 Comments: 1
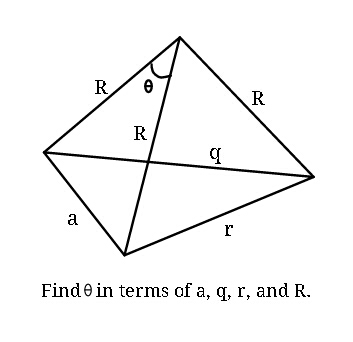
Question Number 40857 Answers: 1 Comments: 2

Question Number 40847 Answers: 1 Comments: 0
Question Number 40830 Answers: 0 Comments: 1
$${find}\:\int\:\sqrt{\mathrm{2}+{tan}^{\mathrm{2}} {t}}{dt} \\ $$
Question Number 40829 Answers: 1 Comments: 0
Question Number 40826 Answers: 1 Comments: 0
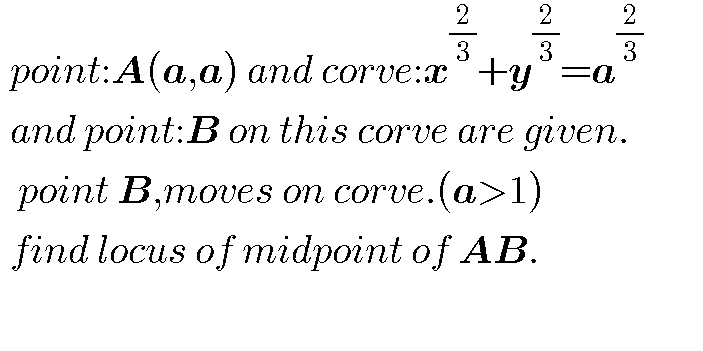
Question Number 40823 Answers: 0 Comments: 1
Question Number 40822 Answers: 2 Comments: 0

Question Number 40818 Answers: 1 Comments: 0
Question Number 40817 Answers: 1 Comments: 0
Question Number 40816 Answers: 0 Comments: 0
Question Number 40815 Answers: 1 Comments: 0
Question Number 40814 Answers: 0 Comments: 3

Question Number 40813 Answers: 0 Comments: 4

Question Number 40804 Answers: 0 Comments: 6

Question Number 40787 Answers: 1 Comments: 4
Question Number 40786 Answers: 1 Comments: 0
Question Number 40782 Answers: 0 Comments: 0
Pg 1660 Pg 1661 Pg 1662 Pg 1663 Pg 1664 Pg 1665 Pg 1666 Pg 1667 Pg 1668 Pg 1669
