
AllQuestion and Answers: Page 1664
Question Number 40984 Answers: 0 Comments: 1
Question Number 40975 Answers: 2 Comments: 0

Question Number 40974 Answers: 0 Comments: 0
Question Number 40965 Answers: 1 Comments: 0
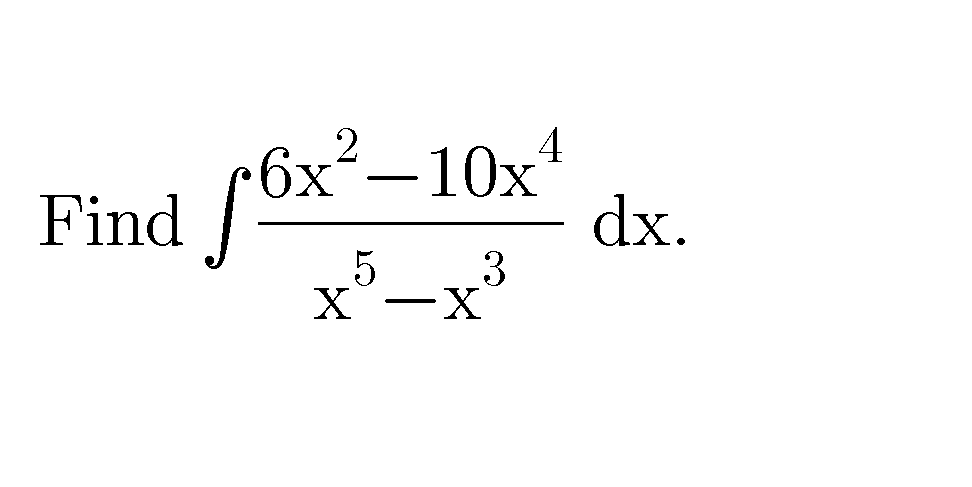
Question Number 40961 Answers: 1 Comments: 0

Question Number 40960 Answers: 0 Comments: 1

Question Number 40952 Answers: 0 Comments: 1

Question Number 40948 Answers: 2 Comments: 1

Question Number 40939 Answers: 0 Comments: 1

Question Number 40930 Answers: 0 Comments: 1

Question Number 40928 Answers: 1 Comments: 0

Question Number 40920 Answers: 1 Comments: 0
Question Number 40918 Answers: 1 Comments: 0

Question Number 40917 Answers: 1 Comments: 0

Question Number 40911 Answers: 0 Comments: 7
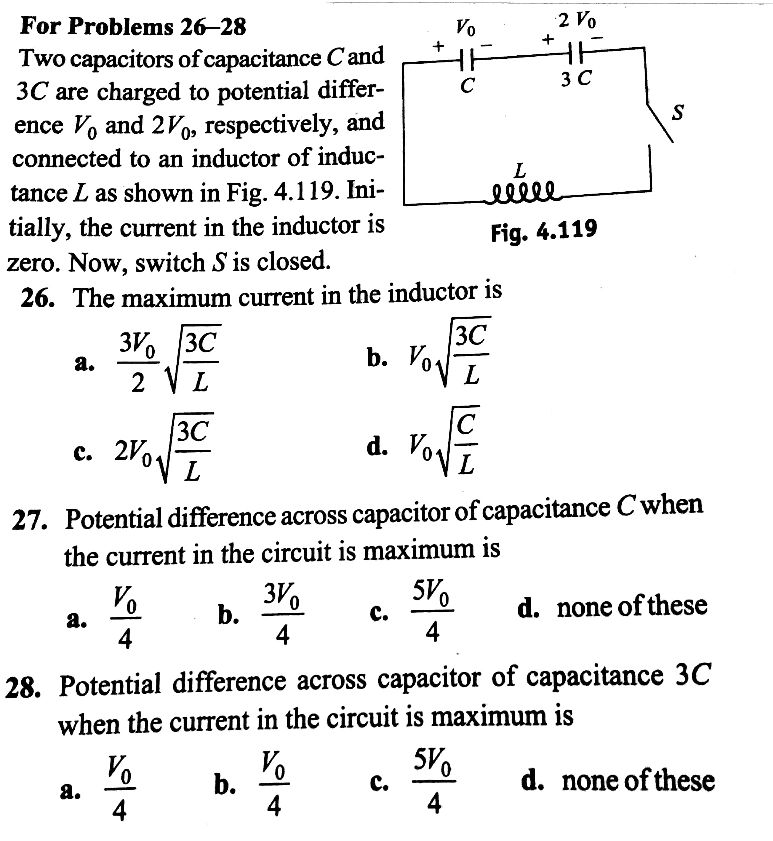
Question Number 40910 Answers: 1 Comments: 0

Question Number 40906 Answers: 2 Comments: 1
Question Number 40898 Answers: 2 Comments: 1
Question Number 40897 Answers: 0 Comments: 1
Question Number 40896 Answers: 0 Comments: 0
Question Number 40895 Answers: 0 Comments: 0
Question Number 40893 Answers: 0 Comments: 0
Question Number 40892 Answers: 0 Comments: 0
Question Number 40891 Answers: 0 Comments: 1
Question Number 40890 Answers: 0 Comments: 2
Question Number 40889 Answers: 1 Comments: 0
Pg 1659 Pg 1660 Pg 1661 Pg 1662 Pg 1663 Pg 1664 Pg 1665 Pg 1666 Pg 1667 Pg 1668
