
AllQuestion and Answers: Page 1663
Question Number 40657 Answers: 1 Comments: 0

Question Number 40656 Answers: 1 Comments: 0
Question Number 40640 Answers: 2 Comments: 0
Question Number 41351 Answers: 1 Comments: 3
Question Number 40637 Answers: 2 Comments: 0
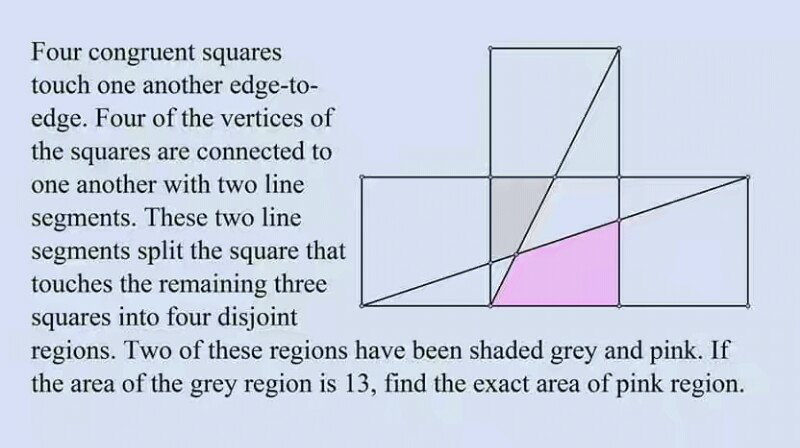
Question Number 40625 Answers: 2 Comments: 0

Question Number 40624 Answers: 0 Comments: 0
Question Number 40621 Answers: 2 Comments: 0
Question Number 40620 Answers: 3 Comments: 0
Question Number 40619 Answers: 0 Comments: 2
Question Number 40615 Answers: 1 Comments: 2
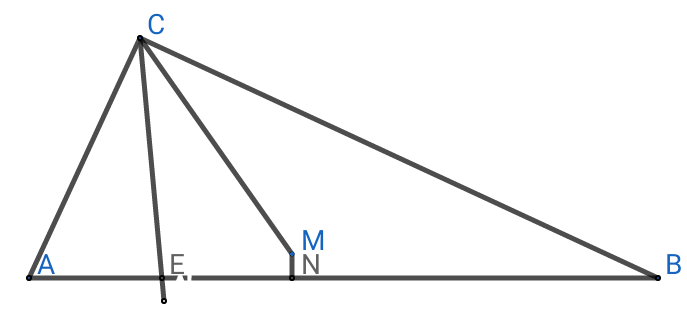
Question Number 40610 Answers: 1 Comments: 0

Question Number 40594 Answers: 1 Comments: 1

Question Number 40587 Answers: 0 Comments: 1
Question Number 40583 Answers: 2 Comments: 0
Question Number 40581 Answers: 1 Comments: 2

Question Number 40580 Answers: 0 Comments: 3
Question Number 40591 Answers: 1 Comments: 1

Question Number 40569 Answers: 0 Comments: 0

Question Number 40551 Answers: 1 Comments: 5

Question Number 40550 Answers: 1 Comments: 0
Question Number 40523 Answers: 1 Comments: 1
Question Number 40544 Answers: 1 Comments: 0
Question Number 40519 Answers: 1 Comments: 1

Question Number 40499 Answers: 1 Comments: 0
Question Number 40498 Answers: 1 Comments: 0
Pg 1658 Pg 1659 Pg 1660 Pg 1661 Pg 1662 Pg 1663 Pg 1664 Pg 1665 Pg 1666 Pg 1667
