
AllQuestion and Answers: Page 1661
Question Number 39868 Answers: 2 Comments: 0
Question Number 39860 Answers: 1 Comments: 1

Question Number 39854 Answers: 2 Comments: 2
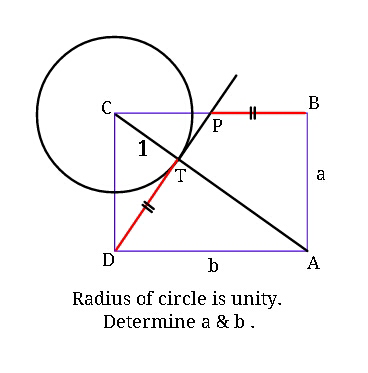
Question Number 39851 Answers: 2 Comments: 1

Question Number 39848 Answers: 2 Comments: 0

Question Number 39846 Answers: 2 Comments: 2
Question Number 39840 Answers: 0 Comments: 1
Question Number 39839 Answers: 0 Comments: 1
Question Number 39838 Answers: 0 Comments: 1
Question Number 39837 Answers: 0 Comments: 0
Question Number 39836 Answers: 0 Comments: 0
Question Number 39835 Answers: 0 Comments: 0
Question Number 39834 Answers: 1 Comments: 0
Question Number 39833 Answers: 2 Comments: 1
Question Number 39827 Answers: 2 Comments: 0
Question Number 40139 Answers: 0 Comments: 3
Question Number 39814 Answers: 2 Comments: 0
Question Number 39811 Answers: 1 Comments: 1

Question Number 39799 Answers: 1 Comments: 0
Question Number 39798 Answers: 0 Comments: 5
Question Number 39794 Answers: 1 Comments: 0
Question Number 39792 Answers: 1 Comments: 1
Question Number 39787 Answers: 0 Comments: 2
Question Number 39783 Answers: 1 Comments: 0
Question Number 39779 Answers: 1 Comments: 0
Question Number 39776 Answers: 0 Comments: 3

Pg 1656 Pg 1657 Pg 1658 Pg 1659 Pg 1660 Pg 1661 Pg 1662 Pg 1663 Pg 1664 Pg 1665
