
AllQuestion and Answers: Page 1659
Question Number 41530 Answers: 1 Comments: 0

Question Number 41522 Answers: 2 Comments: 2
Question Number 41521 Answers: 0 Comments: 0
Question Number 41520 Answers: 3 Comments: 0
Question Number 41519 Answers: 2 Comments: 0
Question Number 41518 Answers: 3 Comments: 0
Question Number 41517 Answers: 1 Comments: 1
Question Number 41516 Answers: 1 Comments: 1
Question Number 41515 Answers: 1 Comments: 3
Question Number 41514 Answers: 1 Comments: 0
Question Number 41513 Answers: 2 Comments: 0
Question Number 41512 Answers: 0 Comments: 0
Question Number 41508 Answers: 0 Comments: 0
Question Number 41495 Answers: 2 Comments: 3
Question Number 41488 Answers: 2 Comments: 0

Question Number 41487 Answers: 3 Comments: 4

Question Number 41461 Answers: 1 Comments: 3
Question Number 41459 Answers: 0 Comments: 1

Question Number 41454 Answers: 1 Comments: 1
Question Number 41447 Answers: 1 Comments: 0
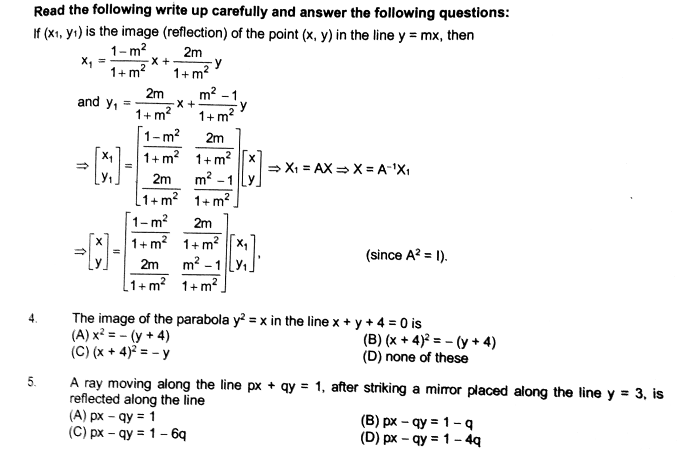
Question Number 41444 Answers: 0 Comments: 1
Question Number 41443 Answers: 1 Comments: 0
Question Number 41479 Answers: 1 Comments: 0

Question Number 41436 Answers: 4 Comments: 0

Question Number 41434 Answers: 1 Comments: 1
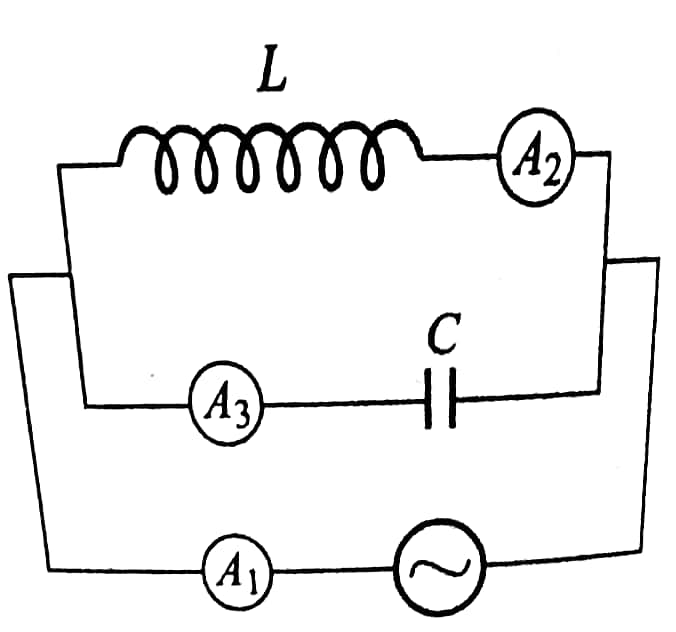
Question Number 41431 Answers: 0 Comments: 3

Pg 1654 Pg 1655 Pg 1656 Pg 1657 Pg 1658 Pg 1659 Pg 1660 Pg 1661 Pg 1662 Pg 1663
