Question and Answers Forum

AllQuestion and Answers: Page 1657

Question Number 41780 Answers: 2 Comments: 0

Question Number 41766 Answers: 2 Comments: 0

Question Number 41765 Answers: 2 Comments: 0

find range of the function f defined by f(x)=(1/(1−x^2 ))

$${find}\:{range}\:{of}\:{the}\:{function}\:{f}\: \\ $$$${defined}\:{by}\:{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}−{x}^{\mathrm{2}} } \\ $$

Question Number 41762 Answers: 0 Comments: 3

let f(x) = ∫_0 ^1 ((ln(1+xt^2 ))/(1+t^2 )) dt 1) find a simple form of f(x) 2) calculate ∫_0 ^1 ((ln(1+t^2 ))/(1+t^2 ))dt 3) calculate ∫_0 ^1 ((ln(1+2t^2 ))/(1+t^2 )) dt

$${let}\:{f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{simple}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt} \\ $$

Question Number 41761 Answers: 1 Comments: 0

calculate lim_(x→0) x ln(1−e^(sinx) )

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:{x}\:{ln}\left(\mathrm{1}−{e}^{{sinx}} \right)\: \\ $$

Question Number 41757 Answers: 0 Comments: 0

Find the fourier sine transform of (1/(x(x^r + a^r )))

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{fourier}\:\mathrm{sine}\:\mathrm{transform}\:\mathrm{of}\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{r}} \:+\:\mathrm{a}^{\mathrm{r}} \right)} \\ $$

Question Number 41744 Answers: 1 Comments: 0

Question Number 41754 Answers: 3 Comments: 0

Question Number 41753 Answers: 2 Comments: 0

How far must a man stand in front of a concave mirror of radius 120cm in order to see an erect image of his face four times its actual size?

$${How}\:{far}\:{must}\:{a}\:{man}\:{stand}\:{in}\:{front} \\ $$$${of}\:{a}\:{concave}\:{mirror}\:{of}\:{radius}\:\mathrm{120}{cm} \\ $$$${in}\:{order}\:{to}\:{see}\:{an}\:{erect}\:{image}\:{of} \\ $$$${his}\:{face}\:{four}\:{times}\:{its}\:{actual}\:{size}? \\ $$

Question Number 41726 Answers: 0 Comments: 0

Tank T_1 and T_2 initially contains 100gal of water each.In T_1 the water is pure,whereas 150lb of fertilizer are dissolved in T_2 .By circulating liquid at a rate of 1gal/min and stirring the amount of fertilizer y_1 (t) in T_1 and y_2 (t) in T_2 change with time t. a)with a schematic diagram write the model linear equations for the mixing problem. (b)determine the eigenvalues and⊛ and eigenvectors of the derived equation.

$${Tank}\:{T}_{\mathrm{1}} \:{and}\:{T}_{\mathrm{2}} \:{initially}\:{contains} \\ $$$$\mathrm{100}{gal}\:{of}\:{water}\:{each}.{In}\:{T}_{\mathrm{1}} \:{the} \\ $$$${water}\:{is}\:{pure},{whereas}\:\mathrm{150}{lb}\:{of} \\ $$$${fertilizer}\:{are}\:{dissolved}\:{in}\:{T}_{\mathrm{2}} .{By} \\ $$$${circulating}\:{liquid}\:{at}\:{a}\:{rate}\:{of} \\ $$$$\mathrm{1}{gal}/{min}\:{and}\:{stirring}\:{the}\:{amount} \\ $$$${of}\:{fertilizer}\:{y}_{\mathrm{1}} \left({t}\right)\:{in}\:{T}_{\mathrm{1}} \:{and}\:{y}_{\mathrm{2}} \left({t}\right) \\ $$$${in}\:{T}_{\mathrm{2}} \:{change}\:{with}\:{time}\:{t}. \\ $$$$\left.{a}\right){with}\:{a}\:{schematic}\:{diagram}\:{write} \\ $$$${the}\:{model}\:{linear}\:{equations}\:{for} \\ $$$${the}\:{mixing}\:{problem}. \\ $$$$\left({b}\right){determine}\:{the}\:{eigenvalues}\:{and}\circledast \\ $$$${and}\:{eigenvectors}\:{of}\:{the}\:{derived} \\ $$$${equation}. \\ $$

Question Number 41722 Answers: 0 Comments: 0

sir Tinkutara is the anyway i could get to talk to you guys(Equation Editor group) i′ve got some ideas i wanna share with you.just comment the messenger media below and the name i′ll get you soon= thanks

$${sir}\:{Tinkutara}\:{is}\:{the}\:{anyway}\:{i}\:{could}\:{get}\:{to}\:{talk}\:{to}\:{you}\:{guys}\left({Equation}\:{Editor}\:{group}\right) \\ $$$${i}'{ve}\:{got}\:{some}\:{ideas}\:{i}\:{wanna}\:{share}\:{with}\:{you}.{just}\:{comment} \\ $$$${the}\:{messenger}\:{media}\:{below}\:{and}\:{the}\:{name}\:{i}'{ll}\:{get}\:{you}\:{soon}= \\ $$$$\:\:{thanks} \\ $$

Question Number 41721 Answers: 0 Comments: 1

show that U_n = 1+ nx + ((n(n−1))/(2!)) x^(2 ) + ((n(n−1)(n−2))/(3!))x^3 + .... for which U_n = (1 + x)^n .

$${show}\:{that} \\ $$$${U}_{{n}} =\:\mathrm{1}+\:{nx}\:+\:\frac{{n}\left({n}−\mathrm{1}\right)}{\mathrm{2}!}\:{x}^{\mathrm{2}\:\:} +\:\frac{{n}\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)}{\mathrm{3}!}{x}^{\mathrm{3}} +\:.... \\ $$$${for}\:{which}\:{U}_{{n}} =\:\left(\mathrm{1}\:+\:{x}\right)^{{n}} . \\ $$

Question Number 41720 Answers: 0 Comments: 2

Difference of last two digits of 2019^(2019^(2019) ) is ?

$$\mathrm{Difference}\:\mathrm{of}\:\mathrm{last}\:\mathrm{two}\:\mathrm{digits}\:\mathrm{of}\:\:\:\:\mathrm{2019}^{\mathrm{2019}^{\mathrm{2019}} } \:\:\:\mathrm{is}\:\:? \\ $$

Question Number 41710 Answers: 2 Comments: 0

Factorize: 60x^3 y + 115x^2 y^2 − 120xy^3

$$\mathrm{Factorize}:\:\:\mathrm{60x}^{\mathrm{3}} \mathrm{y}\:+\:\mathrm{115x}^{\mathrm{2}} \mathrm{y}^{\mathrm{2}} \:−\:\mathrm{120xy}^{\mathrm{3}} \\ $$

Question Number 41707 Answers: 2 Comments: 0

Here′s a question that has troubled me for months now.Please help 5^(√x) − 5^(x−7) =100 find the possible value(s) of x.

$${Here}'{s}\:{a}\:{question}\:{that}\:{has} \\ $$$${troubled}\:{me}\:{for}\:{months}\:{now}.{Please} \\ $$$${help} \\ $$$$ \\ $$$$\mathrm{5}^{\sqrt{{x}}} \:−\:\mathrm{5}^{{x}−\mathrm{7}} \:=\mathrm{100} \\ $$$$ \\ $$$${find}\:{the}\:{possible}\:{value}\left({s}\right)\:{of}\:{x}. \\ $$

Question Number 41706 Answers: 1 Comments: 1

study the convergence of u_n =Σ_(k=2) ^n (1/(kln(k))) −ln(ln(n)

$${study}\:{the}\:{convergence}\:{of}\: \\ $$$${u}_{{n}} =\sum_{{k}=\mathrm{2}} ^{{n}} \:\:\frac{\mathrm{1}}{{kln}\left({k}\right)}\:−{ln}\left({ln}\left({n}\right)\right. \\ $$

Question Number 41705 Answers: 0 Comments: 1

find nsture of the serie Σ u_n with u_n =^n (√(n/(n+1))) −1

$${find}\:{nsture}\:{of}\:{the}\:{serie}\:\Sigma\:{u}_{{n}} \\ $$$${with}\:{u}_{{n}} =^{{n}} \sqrt{\frac{{n}}{{n}+\mathrm{1}}}\:\:\:−\mathrm{1} \\ $$

Question Number 41704 Answers: 0 Comments: 0

let u_n = (1/(√(n−1))) +(2/(√n)) +(1/(√(n+1))) calculate Σ_(n=2) ^∞ u_n

$${let}\:{u}_{{n}} =\:\frac{\mathrm{1}}{\sqrt{{n}−\mathrm{1}}}\:+\frac{\mathrm{2}}{\sqrt{{n}}}\:+\frac{\mathrm{1}}{\sqrt{{n}+\mathrm{1}}} \\ $$$${calculate}\:\sum_{{n}=\mathrm{2}} ^{\infty} \:{u}_{{n}} \\ $$

Question Number 41703 Answers: 0 Comments: 1

calculate I = ∫_0 ^(π/4) ((cosx)/(cos^3 x +sin^3 x))dx

$${calculate}\:\:{I}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\frac{{cosx}}{{cos}^{\mathrm{3}} {x}\:+{sin}^{\mathrm{3}} {x}}{dx} \\ $$

Question Number 41702 Answers: 1 Comments: 3

find the value of ∫_0 ^(√3) arcsin(((2t)/(1+t^2 )))dt

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:{arcsin}\left(\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }\right){dt} \\ $$

Question Number 41699 Answers: 0 Comments: 0

find the perpendicular distance btn two lines y=2x−3 and y=3x−1

$$\boldsymbol{\mathrm{find}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{perpendicular}}\:\boldsymbol{\mathrm{distance}}\:\boldsymbol{\mathrm{btn}} \\ $$$$\boldsymbol{\mathrm{two}}\:\boldsymbol{\mathrm{lines}}\:\boldsymbol{{y}}=\mathrm{2}\boldsymbol{{x}}−\mathrm{3} \\ $$$$\boldsymbol{\mathrm{and}}\:\boldsymbol{{y}}=\mathrm{3}\boldsymbol{{x}}−\mathrm{1} \\ $$

Question Number 44610 Answers: 0 Comments: 1

Let A be 2×3 matrix , whereas B be 3×2 matrix. If determinant(AB)=4, then the value of determinant (BA) ?

$${Let}\:{A}\:{be}\:\mathrm{2}×\mathrm{3}\:{matrix}\:,\:{whereas}\:{B}\:{be} \\ $$$$\mathrm{3}×\mathrm{2}\:{matrix}.\:{If}\:{determinant}\left({AB}\right)=\mathrm{4}, \\ $$$${then}\:{the}\:{value}\:{of}\:{determinant}\:\left({BA}\right)\:? \\ $$

Question Number 41691 Answers: 2 Comments: 0

tan^2 20+tan^2 40+tan^2 80=33

$${tan}^{\mathrm{2}} \mathrm{20}+{tan}^{\mathrm{2}} \mathrm{40}+{tan}^{\mathrm{2}} \mathrm{80}=\mathrm{33} \\ $$

Question Number 41686 Answers: 1 Comments: 2

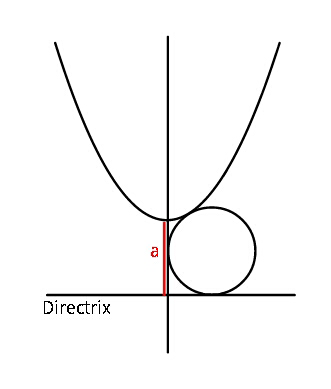
Question Number 41682 Answers: 2 Comments: 0

find radius of curvature to y=sin x at x=π/6 .

$${find}\:{radius}\:{of}\:{curvature}\:{to} \\ $$$${y}=\mathrm{sin}\:{x}\:\:{at}\:\:{x}=\pi/\mathrm{6}\:. \\ $$

Question Number 41679 Answers: 1 Comments: 5

let f(x) = ∫_0 ^1 ln(1+t +xt^2 )dt 1) calculate f^′ (x) then find a simple form of f(x) 2) calculate ∫_0 ^1 ln(1+t +t^2 )dt 3) calculate ∫_0 ^1 ln(1−t^3 )dt .

$${let}\:{f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{t}\:+{xt}^{\mathrm{2}} \right){dt} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{'} \left({x}\right)\:{then}\:{find}\:{a}\:{simple}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{t}\:+{t}^{\mathrm{2}} \right){dt} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:{ln}\left(\mathrm{1}−{t}^{\mathrm{3}} \right){dt}\:. \\ $$

Pg 1652 Pg 1653 Pg 1654 Pg 1655 Pg 1656 Pg 1657 Pg 1658 Pg 1659 Pg 1660 Pg 1661

Contact: info@tinkutara.com