Question and Answers Forum

AllQuestion and Answers: Page 1656

Question Number 42012 Answers: 2 Comments: 0

Solve : (du/dt) = ((3u−7t)/(−7u+3t))

$$\mathrm{Solve}\:: \\ $$$$\frac{{d}\mathrm{u}}{{d}\mathrm{t}}\:=\:\frac{\mathrm{3u}−\mathrm{7t}}{−\mathrm{7u}+\mathrm{3t}} \\ $$

Question Number 42009 Answers: 1 Comments: 0

if (a+bω+cω^2 )+(aω+bω^2 +c)^2 +(aω^2 +b+cω)^2 =0 then prove that a=c or a+c=2b

$$\mathrm{if}\:\left(\mathrm{a}+\mathrm{b}\omega+\mathrm{c}\omega^{\mathrm{2}} \right)+\left(\mathrm{a}\omega+\mathrm{b}\omega^{\mathrm{2}} +\mathrm{c}\right)^{\mathrm{2}} +\left(\mathrm{a}\omega^{\mathrm{2}} +\mathrm{b}+\mathrm{c}\omega\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\mathrm{then}\:\mathrm{prove}\:\mathrm{that}\:\mathrm{a}=\mathrm{c}\:\:\mathrm{or}\:\:\:\mathrm{a}+\mathrm{c}=\mathrm{2b} \\ $$

Question Number 42007 Answers: 2 Comments: 0

Question Number 41998 Answers: 1 Comments: 0

lim_(x→∞) ((x + cos x)/(x + sin x))

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{{x}\:+\:\mathrm{cos}\:{x}}{{x}\:+\:\mathrm{sin}\:{x}} \\ $$

Question Number 41989 Answers: 2 Comments: 1

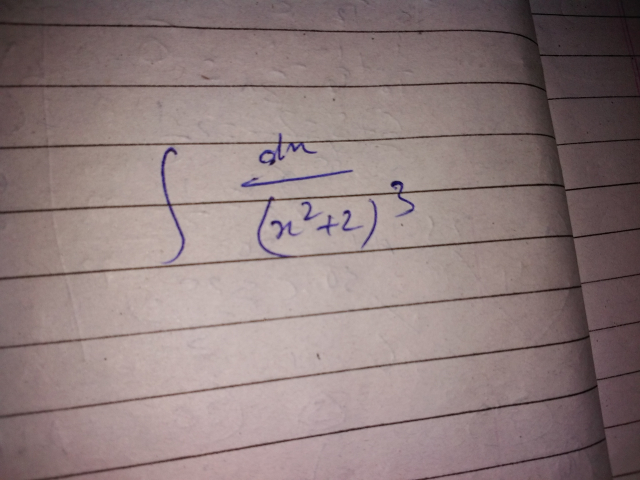
Question Number 41985 Answers: 1 Comments: 1

Prove e^x ≥x+1 ∀x∈R in as many ways as you can show

$$\mathrm{Prove}\:{e}^{{x}} \geqslant{x}+\mathrm{1}\:\forall{x}\in\mathbb{R}\:\mathrm{in}\:\mathrm{as}\:\mathrm{many}\:\mathrm{ways} \\ $$$$\mathrm{as}\:\mathrm{you}\:\mathrm{can}\:\mathrm{show} \\ $$

Question Number 41984 Answers: 2 Comments: 7

solve simultaneously: 2(√k) + h = 9 ....... (i) k + 2(√h) = 3 ....... (ii)

$$\mathrm{solve}\:\mathrm{simultaneously}:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\sqrt{\mathrm{k}}\:\:+\:\mathrm{h}\:=\:\mathrm{9}\:\:\:.......\:\left(\mathrm{i}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{k}\:+\:\mathrm{2}\sqrt{\mathrm{h}}\:\:=\:\mathrm{3}\:\:.......\:\left(\mathrm{ii}\right) \\ $$

Question Number 41966 Answers: 1 Comments: 0

Question Number 41964 Answers: 1 Comments: 10

Prof Kintush has a near point of 45cm and his far point is infinity. What type of lens and focal length should be recommended for his normal reading?

$${Prof}\:{Kintush}\:{has}\:{a}\:{near}\:{point}\:{of} \\ $$$$\mathrm{45}{cm}\:{and}\:{his}\:{far}\:{point}\:{is}\:{infinity}. \\ $$$${What}\:{type}\:{of}\:{lens}\:{and}\:{focal}\:{length} \\ $$$${should}\:{be}\:{recommended}\:{for}\:{his} \\ $$$${normal}\:{reading}? \\ $$

Question Number 41958 Answers: 1 Comments: 0

{ ((x^(√y) +y^(√x) =((49)/(48)))),(((√x)+(√y)=(7/2))) :} find x and y k.k

$$\begin{cases}{\mathrm{x}^{\sqrt{\mathrm{y}}} +\mathrm{y}^{\sqrt{\mathrm{x}}} =\frac{\mathrm{49}}{\mathrm{48}}}\\{\sqrt{\mathrm{x}}+\sqrt{\mathrm{y}}=\frac{\mathrm{7}}{\mathrm{2}}}\end{cases} \\ $$$$\mathrm{find}\:\mathrm{x}\:\mathrm{and}\:\mathrm{y} \\ $$$$\mathrm{k}.\mathrm{k} \\ $$

Question Number 41957 Answers: 1 Comments: 1

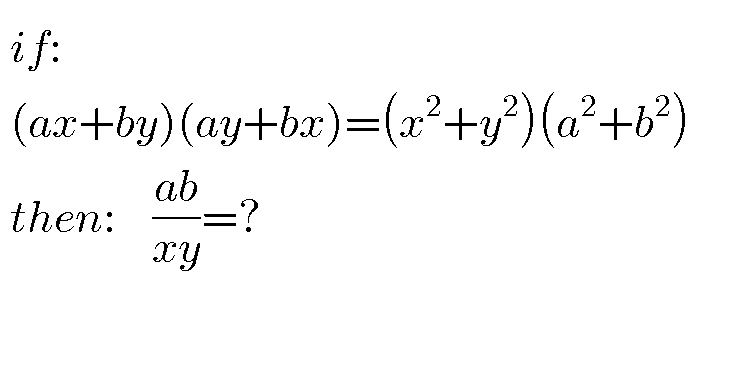
Question Number 41913 Answers: 1 Comments: 0

let f(a) = ∫_0 ^π (x/(1+acosx))dx 1) find f(a) 2) calculate ∫_0 ^π (x/(1+2cosx))dx and ∫_0 ^π (x/(1−2cosx))dx 3) calculate ∫_0 ^π ((xcosx)/((1+acosx)^2 ))dx 4) find the value of ∫_0 ^π ((xcosx)/((1+2cosx)^2 ))dx .

$${let}\:{f}\left({a}\right)\:=\:\int_{\mathrm{0}} ^{\pi} \:\:\:\:\frac{{x}}{\mathrm{1}+{acosx}}{dx} \\ $$$$\left.\mathrm{1}\right)\:{find}\:\:{f}\left({a}\right)\: \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\pi} \:\:\:\frac{{x}}{\mathrm{1}+\mathrm{2}{cosx}}{dx}\:{and}\:\int_{\mathrm{0}} ^{\pi} \:\:\:\frac{{x}}{\mathrm{1}−\mathrm{2}{cosx}}{dx} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\pi} \:\:\frac{{xcosx}}{\left(\mathrm{1}+{acosx}\right)^{\mathrm{2}} }{dx} \\ $$$$\left.\mathrm{4}\right)\:{find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\pi} \:\:\:\frac{{xcosx}}{\left(\mathrm{1}+\mathrm{2}{cosx}\right)^{\mathrm{2}} }{dx}\:. \\ $$

Question Number 41912 Answers: 2 Comments: 0

The estimated time for Abu and Ali to repair a faulty car is six hours. If Abu used 10 hours to repair the car, how many hours will Ali use to repair the car?

$$\mathrm{The}\:\mathrm{estimated}\:\mathrm{time}\:\mathrm{for}\:\mathrm{Abu}\:\mathrm{and}\:\mathrm{Ali}\:\mathrm{to}\:\mathrm{repair}\:\mathrm{a} \\ $$$$\mathrm{faulty}\:\mathrm{car}\:\mathrm{is}\:\mathrm{six}\:\mathrm{hours}.\:\mathrm{If}\:\mathrm{Abu}\:\mathrm{used}\:\mathrm{10}\:\mathrm{hours}\:\mathrm{to} \\ $$$$\mathrm{repair}\:\mathrm{the}\:\mathrm{car},\:\mathrm{how}\:\mathrm{many}\:\mathrm{hours}\:\mathrm{will}\:\mathrm{Ali}\:\mathrm{use}\:\mathrm{to}\:\mathrm{repair} \\ $$$$\mathrm{the}\:\mathrm{car}? \\ $$

Question Number 41911 Answers: 2 Comments: 5

h(x)=(√(sin^4 x+cos^4 x−2msinxcosx)) Find all the values of the parameter m for the funtion denined on R

$${h}\left({x}\right)=\sqrt{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}−\mathrm{2}{msinxcosx}} \\ $$$${Find}\:{all}\:{the}\:{values}\:{of}\:{the}\:{parameter}\:{m}\:{for}\:{the}\:{funtion}\:{denined}\:{on}\:{R} \\ $$$$ \\ $$

Question Number 41906 Answers: 0 Comments: 1

A concave lens of focal length 60cm is made of material whose refractive index for red light is 1.641 and refractive index for blue light is 1.659.That for white light is 1.65.It is combined with a convex lens of dispersive power 0.0173 to form an achromatic doublet. Calculate the focal length of the achromatic lens.

$${A}\:{concave}\:{lens}\:{of}\:{focal}\:{length}\:\mathrm{60}{cm} \\ $$$${is}\:{made}\:{of}\:{material}\:{whose} \\ $$$${refractive}\:{index}\:{for}\:{red}\:{light}\:{is} \\ $$$$\mathrm{1}.\mathrm{641}\:{and}\:{refractive}\:{index}\:{for}\:{blue} \\ $$$${light}\:{is}\:\mathrm{1}.\mathrm{659}.{That}\:{for}\:{white}\:{light} \\ $$$${is}\:\mathrm{1}.\mathrm{65}.{It}\:{is}\:{combined}\:{with}\:{a}\:{convex} \\ $$$${lens}\:{of}\:{dispersive}\:{power}\:\mathrm{0}.\mathrm{0173}\:{to} \\ $$$${form}\:{an}\:{achromatic}\:{doublet}. \\ $$$${Calculate}\:{the}\:{focal}\:{length}\:{of}\:{the} \\ $$$${achromatic}\:{lens}. \\ $$

Question Number 41962 Answers: 1 Comments: 1

A student holding a 324Hz tuning fork approaches a wall at a speed of 6ms^(−1) .The speed of sound in air is 336ms^(−1) .What frequency will the student detect from waves omitted from the fork and waves coming from the wall?

$${A}\:{student}\:{holding}\:{a}\:\mathrm{324}{Hz}\:{tuning} \\ $$$${fork}\:{approaches}\:{a}\:{wall}\:{at}\:{a}\:{speed} \\ $$$${of}\:\mathrm{6}{ms}^{−\mathrm{1}} .{The}\:{speed}\:{of}\:{sound}\:{in}\:{air} \\ $$$${is}\:\mathrm{336}{ms}^{−\mathrm{1}} .{What}\:{frequency}\:{will} \\ $$$${the}\:{student}\:{detect}\:{from}\:{waves} \\ $$$${omitted}\:{from}\:{the}\:{fork}\:{and}\:{waves} \\ $$$${coming}\:{from}\:{the}\:{wall}? \\ $$

Question Number 41902 Answers: 1 Comments: 0

tan3θ tan2θ =1 find the general^(solution......)

$$\mathrm{tan3}\theta\:\mathrm{tan2}\theta\:=\mathrm{1} \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{general}\:^{\mathrm{solution}......} \\ $$

Question Number 41921 Answers: 2 Comments: 0

Find x : 625^(x − 5) = 200((√x))^3

$$\mathrm{Find}\:\mathrm{x}\::\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{625}^{\mathrm{x}\:−\:\mathrm{5}} \:=\:\mathrm{200}\left(\sqrt{\mathrm{x}}\right)^{\mathrm{3}} \\ $$

Question Number 41896 Answers: 2 Comments: 1

∫_(−1/2) ^(1/2) [ (((x+1)/(x−1)))^2 +(((x−1)/(x+1)))^2 −2]^(1/2) dx =

$$\underset{−\mathrm{1}/\mathrm{2}} {\overset{\mathrm{1}/\mathrm{2}} {\int}}\:\:\left[\:\left(\frac{{x}+\mathrm{1}}{{x}−\mathrm{1}}\right)^{\mathrm{2}} +\left(\frac{{x}−\mathrm{1}}{{x}+\mathrm{1}}\right)^{\mathrm{2}} −\mathrm{2}\right]^{\mathrm{1}/\mathrm{2}} {dx}\:= \\ $$

Question Number 41895 Answers: 1 Comments: 1

(√3)sin3x−cos3x+2sin((9x)/4)=4

$$\sqrt{\mathrm{3}}{sin}\mathrm{3}{x}−{cos}\mathrm{3}{x}+\mathrm{2}{sin}\frac{\mathrm{9}{x}}{\mathrm{4}}=\mathrm{4} \\ $$

Question Number 41888 Answers: 0 Comments: 5

The length of mercury column in a mercury-in-glass thermometer is 4cm at triple point.What is the length of the column when the scale indicates temperature of 560K.

$${The}\:{length}\:{of}\:{mercury}\:{column}\:{in} \\ $$$${a}\:{mercury}-{in}-{glass}\:{thermometer} \\ $$$${is}\:\mathrm{4}{cm}\:{at}\:{triple}\:{point}.{What}\:{is}\:{the} \\ $$$${length}\:{of}\:{the}\:{column}\:{when}\:{the} \\ $$$${scale}\:{indicates}\:{temperature}\:{of} \\ $$$$\mathrm{560}{K}. \\ $$

Question Number 41887 Answers: 1 Comments: 0

x+(√y)+xy=82 (√x)+y+(√(xy))=16 Find x and y by khaled.k

$$\mathrm{x}+\sqrt{\mathrm{y}}+\mathrm{xy}=\mathrm{82} \\ $$$$\sqrt{\mathrm{x}}+\mathrm{y}+\sqrt{\mathrm{xy}}=\mathrm{16} \\ $$$$\mathrm{Find}\:\mathrm{x}\:\mathrm{and}\:\mathrm{y} \\ $$$$\mathrm{by}\:\mathrm{khaled}.\mathrm{k} \\ $$

Question Number 41879 Answers: 1 Comments: 0

If a fraction is added to its denominator , it reduces to (1/2) and when the same fraction added to numerator, it also reduces to (2/3) (a) what is the fraction (b) find the square root such that the result of the fraction is less than 1

$$\mathrm{If}\:\mathrm{a}\:\mathrm{fraction}\:\mathrm{is}\:\mathrm{added}\:\mathrm{to}\:\mathrm{its}\:\mathrm{denominator}\:,\:\:\mathrm{it}\:\mathrm{reduces}\:\mathrm{to}\:\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{and}\: \\ $$$$\mathrm{when}\:\mathrm{the}\:\mathrm{same}\:\mathrm{fraction}\:\mathrm{added}\:\mathrm{to}\:\mathrm{numerator},\:\mathrm{it}\:\mathrm{also}\:\mathrm{reduces}\:\mathrm{to}\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{fraction} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{find}\:\mathrm{the}\:\mathrm{square}\:\mathrm{root}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{result}\:\mathrm{of}\:\mathrm{the}\:\mathrm{fraction}\:\mathrm{is}\:\mathrm{less} \\ $$$$\mathrm{than}\:\mathrm{1} \\ $$$$ \\ $$

Question Number 41878 Answers: 1 Comments: 0

(√3)sin3x−cos3x+2sin((9x)/4)=2

$$\sqrt{\mathrm{3}}{sin}\mathrm{3}{x}−{cos}\mathrm{3}{x}+\mathrm{2}{sin}\frac{\mathrm{9}{x}}{\mathrm{4}}=\mathrm{2} \\ $$

Question Number 41877 Answers: 1 Comments: 0

The tens digit of a two digit number is 3 greater than the units digit. When the digits are reversed, the number is reduced by 27. what is the number ?

$$\mathrm{The}\:\mathrm{tens}\:\mathrm{digit}\:\mathrm{of}\:\mathrm{a}\:\mathrm{two}\:\mathrm{digit}\:\mathrm{number}\:\mathrm{is}\:\mathrm{3}\:\mathrm{greater}\:\mathrm{than}\:\mathrm{the}\:\mathrm{units}\:\mathrm{digit}. \\ $$$$\mathrm{When}\:\mathrm{the}\:\mathrm{digits}\:\mathrm{are}\:\mathrm{reversed},\:\mathrm{the}\:\mathrm{number}\:\mathrm{is}\:\mathrm{reduced}\:\mathrm{by}\:\mathrm{27}. \\ $$$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{number}\:? \\ $$

Question Number 41870 Answers: 0 Comments: 0

Pg 1651 Pg 1652 Pg 1653 Pg 1654 Pg 1655 Pg 1656 Pg 1657 Pg 1658 Pg 1659 Pg 1660

Contact: info@tinkutara.com