
AllQuestion and Answers: Page 1636
Question Number 43625 Answers: 1 Comments: 0
Question Number 43624 Answers: 1 Comments: 1
Question Number 43623 Answers: 1 Comments: 3
Question Number 43621 Answers: 1 Comments: 2
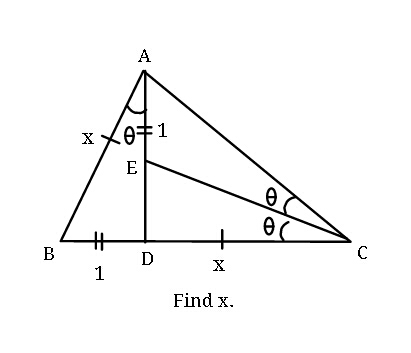
Question Number 43616 Answers: 1 Comments: 0
Question Number 43613 Answers: 0 Comments: 0
Question Number 43599 Answers: 0 Comments: 0
Question Number 43608 Answers: 0 Comments: 3
Question Number 43606 Answers: 0 Comments: 0

Question Number 43603 Answers: 1 Comments: 0
Question Number 43602 Answers: 1 Comments: 0
Question Number 43596 Answers: 0 Comments: 0

Question Number 43574 Answers: 1 Comments: 0
Question Number 43571 Answers: 1 Comments: 0

Question Number 43569 Answers: 0 Comments: 1
Question Number 43551 Answers: 1 Comments: 0
Question Number 43550 Answers: 0 Comments: 1
Question Number 43549 Answers: 1 Comments: 1
Question Number 43546 Answers: 1 Comments: 0
Question Number 43545 Answers: 1 Comments: 0
Question Number 43544 Answers: 1 Comments: 0
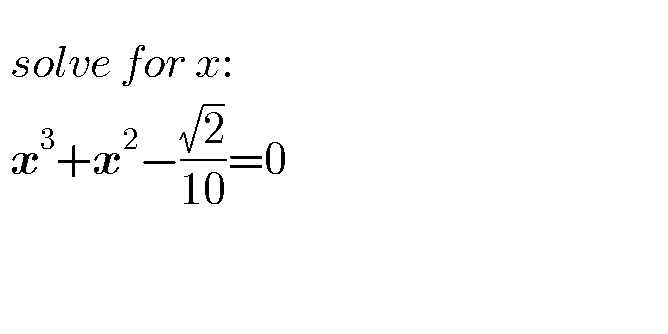
Question Number 43543 Answers: 1 Comments: 0
Question Number 43540 Answers: 2 Comments: 0
Question Number 43539 Answers: 0 Comments: 1
Question Number 43538 Answers: 0 Comments: 1
Question Number 43541 Answers: 1 Comments: 0
Pg 1631 Pg 1632 Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638 Pg 1639 Pg 1640
