
AllQuestion and Answers: Page 1635
Question Number 44201 Answers: 1 Comments: 4
Question Number 44200 Answers: 0 Comments: 4
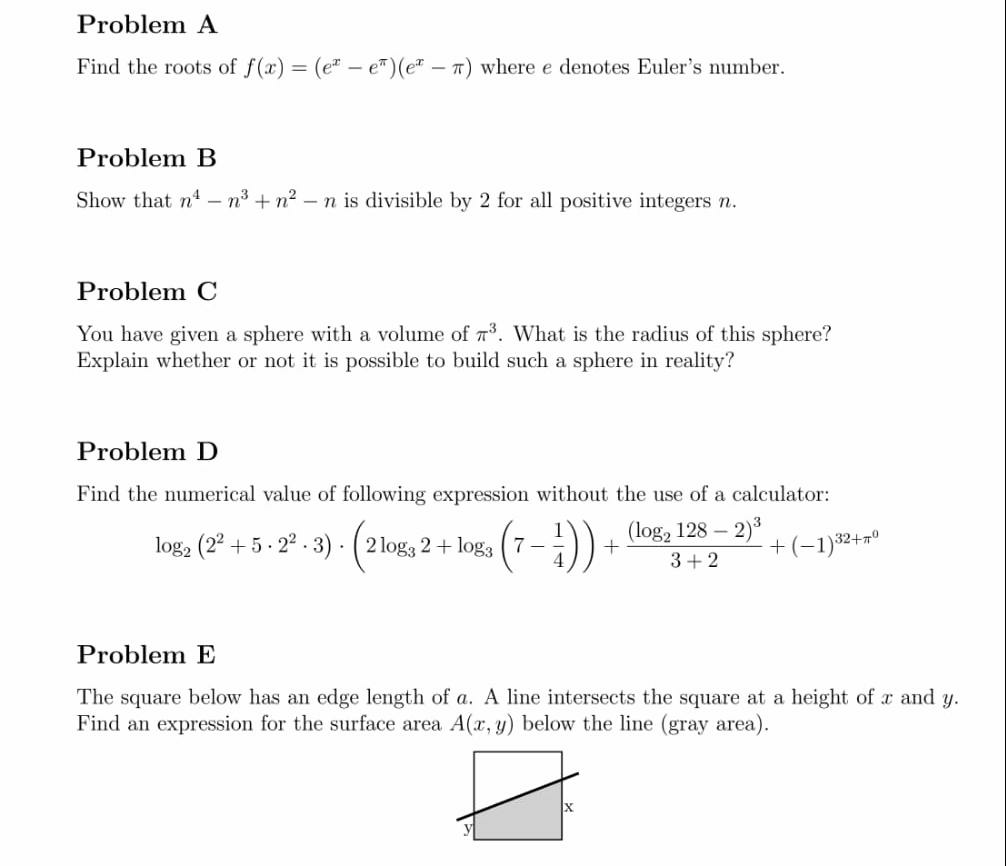
Question Number 44194 Answers: 2 Comments: 3

Question Number 44190 Answers: 1 Comments: 1
Question Number 44179 Answers: 1 Comments: 1
Question Number 44178 Answers: 0 Comments: 0
Question Number 44176 Answers: 0 Comments: 1
Question Number 44175 Answers: 0 Comments: 2
Question Number 44174 Answers: 0 Comments: 0
Question Number 44173 Answers: 1 Comments: 1
Question Number 44161 Answers: 2 Comments: 0
Question Number 44159 Answers: 3 Comments: 0
Question Number 44148 Answers: 1 Comments: 0
$$\int{dx}/{sinx}\centerdot{sin}\left({x}+\alpha\right)=? \\ $$
Question Number 44142 Answers: 1 Comments: 0
Question Number 44128 Answers: 1 Comments: 0
Question Number 44120 Answers: 1 Comments: 0

Question Number 44117 Answers: 0 Comments: 0

Question Number 44103 Answers: 1 Comments: 1

Question Number 44098 Answers: 3 Comments: 0
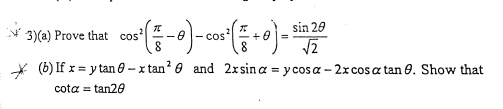
Question Number 44097 Answers: 3 Comments: 0

Question Number 44096 Answers: 2 Comments: 0

Question Number 44095 Answers: 1 Comments: 0

Question Number 44094 Answers: 2 Comments: 2

Question Number 44092 Answers: 0 Comments: 1

Question Number 44091 Answers: 1 Comments: 0
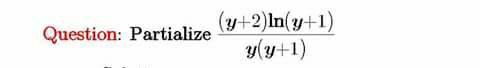
Question Number 44089 Answers: 0 Comments: 0

Pg 1630 Pg 1631 Pg 1632 Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638 Pg 1639
