Question and Answers Forum

AllQuestion and Answers: Page 1634

Question Number 44267 Answers: 1 Comments: 0

If xε ((1/(√2)) , 1) ,differentiate cos^(−1) (2x(√(1−x^2 ))).

$${If}\:{x}\epsilon\:\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:,\:\mathrm{1}\right)\:,{differentiate}\:\mathrm{cos}^{−\mathrm{1}} \left(\mathrm{2}{x}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\right). \\ $$

Question Number 44264 Answers: 1 Comments: 1

∫(1/((x^2 +2x+5)^2 ))dx

$$\int\frac{\mathrm{1}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2x}+\mathrm{5}\right)^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 44250 Answers: 1 Comments: 0

Evaliate ∫sin^4 x dx please show working.

$$\mathrm{Evaliate}\:\int\mathrm{sin}^{\mathrm{4}} \mathrm{x}\:\mathrm{dx} \\ $$$$\mathrm{please}\:\mathrm{show}\:\mathrm{working}. \\ $$

Question Number 44236 Answers: 0 Comments: 0

in howmanyways can 6 people sit around a roundtable? willbe happy when workings are shown PLEASE.

$$\mathrm{in}\:\mathrm{howmanyways}\:\mathrm{can}\:\mathrm{6}\:\mathrm{people}\:\mathrm{sit}\:\mathrm{around}\:\mathrm{a}\:\mathrm{roundtable}? \\ $$$$\mathrm{willbe}\:\mathrm{happy}\:\mathrm{when}\:\mathrm{workings}\:\mathrm{are}\:\mathrm{shown}\:\mathrm{PLEASE}. \\ $$

Question Number 44237 Answers: 2 Comments: 0

in how many ways can 6 people sit around a roundtable? I need workings please?

$$\mathrm{in}\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{6}\:\mathrm{people}\:\mathrm{sit}\:\mathrm{around}\:\mathrm{a}\:\mathrm{roundtable}? \\ $$$$\mathrm{I}\:\mathrm{need}\:\mathrm{workings}\:\mathrm{please}? \\ $$

Question Number 44232 Answers: 1 Comments: 0

∫_0 ^π e^(sin^2 x) Cos^3 xdx

$$\int_{\mathrm{0}} ^{\pi} \mathrm{e}^{\mathrm{sin}^{\mathrm{2}} \mathrm{x}} \mathrm{Cos}^{\mathrm{3}} \mathrm{xdx} \\ $$

Question Number 44231 Answers: 1 Comments: 0

The square root of x^(m^2 −n^2 ) ∙ x^(n^2 +2mn) ∙ x^n^2 is

$$\mathrm{The}\:\mathrm{square}\:\mathrm{root}\:\mathrm{of}\:\:{x}^{{m}^{\mathrm{2}} −{n}^{\mathrm{2}} } \centerdot\:{x}^{{n}^{\mathrm{2}} +\mathrm{2}{mn}} \centerdot\:{x}^{{n}^{\mathrm{2}} } \:\mathrm{is} \\ $$

Question Number 44230 Answers: 1 Comments: 0

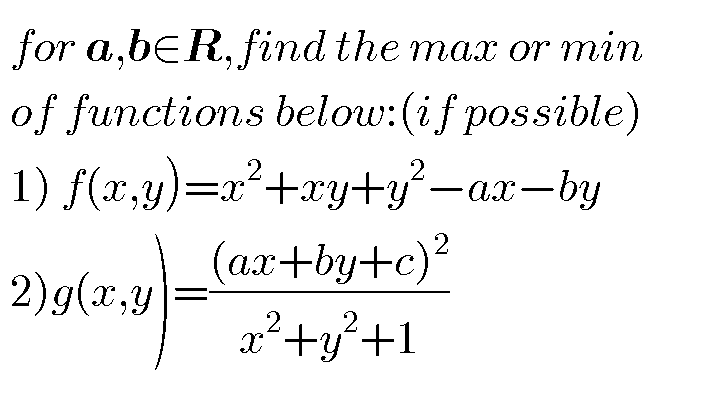
Question Number 44222 Answers: 2 Comments: 0

A man borrowed x at 5% per annum simple interest.He paid 198000 after 2years.Calculate _ a)the value of x b)simple interest

$${A}\:{man}\:{borrowed}\:{x}\:{at}\:\mathrm{5\%}\:{per}\:{annum} \\ $$$${simple}\:{interest}.{He}\:{paid}\:\mathrm{198000}\: \\ $$$${after}\:\mathrm{2}{years}.{Calculate}\:_{} \\ $$$$\left.{a}\right){the}\:{value}\:{of}\:{x} \\ $$$$\left.{b}\right){simple}\:{interest} \\ $$

Question Number 44214 Answers: 1 Comments: 0

find x inthe eqn. 3^x −1=x^3

$$\mathrm{find}\:\mathrm{x}\:\mathrm{inthe}\:\mathrm{eqn}. \\ $$$$\mathrm{3}^{\mathrm{x}} −\mathrm{1}=\mathrm{x}^{\mathrm{3}} \\ $$

Question Number 44212 Answers: 1 Comments: 0

solve for x if. 3^2 −1=x^3

$$\mathrm{solve}\:\mathrm{for}\:\mathrm{x}\:\mathrm{if}. \\ $$$$\mathrm{3}^{\mathrm{2}} −\mathrm{1}=\mathrm{x}^{\mathrm{3}} \\ $$

Question Number 44209 Answers: 0 Comments: 0

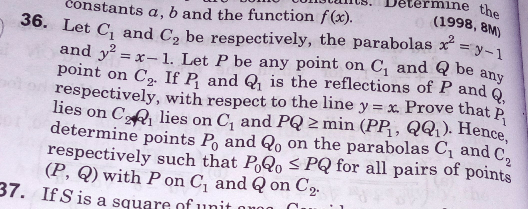
Question Number 44208 Answers: 0 Comments: 3

Question Number 44202 Answers: 1 Comments: 4

find f(a) =∫_0 ^∞ (dx/(x^3 +a^3 )) with a>0 2)find g(a)=∫_0 ^∞ (dx/((x^3 +a^3 )^2 )) 3)find the value of ∫_0 ^∞ (dx/((1+x^3 )^2 )) 4)find the value of ∫_0 ^∞ (dx/(8x^3 +1))

$${find}\:\:{f}\left({a}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{{x}^{\mathrm{3}} \:+{a}^{\mathrm{3}} }\:{with}\:{a}>\mathrm{0} \\ $$$$\left.\mathrm{2}\right){find}\:{g}\left({a}\right)=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\left({x}^{\mathrm{3}} \:+{a}^{\mathrm{3}} \right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{3}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{3}} \right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{4}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\mathrm{8}{x}^{\mathrm{3}} \:+\mathrm{1}} \\ $$

Question Number 44201 Answers: 1 Comments: 4

let f(x)=∫_0 ^1 ((ln(1+xt^2 ))/t^2 )dt with x ∈R 1) find a explicit form of f(x) 2)calculate ∫_0 ^1 ((ln(1+t^2 ))/t^2 )dt 3)calculate ∫_0 ^1 ((ln(1+2t^2 ))/t^2 )dt 4) calculate ∫_0 ^1 ((ln(1−t^2 ))/t^2 )dt

$${let}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)}{{t}^{\mathrm{2}} }{dt}\:\:{with}\:{x}\:\in{R} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right){calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{{t}^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{3}\right){calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{t}^{\mathrm{2}} \right)}{{t}^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{4}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{t}^{\mathrm{2}} \right)}{{t}^{\mathrm{2}} }{dt} \\ $$

Question Number 44200 Answers: 0 Comments: 4

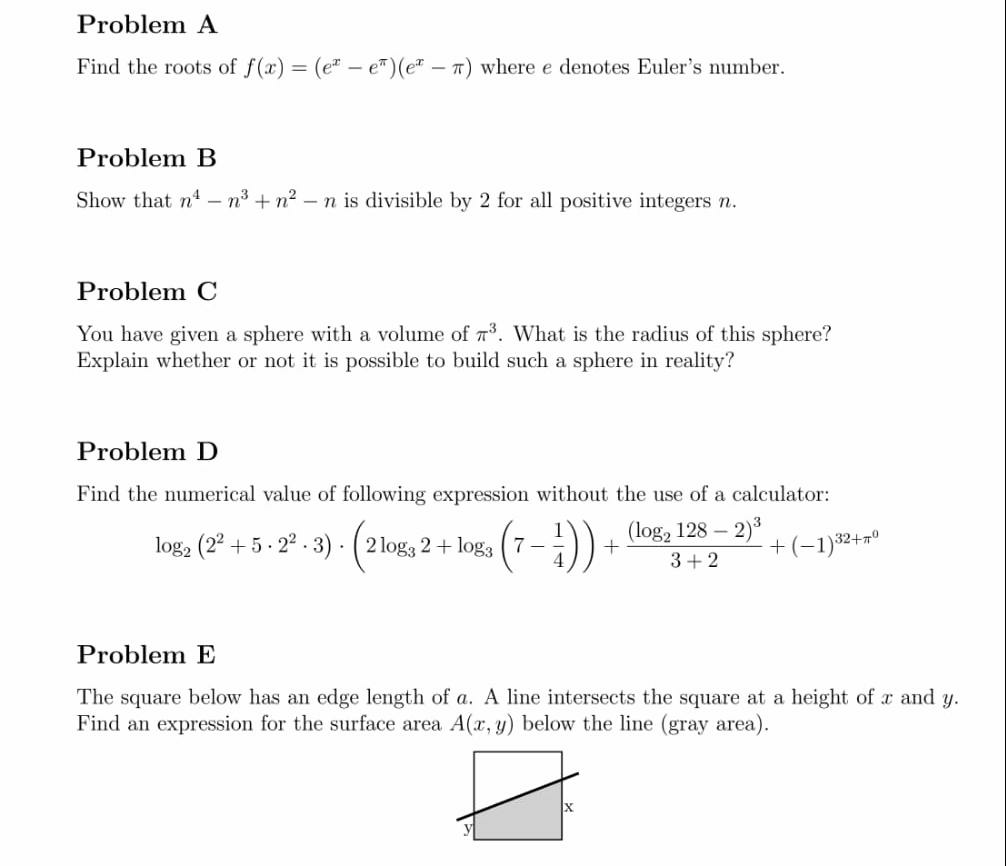
Question Number 44194 Answers: 2 Comments: 3

Question Number 44190 Answers: 1 Comments: 1

Q..Find second derivative.. 5^(x ) solve please stap by stap

$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$${Q}..{Find}\:{second}\:{derivative}.. \\ $$$$\:\:\mathrm{5}^{{x}\:\:\:\:} {solve}\:{please}\:{stap}\:{by}\:{stap} \\ $$

Question Number 44179 Answers: 1 Comments: 1

1) find ∫ (dx/((√(x^2 +x+1))+(√(x^2 −x+1)))) 2)calculate ∫_0 ^1 (dx/((√(x^2 +x+1))+(√(x^2 −x +1))))

$$\left.\mathrm{1}\right)\:{find}\:\int\:\:\:\frac{{dx}}{\sqrt{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}+\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}} \\ $$$$\left.\mathrm{2}\right){calculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\:\frac{{dx}}{\sqrt{{x}^{\mathrm{2}} \:+{x}+\mathrm{1}}+\sqrt{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}}} \\ $$

Question Number 44178 Answers: 0 Comments: 0

find f(x)=∫_0 ^π ((sin^2 t)/((x^2 −2x cost +1)^2 ))dt 2)find the value of ∫_0 ^π ((sin^2 t)/((x^2 −cost +1)^2 ))dt

$${find}\:\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\pi} \:\:\frac{{sin}^{\mathrm{2}} {t}}{\left({x}^{\mathrm{2}} −\mathrm{2}{x}\:{cost}\:+\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\pi} \:\:\frac{{sin}^{\mathrm{2}} {t}}{\left({x}^{\mathrm{2}} −{cost}\:+\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$

Question Number 44176 Answers: 0 Comments: 1

find A_n =∫_0 ^∞ (t^n −[t])e^(−nt) dt and lim_(n→+∞) A_n n integr natural.

$${find}\:{A}_{{n}} =\int_{\mathrm{0}} ^{\infty} \left({t}^{{n}} −\left[{t}\right]\right){e}^{−{nt}} {dt} \\ $$$${and}\:{lim}_{{n}\rightarrow+\infty} \:{A}_{{n}} \\ $$$${n}\:{integr}\:{natural}. \\ $$

Question Number 44175 Answers: 0 Comments: 2

find f(a) =∫_1 ^(+∞) (dx/(ch^2 x +a sh^2 x)) 2) find the value of ∫_1 ^(+∞) (dx/(ch^2 x+2sh^2 x))

$${find}\:\:{f}\left({a}\right)\:=\int_{\mathrm{1}} ^{+\infty} \:\:\frac{{dx}}{{ch}^{\mathrm{2}} {x}\:+{a}\:{sh}^{\mathrm{2}} {x}} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{1}} ^{+\infty} \:\:\:\frac{{dx}}{{ch}^{\mathrm{2}} {x}+\mathrm{2}{sh}^{\mathrm{2}} {x}} \\ $$

Question Number 44174 Answers: 0 Comments: 0

find ∫_(−∞) ^(+∞) (dx/((1+x^2 )(1+x e^(iθ) )))

$${find}\:\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left(\mathrm{1}+{x}\:{e}^{{i}\theta} \right)} \\ $$

Question Number 44173 Answers: 1 Comments: 1

calculate ∫_0 ^∞ (dt/((3+t^2 )(√(1+t))))dt

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dt}}{\left(\mathrm{3}+{t}^{\mathrm{2}} \right)\sqrt{\mathrm{1}+{t}}}{dt} \\ $$

Question Number 44161 Answers: 2 Comments: 0

LOL! found this on the web: 1=(√1)=(√((−1)(−1)))=(√(−1))(√(−1))=i^2 =−1 each step seems right, so where′s the mistake?

$$\mathrm{LOL}!\:\mathrm{found}\:\mathrm{this}\:\mathrm{on}\:\mathrm{the}\:\mathrm{web}: \\ $$$$\mathrm{1}=\sqrt{\mathrm{1}}=\sqrt{\left(−\mathrm{1}\right)\left(−\mathrm{1}\right)}=\sqrt{−\mathrm{1}}\sqrt{−\mathrm{1}}=\mathrm{i}^{\mathrm{2}} =−\mathrm{1} \\ $$$$\mathrm{each}\:\mathrm{step}\:\mathrm{seems}\:\mathrm{right},\:\mathrm{so}\:\mathrm{where}'\mathrm{s}\:\mathrm{the}\:\mathrm{mistake}? \\ $$

Question Number 44159 Answers: 3 Comments: 0

If sin^(−1) x + sin^(−1) y + sin^(−1) z =π prove that : a) x(√(1−x^2 )) + y(√(1−y^2 )) +z(√(1−z^2 ))= 2xyz b) x^4 +y^4 +z^4 +4x^2 y^2 z^2 = 2(x^2 y^2 +y^2 z^2 +z^2 x^2 ).

$${If}\:\mathrm{sin}^{−\mathrm{1}} {x}\:+\:\mathrm{sin}^{−\mathrm{1}} {y}\:+\:\mathrm{sin}^{−\mathrm{1}} \boldsymbol{{z}}\:=\pi\: \\ $$$${prove}\:{that}\:: \\ $$$$\left.{a}\right)\:{x}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\:+\:{y}\sqrt{\mathrm{1}−{y}^{\mathrm{2}} }\:+\boldsymbol{{z}}\sqrt{\mathrm{1}−\boldsymbol{{z}}^{\mathrm{2}} }=\:\mathrm{2}{xy}\boldsymbol{{z}} \\ $$$$\left.{b}\right)\:{x}^{\mathrm{4}} +{y}^{\mathrm{4}} +\boldsymbol{{z}}^{\mathrm{4}} +\mathrm{4}{x}^{\mathrm{2}} {y}^{\mathrm{2}} \boldsymbol{{z}}^{\mathrm{2}} =\:\mathrm{2}\left({x}^{\mathrm{2}} {y}^{\mathrm{2}} +{y}^{\mathrm{2}} \boldsymbol{{z}}^{\mathrm{2}} +\boldsymbol{{z}}^{\mathrm{2}} \boldsymbol{{x}}^{\mathrm{2}} \right). \\ $$

Pg 1629 Pg 1630 Pg 1631 Pg 1632 Pg 1633 Pg 1634 Pg 1635 Pg 1636 Pg 1637 Pg 1638

Contact: info@tinkutara.com