Question and Answers Forum

AllQuestion and Answers: Page 1633

Question Number 44546 Answers: 1 Comments: 0

Question Number 44543 Answers: 1 Comments: 3

If y =f(x) = ax^2 +bx+c and at some x, say x= p ∫_0 ^( p) ydx = y(p)= y ′(p) = y ′′(p)= p , then find p .

$${If}\:\:{y}\:={f}\left({x}\right)\:=\:{ax}^{\mathrm{2}} +{bx}+{c} \\ $$$${and}\:\:{at}\:{some}\:{x},\:{say}\:\:{x}=\:{p} \\ $$$$\int_{\mathrm{0}} ^{\:\:{p}} {ydx}\:=\:{y}\left({p}\right)=\:{y}\:'\left({p}\right)\:=\:{y}\:''\left({p}\right)=\:{p}\:, \\ $$$${then}\:{find}\:\boldsymbol{{p}}\:. \\ $$

Question Number 44541 Answers: 1 Comments: 1

Find the remainder when the polynomial p(y)=y^4 −3y^2 +2y+1 is divided by y−1.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{when}\:\mathrm{the} \\ $$$$\mathrm{polynomial}\:{p}\left({y}\right)={y}^{\mathrm{4}} −\mathrm{3}{y}^{\mathrm{2}} +\mathrm{2}{y}+\mathrm{1}\:\mathrm{is} \\ $$$$\mathrm{divided}\:\mathrm{by}\:{y}−\mathrm{1}. \\ $$

Question Number 44537 Answers: 1 Comments: 0

Question Number 44535 Answers: 0 Comments: 1

Question Number 44527 Answers: 0 Comments: 2

Question Number 44526 Answers: 1 Comments: 1

Find moment of inertia of the area bounded by the curve r^2 =a^2 cos2θ about its axis

$$\mathrm{Find}\:\mathrm{moment}\:\mathrm{of}\:\mathrm{inertia}\:\mathrm{of}\:\mathrm{the}\:\mathrm{area}\:\mathrm{bounded} \\ $$$$\mathrm{by}\:\mathrm{the}\:\mathrm{curve}\:\mathrm{r}^{\mathrm{2}} =\mathrm{a}^{\mathrm{2}} \mathrm{cos2}\theta \\ $$$$\mathrm{about}\:\mathrm{its}\:\mathrm{axis} \\ $$

Question Number 44515 Answers: 1 Comments: 0

let g(x) =∫_0 ^∞ ((t ln(t)dt)/((1+xt)^3 )) with x>0 1) give a explicit form of g(x) 2) calculate ∫_0 ^∞ ((t ln(t))/((1+t)^3 ))dt 3) calculate ∫_0 ^∞ ((tln(t))/((1+2t)^3 )) dt 4) calculate A(θ) =∫_0 ^∞ ((t ln(t))/((1+t sinθ)^3 ))dt with 0<θ<(π/2)

$${let}\:{g}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}\:{ln}\left({t}\right){dt}}{\left(\mathrm{1}+{xt}\right)^{\mathrm{3}} }\:{with}\:{x}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{give}\:{a}\:{explicit}\:{form}\:{of}\:{g}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}\:{ln}\left({t}\right)}{\left(\mathrm{1}+{t}\right)^{\mathrm{3}} }{dt} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\frac{{tln}\left({t}\right)}{\left(\mathrm{1}+\mathrm{2}{t}\right)^{\mathrm{3}} }\:{dt} \\ $$$$\left.\mathrm{4}\right)\:{calculate}\:{A}\left(\theta\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}\:{ln}\left({t}\right)}{\left(\mathrm{1}+{t}\:{sin}\theta\right)^{\mathrm{3}} }{dt}\:\:{with}\:\:\mathrm{0}<\theta<\frac{\pi}{\mathrm{2}} \\ $$

Question Number 44512 Answers: 1 Comments: 1

prove that:−∫2^(ln x) dx = ((x.2^(ln x) )/(ln(xe))) +C

$$\boldsymbol{{prove}}\:\boldsymbol{{that}}:−\int\mathrm{2}^{\boldsymbol{\mathrm{ln}}\:\boldsymbol{\mathrm{x}}} \:\boldsymbol{\mathrm{dx}}\:=\:\frac{\boldsymbol{\mathrm{x}}.\mathrm{2}^{\boldsymbol{\mathrm{ln}}\:\boldsymbol{\mathrm{x}}} }{\boldsymbol{\mathrm{ln}}\left(\boldsymbol{\mathrm{xe}}\right)}\:+\boldsymbol{\mathrm{C}} \\ $$$$ \\ $$

Question Number 44509 Answers: 1 Comments: 1

∫(√(tan x)) dx=?

$$\int\sqrt{\boldsymbol{\mathrm{tan}}\:\boldsymbol{\mathrm{x}}}\:\:\boldsymbol{\mathrm{dx}}=? \\ $$

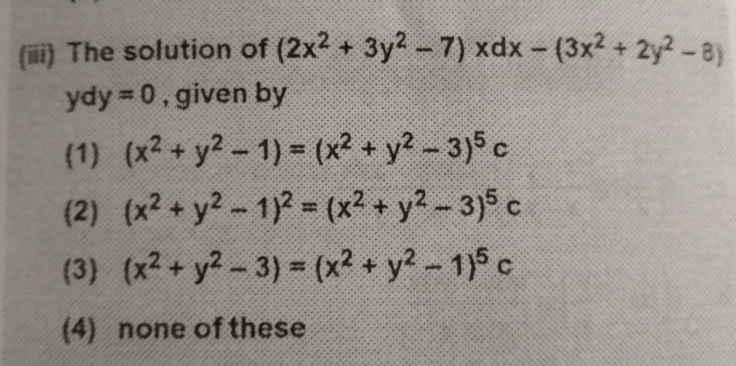
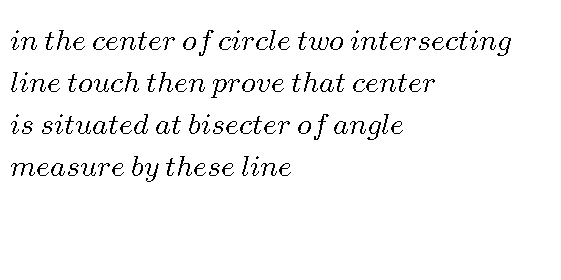
Question Number 44508 Answers: 1 Comments: 1

∫(√(sin x ))dx=?

$$\int\sqrt{\boldsymbol{\mathrm{sin}}\:\boldsymbol{\mathrm{x}}\:}\boldsymbol{\mathrm{dx}}=? \\ $$

Question Number 44502 Answers: 1 Comments: 0

If a>b,and c>d,prove that a−c may be greater than, equal to or less than b−d.

$$\mathrm{If}\:\mathrm{a}>\mathrm{b},\mathrm{and}\:\mathrm{c}>\mathrm{d},\mathrm{prove}\:\mathrm{that}\:\mathrm{a}−\mathrm{c}\:\mathrm{may}\:\mathrm{be}\:\mathrm{greater}\:\mathrm{than}, \\ $$$$\mathrm{equal}\:\mathrm{to}\:\mathrm{or}\:\mathrm{less}\:\mathrm{than}\:\mathrm{b}−\mathrm{d}. \\ $$$$ \\ $$

Question Number 44498 Answers: 0 Comments: 2

Question Number 44497 Answers: 0 Comments: 8

Question Number 44480 Answers: 2 Comments: 0

prove that ((9π)/(8 ))−(9/4)sin^(−1) (1/3)=(9/4)sin^(−1) ((2(√2))/3)

$${prove}\:{that}\:\:\frac{\mathrm{9}\pi}{\mathrm{8}\:\:}−\frac{\mathrm{9}}{\mathrm{4}}\mathrm{sin}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{9}}{\mathrm{4}}\mathrm{sin}^{−\mathrm{1}} \frac{\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{3}} \\ $$

Question Number 44479 Answers: 1 Comments: 5

Question Number 44478 Answers: 1 Comments: 0

prove that 2tan^(−1) ((√((a−b)/(a+b ))) tan (θ/2))=cos^(−1) (((b+acosθ)/(a+bcosθ)))

$${prove}\:{that}\:\mathrm{2tan}^{−\mathrm{1}} \left(\sqrt{\frac{{a}−{b}}{{a}+{b}\:}}\:\:\mathrm{tan}\:\frac{\theta}{\mathrm{2}}\right)=\mathrm{cos}^{−\mathrm{1}} \left(\frac{{b}+{acos}\theta}{{a}+{bcos}\theta}\right) \\ $$

Question Number 44491 Answers: 1 Comments: 0

prove that the sum of interior angles of any triangle is 180.

$$\boldsymbol{\mathrm{prove}}\:\boldsymbol{\mathrm{that}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{sum}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{interior}}\:\boldsymbol{\mathrm{angles}} \\ $$$$\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{any}}\:\boldsymbol{\mathrm{triangle}}\:\boldsymbol{\mathrm{is}}\:\mathrm{180}. \\ $$

Question Number 44476 Answers: 0 Comments: 6

let f(x) =∫_0 ^∞ (dt/(t^2 +2xt−1)) 1)find a explicit form of f(x) 2) cslvulste ∫_0 ^∞ (dt/(t^2 +t−1)) 3)calculate A(θ)=∫_0 ^∞ (dt/(t^2 +2tan(θ)t −1)) 4) calculate g(x)=∫_0 ^∞ ((tdt)/((t^2 +2xt−1)^2 )) 5)find the value of ∫_0 ^∞ ((tdt)/((t^2 +4t−1)^2 ))

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{2}{xt}−\mathrm{1}} \\ $$$$\left.\mathrm{1}\right){find}\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{cslvulste}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+{t}−\mathrm{1}} \\ $$$$\left.\mathrm{3}\right){calculate}\:{A}\left(\theta\right)=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{2}{tan}\left(\theta\right){t}\:−\mathrm{1}} \\ $$$$\left.\mathrm{4}\right)\:{calculate}\:{g}\left({x}\right)=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{tdt}}{\left({t}^{\mathrm{2}} \:+\mathrm{2}{xt}−\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{5}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{tdt}}{\left({t}^{\mathrm{2}} \:+\mathrm{4}{t}−\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Question Number 44475 Answers: 0 Comments: 0

find a and b if ∫_0 ^∞ ((√t) +a(√(t+1))+b(√(t+2)))dt converges and give its value in this case.

$${find}\:{a}\:{and}\:{b}\:\:{if}\:\int_{\mathrm{0}} ^{\infty} \:\left(\sqrt{{t}}\:+{a}\sqrt{{t}+\mathrm{1}}+{b}\sqrt{{t}+\mathrm{2}}\right){dt} \\ $$$${converges}\:{and}\:{give}\:{its}\:{value}\:{in}\:{this}\:{case}. \\ $$

Question Number 44473 Answers: 0 Comments: 1

let A_n =∫_0 ^∞ sin(n[t])e^(−t) dt 2)calculate A_n and lim_(n→+∞) n A_n 3)study the convergence of Σ_n A_n

$${let}\:{A}_{{n}} =\int_{\mathrm{0}} ^{\infty} \:{sin}\left({n}\left[{t}\right]\right){e}^{−{t}} {dt} \\ $$$$\left.\mathrm{2}\right){calculate}\:{A}_{{n}} \:\:{and}\:{lim}_{{n}\rightarrow+\infty} {n}\:{A}_{{n}} \\ $$$$\left.\mathrm{3}\right){study}\:{the}\:{convergence}\:{of}\:\sum_{{n}} \:{A}_{{n}} \\ $$

Question Number 44472 Answers: 0 Comments: 1

find f(x)=∫_0 ^∞ ((ln(t)dt)/((1+xt)^2 )) withx>0

$${find}\:\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\infty} \:\frac{{ln}\left({t}\right){dt}}{\left(\mathrm{1}+{xt}\right)^{\mathrm{2}} }\:{withx}>\mathrm{0} \\ $$

Question Number 44471 Answers: 0 Comments: 2

calculste ∫_0 ^∞ ((ln(x))/((1+x)^2 ))dx