
AllQuestion and Answers: Page 1632
Question Number 44001 Answers: 0 Comments: 0
Question Number 43999 Answers: 0 Comments: 1
Question Number 43992 Answers: 1 Comments: 1

Question Number 43991 Answers: 2 Comments: 0
Question Number 43990 Answers: 0 Comments: 1
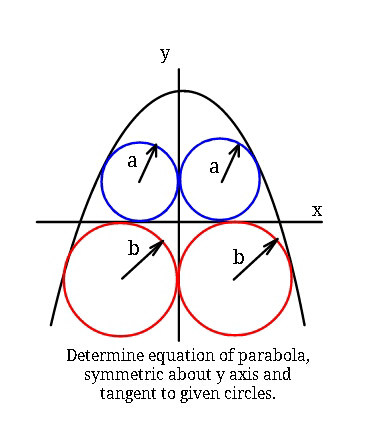
Question Number 43987 Answers: 0 Comments: 2

Question Number 43981 Answers: 0 Comments: 4
Question Number 43980 Answers: 1 Comments: 0

Question Number 43969 Answers: 0 Comments: 0

Question Number 43971 Answers: 1 Comments: 0

Question Number 43966 Answers: 0 Comments: 0

Question Number 43956 Answers: 0 Comments: 0

Question Number 43945 Answers: 1 Comments: 1

Question Number 43944 Answers: 1 Comments: 0
Question Number 43939 Answers: 0 Comments: 1
Question Number 43938 Answers: 0 Comments: 1
Question Number 43937 Answers: 1 Comments: 1
Question Number 43935 Answers: 0 Comments: 1
Question Number 43934 Answers: 0 Comments: 1
Question Number 43923 Answers: 1 Comments: 0
Question Number 43922 Answers: 0 Comments: 0
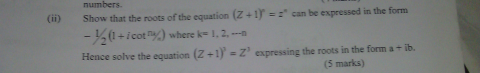
Question Number 43921 Answers: 1 Comments: 0
Question Number 43919 Answers: 0 Comments: 0
Question Number 43918 Answers: 0 Comments: 3
Question Number 43914 Answers: 0 Comments: 1
$${find}\:\int\:\:\:\:\frac{{x}}{\sqrt{\mathrm{1}+{cosx}}}{dx}\:. \\ $$
Question Number 43913 Answers: 2 Comments: 4
Pg 1627 Pg 1628 Pg 1629 Pg 1630 Pg 1631 Pg 1632 Pg 1633 Pg 1634 Pg 1635 Pg 1636
