Question and Answers Forum

All Questions   Topic List

AllQuestion and Answers: Page 1631

Question Number 44812    Answers: 0   Comments: 1

Question Number 44811    Answers: 0   Comments: 1

Question Number 44810    Answers: 1   Comments: 0

Question Number 44809    Answers: 0   Comments: 1

Question Number 44808    Answers: 1   Comments: 1

Question Number 44807    Answers: 1   Comments: 0

Question Number 44806    Answers: 1   Comments: 0

Question Number 44805    Answers: 1   Comments: 0

Question Number 44783    Answers: 1   Comments: 0

(x^2 )^2 +(y^2 )^2 =97.......1 (x^2 )^3 +(y^2 )^3 =793.......2 solve the simultaneous equation

$$\left({x}^{\mathrm{2}} \right)^{\mathrm{2}} +\left({y}^{\mathrm{2}} \right)^{\mathrm{2}} =\mathrm{97}.......\mathrm{1} \\ $$$$\left({x}^{\mathrm{2}} \right)^{\mathrm{3}} +\left({y}^{\mathrm{2}} \right)^{\mathrm{3}} =\mathrm{793}.......\mathrm{2} \\ $$$${solve}\:{the}\:{simultaneous}\:{equation} \\ $$

Question Number 44781    Answers: 2   Comments: 0

prove that:− ∫(1/(t(√(1−t^2 ))))dt = ln(1−(√(1−t^2 )))+C

$$\boldsymbol{{prove}}\:\boldsymbol{{that}}:−\:\int\frac{\mathrm{1}}{\boldsymbol{\mathrm{t}}\sqrt{\mathrm{1}−\boldsymbol{\mathrm{t}}^{\mathrm{2}} }}\boldsymbol{\mathrm{dt}}\:=\:\boldsymbol{\mathrm{ln}}\left(\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{\mathrm{t}}^{\mathrm{2}} }\right)+\boldsymbol{\mathrm{C}} \\ $$

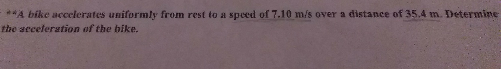
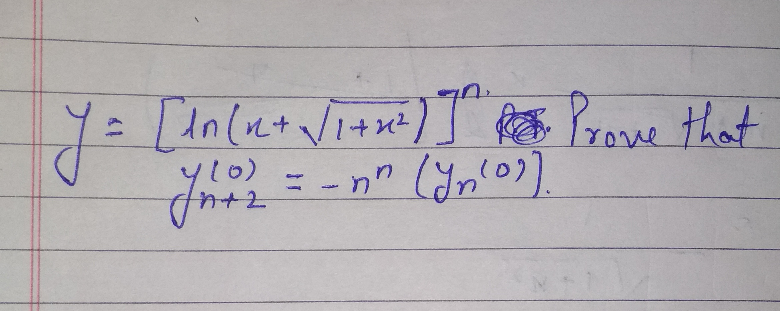
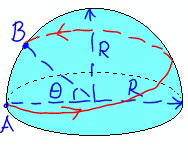
Question Number 44779    Answers: 0   Comments: 2

Question Number 44778    Answers: 0   Comments: 0

Question Number 44777    Answers: 0   Comments: 0

Question Number 44795    Answers: 1   Comments: 4

Question Number 44773    Answers: 0   Comments: 0

$$ \\ $$

Question Number 44765    Answers: 0   Comments: 0

Question Number 44763    Answers: 1   Comments: 1

Question Number 44761    Answers: 0   Comments: 1

Question Number 44749    Answers: 1   Comments: 1

Question Number 44737    Answers: 0   Comments: 0

A and B are two non−singular matrices such that A^6 =I and AB^2 =BA(B≠I). Then value of K for which B^K =I.

$${A}\:{and}\:{B}\:{are}\:{two}\:{non}−{singular}\:{matrices} \\ $$$${such}\:{that}\:{A}^{\mathrm{6}} ={I}\:{and}\:{AB}^{\mathrm{2}} ={BA}\left({B}\neq{I}\right). \\ $$$${Then}\:{value}\:{of}\:{K}\:{for}\:{which}\:{B}^{{K}} ={I}. \\ $$

Question Number 44730    Answers: 1   Comments: 1

Question Number 44729    Answers: 4   Comments: 0

Question Number 44716    Answers: 1   Comments: 0

prove: 1 + 11 + 111 + .... + ((111 ...111)/(n times)) = ((10^(n + 1) − 9n − 10)/(81))

$$\mathrm{prove}:\:\:\:\:\:\mathrm{1}\:+\:\mathrm{11}\:+\:\mathrm{111}\:+\:....\:+\:\frac{\mathrm{111}\:...\mathrm{111}}{\mathrm{n}\:\mathrm{times}}\:\:=\:\:\frac{\mathrm{10}^{\mathrm{n}\:+\:\mathrm{1}} \:−\:\mathrm{9n}\:−\:\mathrm{10}}{\mathrm{81}} \\ $$

Question Number 44712    Answers: 3   Comments: 0

Question Number 44708    Answers: 1   Comments: 0

Let A,B be two n×n matrices such that A+B=AB then prove : AB=BA ?

$${Let}\:{A},{B}\:{be}\:{two}\:{n}×{n}\:{matrices}\:{such} \\ $$$${that}\:{A}+{B}={AB}\:{then}\:{prove}\:: \\ $$$${AB}={BA}\:? \\ $$

Question Number 44702    Answers: 0   Comments: 0

  Pg 1626      Pg 1627      Pg 1628      Pg 1629      Pg 1630      Pg 1631      Pg 1632      Pg 1633      Pg 1634      Pg 1635   

Terms of Service

Privacy Policy

Contact: info@tinkutara.com