
AllQuestion and Answers: Page 1630
Question Number 44639 Answers: 0 Comments: 1
Question Number 44636 Answers: 1 Comments: 0

Question Number 44654 Answers: 1 Comments: 4
Question Number 44623 Answers: 1 Comments: 0
Question Number 44622 Answers: 1 Comments: 0
Question Number 44621 Answers: 2 Comments: 0

Question Number 44613 Answers: 0 Comments: 1
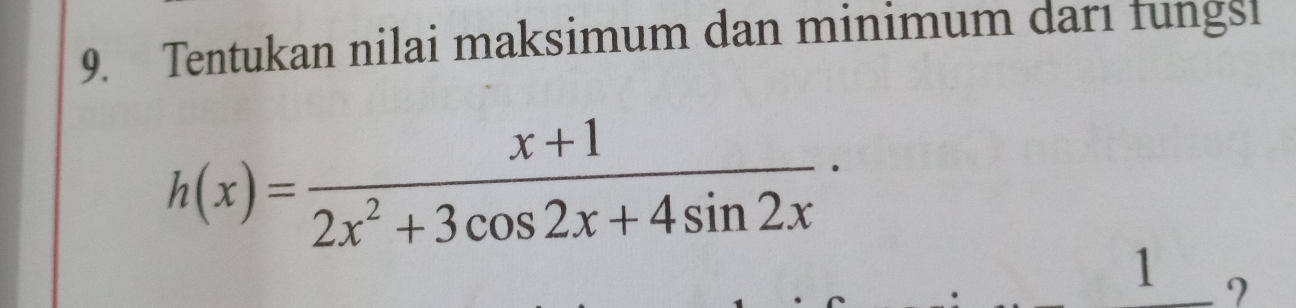
Question Number 44612 Answers: 2 Comments: 0
Question Number 44604 Answers: 1 Comments: 1
Question Number 44602 Answers: 0 Comments: 0
Question Number 44587 Answers: 1 Comments: 2
$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2018}} } \\ $$
Question Number 44584 Answers: 1 Comments: 5
Question Number 44674 Answers: 0 Comments: 1
Question Number 44570 Answers: 1 Comments: 0

Question Number 44573 Answers: 1 Comments: 1

Question Number 44575 Answers: 1 Comments: 3

Question Number 44557 Answers: 1 Comments: 2

Question Number 44548 Answers: 0 Comments: 0
Question Number 44546 Answers: 1 Comments: 0
Question Number 44543 Answers: 1 Comments: 3
Question Number 44541 Answers: 1 Comments: 1
Question Number 44537 Answers: 1 Comments: 0
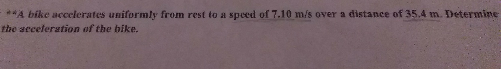
Question Number 44535 Answers: 0 Comments: 1
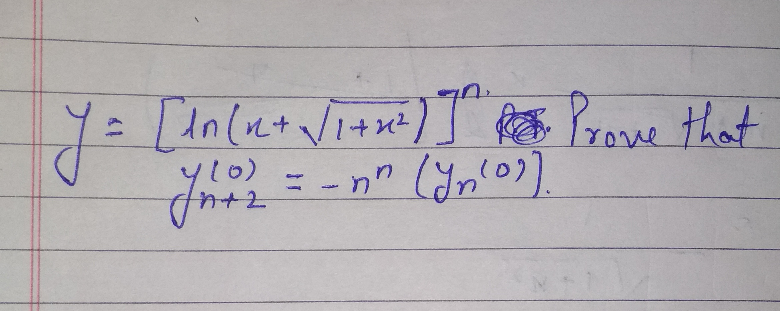
Question Number 44527 Answers: 0 Comments: 2

Question Number 44526 Answers: 1 Comments: 1
Question Number 44515 Answers: 1 Comments: 0
Pg 1625 Pg 1626 Pg 1627 Pg 1628 Pg 1629 Pg 1630 Pg 1631 Pg 1632 Pg 1633 Pg 1634
