
AllQuestion and Answers: Page 1614
Question Number 46636 Answers: 1 Comments: 0
Question Number 46631 Answers: 0 Comments: 1
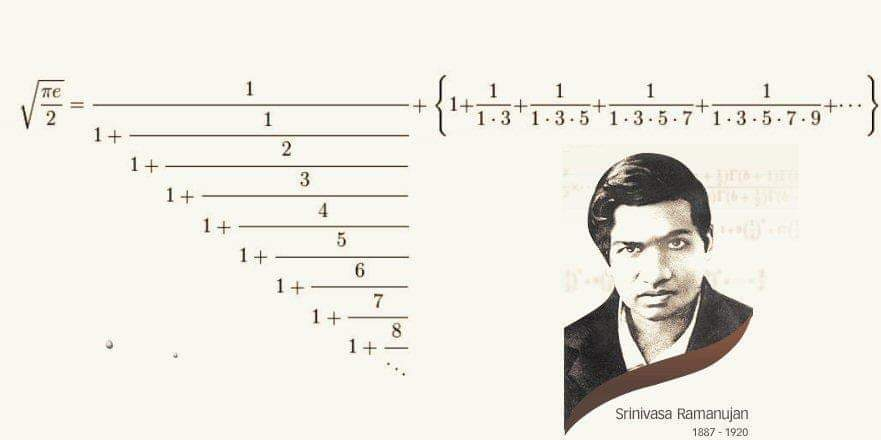
Question Number 46629 Answers: 1 Comments: 0
Question Number 46624 Answers: 1 Comments: 4
Question Number 46617 Answers: 1 Comments: 1
Question Number 46612 Answers: 1 Comments: 4
Question Number 46611 Answers: 2 Comments: 1
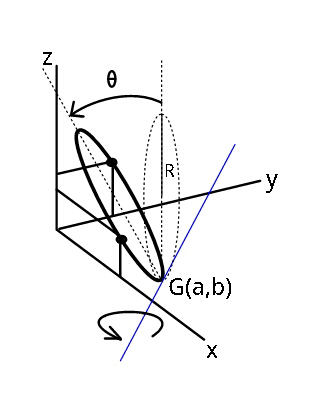
Question Number 46610 Answers: 0 Comments: 2
Question Number 46609 Answers: 0 Comments: 1
$${solve}\:\:\:\:{x}\:{y}^{''} \:−{e}^{−{x}} {y}^{'} \:\:\:={x}\:{sinx} \\ $$
Question Number 46608 Answers: 0 Comments: 0
Question Number 46607 Answers: 0 Comments: 1
Question Number 46604 Answers: 0 Comments: 1
Question Number 46598 Answers: 1 Comments: 1
Question Number 46594 Answers: 1 Comments: 0
$${find}\:\int\:\left(\sqrt{{x}+\sqrt{{x}}}−\sqrt{{x}−\sqrt{{x}}}\right){dx} \\ $$
Question Number 46592 Answers: 2 Comments: 1
Question Number 46586 Answers: 2 Comments: 0
Question Number 46576 Answers: 0 Comments: 2
Question Number 46573 Answers: 1 Comments: 4
Question Number 46570 Answers: 1 Comments: 0
Question Number 46569 Answers: 1 Comments: 0
Question Number 46568 Answers: 0 Comments: 0
Question Number 46567 Answers: 0 Comments: 0
Question Number 46563 Answers: 1 Comments: 0
Question Number 46558 Answers: 1 Comments: 0
Question Number 46553 Answers: 1 Comments: 0
Question Number 46552 Answers: 1 Comments: 2

Pg 1609 Pg 1610 Pg 1611 Pg 1612 Pg 1613 Pg 1614 Pg 1615 Pg 1616 Pg 1617 Pg 1618
