
AllQuestion and Answers: Page 1610
Question Number 47071 Answers: 0 Comments: 0
Question Number 47070 Answers: 0 Comments: 2
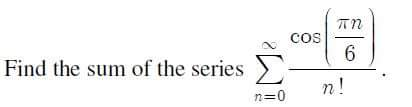
Question Number 47068 Answers: 1 Comments: 0
Question Number 47065 Answers: 0 Comments: 0
Question Number 47064 Answers: 0 Comments: 1
Question Number 47063 Answers: 0 Comments: 0
Question Number 47062 Answers: 1 Comments: 0
$${find}\:\int\:\:\:\sqrt{\frac{\sqrt{{x}}−\mathrm{1}}{\sqrt{{x}}+\mathrm{1}}}{dx} \\ $$
Question Number 47061 Answers: 0 Comments: 0
Question Number 47060 Answers: 0 Comments: 0
Question Number 47059 Answers: 2 Comments: 1
$$\:{find}\:\int\:\:\frac{{dx}}{\mathrm{1}+{cos}\:{x}\:+{cos}\left(\mathrm{2}{x}\right)} \\ $$
Question Number 47058 Answers: 1 Comments: 0
Question Number 47043 Answers: 0 Comments: 0
Question Number 47036 Answers: 0 Comments: 0
Question Number 47035 Answers: 1 Comments: 1
Question Number 47034 Answers: 1 Comments: 0
Question Number 47026 Answers: 4 Comments: 4

Question Number 47019 Answers: 2 Comments: 2

Question Number 47018 Answers: 1 Comments: 1
$${find}\:\int\:\sqrt{{x}+\mathrm{2}−\sqrt{{x}−\mathrm{1}}}{dx} \\ $$
Question Number 47010 Answers: 1 Comments: 1

Question Number 47006 Answers: 1 Comments: 1

Question Number 47005 Answers: 1 Comments: 1

Question Number 47001 Answers: 0 Comments: 0

Question Number 46998 Answers: 1 Comments: 0
Question Number 46996 Answers: 1 Comments: 0

Question Number 47098 Answers: 1 Comments: 8
Question Number 46983 Answers: 0 Comments: 0
Pg 1605 Pg 1606 Pg 1607 Pg 1608 Pg 1609 Pg 1610 Pg 1611 Pg 1612 Pg 1613 Pg 1614
