Question and Answers Forum

AllQuestion and Answers: Page 1602

Question Number 47964 Answers: 0 Comments: 0

A particle A at rest with position vector 2i−j with mass 500kg is hit by another particle B moving at a velocity of (5i−4j)ms^(−1) with mass 300kg. and they all move in the direction of B. a) What is the momentum after impact? b) Calculate the impulse generated,hence or otherwise, Calculate the distance A and B cover after impact at a time of 1minute.

$${A}\:{particle}\:{A}\:{at}\:{rest}\:{with}\:{position}\:{vector}\:\mathrm{2}{i}−{j}\:\:{with}\:{mass}\:\mathrm{500}{kg}\:{is}\:{hit}\:{by}\: \\ $$$${another}\:{particle}\:{B}\:{moving}\:{at}\:{a}\:{velocity}\:{of}\:\left(\mathrm{5}{i}−\mathrm{4}{j}\right){ms}^{−\mathrm{1}} \:{with}\:{mass}\:\mathrm{300}{kg}. \\ $$$${and}\:{they}\:{all}\:{move}\:{in}\:{the}\:{direction}\:{of}\:{B}. \\ $$$$\left.{a}\right)\:{What}\:{is}\:{the}\:{momentum}\:{after}\:{impact}? \\ $$$$\left.{b}\right)\:{Calculate}\:{the}\:{impulse}\:{generated},{hence}\:{or}\:{otherwise}, \\ $$$${Calculate}\:{the}\:{distance}\:{A}\:{and}\:{B}\:{cover}\:{after}\:{impact}\:{at}\: \\ $$$${a}\:{time}\:{of}\:\mathrm{1}{minute}. \\ $$$$ \\ $$

Question Number 47960 Answers: 1 Comments: 0

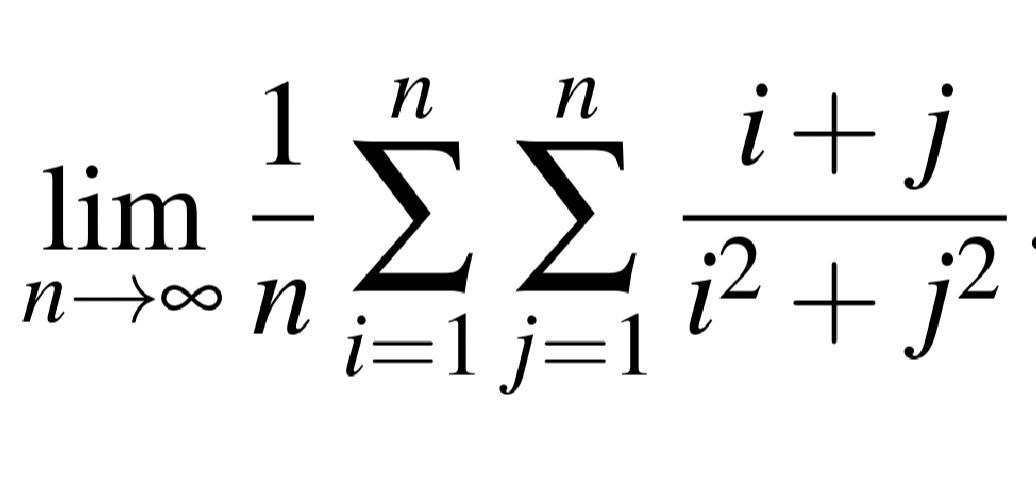
Question Number 47979 Answers: 0 Comments: 8

Question Number 47957 Answers: 3 Comments: 3

Question Number 47947 Answers: 0 Comments: 1

Question Number 47939 Answers: 1 Comments: 2

Question Number 47938 Answers: 0 Comments: 1

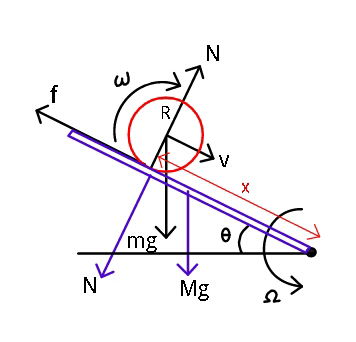
Question Number 47932 Answers: 1 Comments: 1

Question Number 47917 Answers: 0 Comments: 0

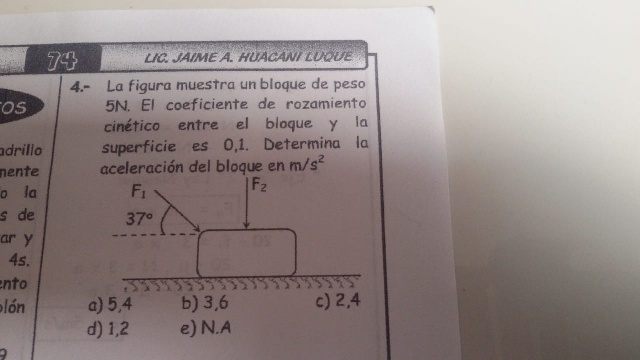
Question Number 47916 Answers: 2 Comments: 1

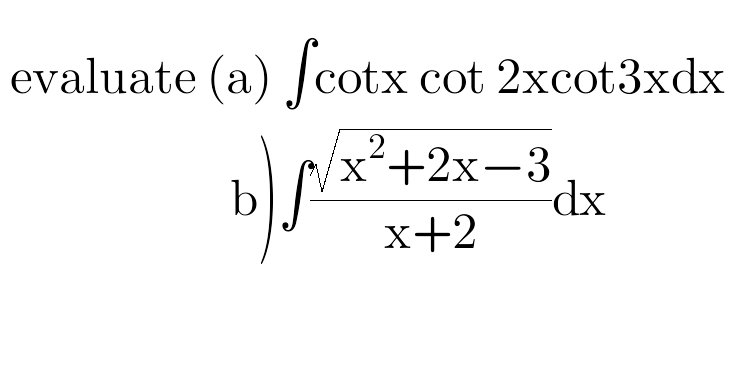
Question Number 47915 Answers: 2 Comments: 0

Question Number 47912 Answers: 0 Comments: 0

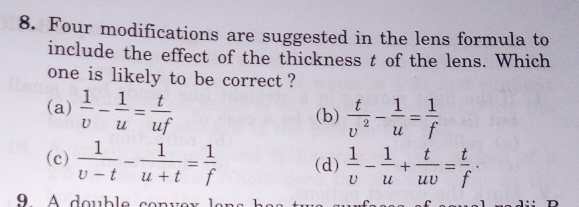
Question Number 47908 Answers: 0 Comments: 0

Question Number 47906 Answers: 0 Comments: 2

Question Number 47903 Answers: 0 Comments: 6

Question Number 47902 Answers: 0 Comments: 1

Question Number 47900 Answers: 0 Comments: 0

thanks sir

$${thanks}\:{sir} \\ $$

Question Number 47899 Answers: 0 Comments: 0

Question Number 47896 Answers: 1 Comments: 0

3(5/7)/2(1/7)=×/1.5 sir plz help me

$$\mathrm{3}\frac{\mathrm{5}}{\mathrm{7}}/\mathrm{2}\frac{\mathrm{1}}{\mathrm{7}}=×/\mathrm{1}.\mathrm{5}\:\:\:\:\:\mathrm{sir}\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me} \\ $$$$ \\ $$

Question Number 47883 Answers: 2 Comments: 1

Question Number 47881 Answers: 0 Comments: 1

Question Number 47874 Answers: 0 Comments: 0

An elevator starts with m passengers and stops at n floors (m≤n). The probability that no passengers alight at the same floor is

$$\mathrm{An}\:\mathrm{elevator}\:\mathrm{starts}\:\mathrm{with}\:{m}\:\mathrm{passengers} \\ $$$$\mathrm{and}\:\mathrm{stops}\:\mathrm{at}\:{n}\:\mathrm{floors}\:\left({m}\leqslant{n}\right).\:\mathrm{The}\: \\ $$$$\mathrm{probability}\:\mathrm{that}\:\mathrm{no}\:\mathrm{passengers}\:\mathrm{alight} \\ $$$$\mathrm{at}\:\mathrm{the}\:\mathrm{same}\:\mathrm{floor}\:\mathrm{is} \\ $$

Question Number 47863 Answers: 0 Comments: 0

find ∫ (√(1−x^4 ))dx

$${find}\:\int\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }{dx} \\ $$

Question Number 47860 Answers: 0 Comments: 1

Anyone know how it came to the working equation?

$$\mathrm{Anyone}\:\mathrm{know}\:\mathrm{how}\:\mathrm{it}\:\mathrm{came}\:\mathrm{to}\:\mathrm{the}\: \\ $$$$\mathrm{working}\:\mathrm{equation}? \\ $$

Question Number 47857 Answers: 0 Comments: 1

let A_n =Σ_(k=0) ^(n−1) sin(((kπ)/(2n))) and B_n =Σ_(k=0) ^(n−1) cos(((kπ)/(2n))) 1) find A_n and B_n interms of n 2)calculate lim_(n→+∞) (A_n /B_n ) 3)calculate ((lim_(n→+∞) A_n )/(lim_(n→+∞ ) B_n )) .

$${let}\:{A}_{{n}} =\sum_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \:{sin}\left(\frac{{k}\pi}{\mathrm{2}{n}}\right)\:{and}\:{B}_{{n}} =\sum_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \:{cos}\left(\frac{{k}\pi}{\mathrm{2}{n}}\right) \\ $$$$\left.\mathrm{1}\right)\:{find}\:{A}_{{n}} \:{and}\:{B}_{{n}} \:{interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right){calculate}\:{lim}_{{n}\rightarrow+\infty} \:\:\frac{{A}_{{n}} }{{B}_{{n}} } \\ $$$$\left.\mathrm{3}\right){calculate}\:\frac{{lim}_{{n}\rightarrow+\infty} {A}_{{n}} }{{lim}_{{n}\rightarrow+\infty\:\:} {B}_{{n}} }\:. \\ $$

Question Number 47852 Answers: 0 Comments: 1

1) find ∫ x arctan(x)dx 2) find the value of ∫_0 ^1 x arctan(x)dx

$$\left.\mathrm{1}\right)\:{find}\:\int\:{x}\:{arctan}\left({x}\right){dx} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}\:{arctan}\left({x}\right){dx} \\ $$

Pg 1597 Pg 1598 Pg 1599 Pg 1600 Pg 1601 Pg 1602 Pg 1603 Pg 1604 Pg 1605 Pg 1606

Contact: info@tinkutara.com