Question and Answers Forum

AllQuestion and Answers: Page 1601

Question Number 47624 Answers: 1 Comments: 0

Solve the d.e using method of variation of parameter. (d^2 y/dx^2 )+3(dy/dx)+2y=sin(e^x )

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{d}.\mathrm{e}\:\mathrm{using}\:\mathrm{method}\:\mathrm{of}\:\mathrm{variation} \\ $$$$\mathrm{of}\:\mathrm{parameter}. \\ $$$$ \\ $$$$\:\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }+\mathrm{3}\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{2y}=\mathrm{sin}\left(\mathrm{e}^{\mathrm{x}} \right) \\ $$

Question Number 47623 Answers: 0 Comments: 0

Solve the d.e using method of variation of parameter. (d^2 y/dx^2 )+3(dy/dx)+2y=sin(e^x )

Question Number 47613 Answers: 1 Comments: 0

1(8/9)=((2x−1)/5) sir plz help me

$$\mathrm{1}\frac{\mathrm{8}}{\mathrm{9}}=\frac{\mathrm{2x}−\mathrm{1}}{\mathrm{5}}\:\:\:\mathrm{sir}\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me} \\ $$

Question Number 47621 Answers: 1 Comments: 1

Find locus of point P from which tangents PA & PB to circles x^2 +y^2 =a^2 and x^2 +y^2 =b^2 respectively are perpendicular.

$${Find}\:{locus}\:{of}\:{point}\:{P}\:{from}\:{which} \\ $$$${tangents}\:{PA}\:\&\:{PB}\:{to}\:{circles}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$${and}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={b}^{\mathrm{2}} \:{respectively}\:{are}\:{perpendicular}. \\ $$

Question Number 47607 Answers: 1 Comments: 1

∫_a ^b ((∣x∣)/x)dx

$$\int_{{a}} ^{{b}} \frac{\mid{x}\mid}{{x}}{dx} \\ $$

Question Number 47801 Answers: 1 Comments: 0

An object starts from rest and moves with a velocity of (3i+4j)ms^(−1) . It covers a distance of 400km.Find a) its acceleration b) the time taken to cover the distance 400km.

$${An}\:{object}\:{starts}\:{from}\:{rest}\:{and}\:{moves}\:{with}\:{a}\:{velocity}\:{of}\: \\ $$$$\left(\mathrm{3}{i}+\mathrm{4}{j}\right){ms}^{−\mathrm{1}} .\:{It}\:{covers}\:{a}\:{distance}\:{of}\:\mathrm{400}{km}.{Find}\: \\ $$$$\left.{a}\right)\:{its}\:{acceleration} \\ $$$$\left.{b}\right)\:{the}\:{time}\:{taken}\:{to}\:{cover}\:{the}\:{distance}\:\mathrm{400}{km}. \\ $$

Question Number 47595 Answers: 0 Comments: 3

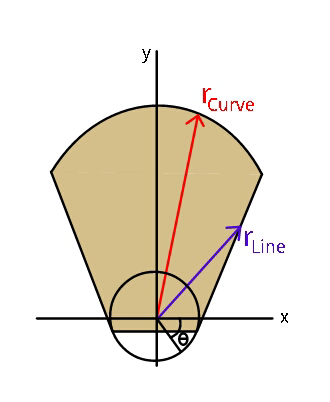
Question Number 47593 Answers: 0 Comments: 0

Which of the following statements are/is true?

$$\mathrm{Which}\:\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{statements}\: \\ $$$$\mathrm{are}/\mathrm{is}\:\mathrm{true}? \\ $$

Question Number 47590 Answers: 1 Comments: 1

f(x)=e^x g(x)=x^2 (f+g)(x)=? (f×x)(x)=? (f/g)(x)=?

$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{e}^{\mathrm{x}} \: \\ $$$$\mathrm{g}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} \\ $$$$\left(\mathrm{f}+\mathrm{g}\right)\left(\mathrm{x}\right)=? \\ $$$$\left(\mathrm{f}×\mathrm{x}\right)\left(\mathrm{x}\right)=? \\ $$$$\left(\mathrm{f}/\mathrm{g}\right)\left(\mathrm{x}\right)=? \\ $$

Question Number 47586 Answers: 1 Comments: 0

Given the function f(θ)= cos2θ − sinθ. (for 0°≤θ≤π) plot the graph for intervals of (π/6). hence find the value of cos2θ=sinθ.

$${Given}\:{the}\:{function}\:{f}\left(\theta\right)=\:{cos}\mathrm{2}\theta\:−\:{sin}\theta.\:\left({for}\:\mathrm{0}°\leqslant\theta\leqslant\pi\right) \\ $$$${plot}\:{the}\:{graph}\:{for}\:\:{intervals}\:{of}\:\frac{\pi}{\mathrm{6}}. \\ $$$${hence}\:{find}\:{the}\:{value}\:{of}\:{cos}\mathrm{2}\theta={sin}\theta. \\ $$

Question Number 47581 Answers: 1 Comments: 1

The root of the equation (2/(x−1)) + (1/(x+2)) + ((3x(x+1))/((x−1)(x+2))) = 0 among the following is

$$\mathrm{The}\:\mathrm{root}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\: \\ $$$$\frac{\mathrm{2}}{{x}−\mathrm{1}}\:+\:\frac{\mathrm{1}}{{x}+\mathrm{2}}\:+\:\frac{\mathrm{3}{x}\left({x}+\mathrm{1}\right)}{\left({x}−\mathrm{1}\right)\left({x}+\mathrm{2}\right)}\:=\:\mathrm{0}\:\: \\ $$$$\mathrm{among}\:\mathrm{the}\:\mathrm{following}\:\mathrm{is} \\ $$

Question Number 47565 Answers: 0 Comments: 0

Question Number 47561 Answers: 0 Comments: 0

thanks sir

$$\mathrm{thanks}\:\mathrm{sir} \\ $$

Question Number 47559 Answers: 1 Comments: 1

Question Number 47556 Answers: 1 Comments: 1

Question Number 47566 Answers: 2 Comments: 0

∫sin51x(sinx)^(49) dx

$$\int{sin}\mathrm{51}{x}\left({sinx}\right)^{\mathrm{49}} {dx} \\ $$

Question Number 47543 Answers: 2 Comments: 0

solve (1+ix)^n =n with x unknown real and n integr natural .

$${solve}\:\left(\mathrm{1}+{ix}\right)^{{n}} ={n}\:\:\:{with}\:{x}\:{unknown}\:{real}\:{and}\:{n}\:{integr}\:{natural}\:. \\ $$

Question Number 47552 Answers: 3 Comments: 1

((5(x−2))/4)=((6x−9)/5) plz help me

$$\frac{\mathrm{5}\left(\mathrm{x}−\mathrm{2}\right)}{\mathrm{4}}=\frac{\mathrm{6x}−\mathrm{9}}{\mathrm{5}}\:\:\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me} \\ $$

Question Number 47532 Answers: 2 Comments: 0

(√(400^2 +100^(2=) ))

$$\sqrt{\mathrm{400}^{\mathrm{2}} +\mathrm{100}^{\mathrm{2}=} } \\ $$

Question Number 47531 Answers: 1 Comments: 2

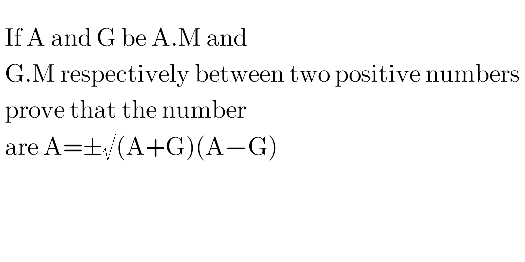
Question Number 47527 Answers: 0 Comments: 0

Question Number 47526 Answers: 0 Comments: 0

Question Number 47523 Answers: 0 Comments: 3

I have a question, Can we consider as a complex number a number if its real part or his imaginary part is null ? For example : a + b i where a=0 xor b=0. for example, a=7 and b=0 7 + 0i = 7 So, is a whole number for example may be considered as a complex number ?

$$\mathrm{I}\:\mathrm{have}\:\mathrm{a}\:\mathrm{question}, \\ $$$$\mathrm{Can}\:\mathrm{we}\:\mathrm{consider}\:\mathrm{as}\:\mathrm{a}\:\mathrm{complex}\:\mathrm{number}\:\mathrm{a} \\ $$$$\mathrm{number}\:\mathrm{if}\:\mathrm{its}\:\mathrm{real}\:\mathrm{part}\:\mathrm{or}\:\mathrm{his}\:\mathrm{imaginary} \\ $$$$\mathrm{part}\:\mathrm{is}\:\mathrm{null}\:? \\ $$$$\mathrm{For}\:\mathrm{example}\:: \\ $$$${a}\:+\:{b}\:{i} \\ $$$$\mathrm{where}\:\mathrm{a}=\mathrm{0}\:\mathrm{xor}\:\mathrm{b}=\mathrm{0}. \\ $$$$\mathrm{for}\:\mathrm{example}, \\ $$$$\mathrm{a}=\mathrm{7}\:\mathrm{and}\:\mathrm{b}=\mathrm{0} \\ $$$$\mathrm{7}\:+\:\mathrm{0}{i}\:=\:\mathrm{7} \\ $$$$\mathrm{So},\:\mathrm{is}\:\mathrm{a}\:\mathrm{whole}\:\mathrm{number}\:\mathrm{for}\:\mathrm{example}\:\mathrm{may}\:\mathrm{be} \\ $$$$\mathrm{considered}\:\mathrm{as}\:\mathrm{a}\:\mathrm{complex}\:\mathrm{number}\:? \\ $$

Question Number 47540 Answers: 1 Comments: 1

find ∫ arctan((√x))dx.

$${find}\:\int\:{arctan}\left(\sqrt{{x}}\right){dx}. \\ $$

Question Number 47519 Answers: 1 Comments: 1

Question Number 47515 Answers: 1 Comments: 1

Pg 1596 Pg 1597 Pg 1598 Pg 1599 Pg 1600 Pg 1601 Pg 1602 Pg 1603 Pg 1604 Pg 1605

Contact: info@tinkutara.com