
AllQuestion and Answers: Page 1600
Question Number 48208 Answers: 1 Comments: 0
Question Number 48204 Answers: 1 Comments: 0
Question Number 48202 Answers: 1 Comments: 1
Question Number 48196 Answers: 1 Comments: 1

Question Number 48194 Answers: 1 Comments: 1
Question Number 48193 Answers: 1 Comments: 0
Question Number 48187 Answers: 0 Comments: 0
Question Number 48182 Answers: 1 Comments: 0
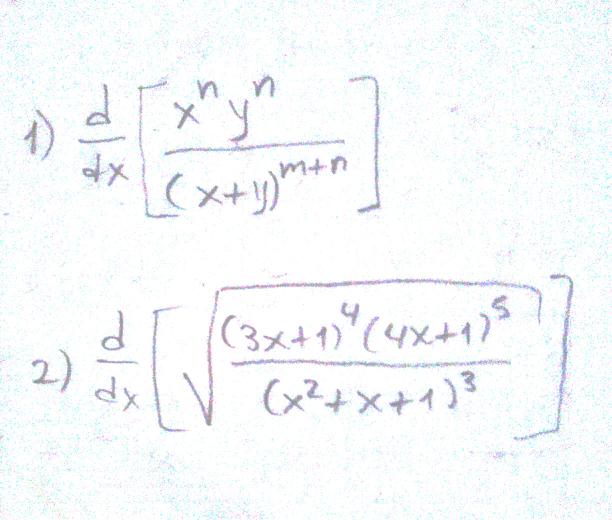
Question Number 48178 Answers: 1 Comments: 1
Question Number 48177 Answers: 0 Comments: 2
Question Number 48175 Answers: 1 Comments: 2
Question Number 48174 Answers: 1 Comments: 1
Question Number 48173 Answers: 1 Comments: 2
Question Number 48172 Answers: 0 Comments: 1
Question Number 48171 Answers: 0 Comments: 1
Question Number 48170 Answers: 1 Comments: 1
Question Number 48165 Answers: 0 Comments: 0
Question Number 48169 Answers: 0 Comments: 1
Question Number 48161 Answers: 1 Comments: 1
Question Number 48156 Answers: 2 Comments: 1
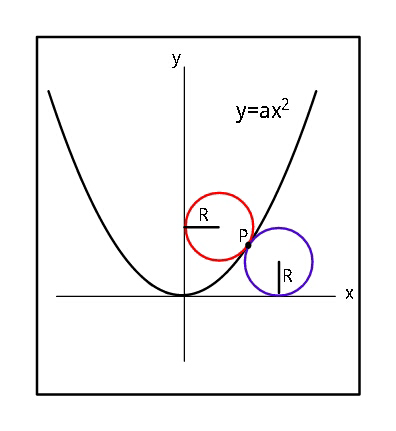
Question Number 48155 Answers: 1 Comments: 0
Question Number 48143 Answers: 1 Comments: 1
Question Number 48129 Answers: 1 Comments: 0
Question Number 48127 Answers: 1 Comments: 0

Question Number 48121 Answers: 0 Comments: 3
Question Number 48118 Answers: 0 Comments: 0
Pg 1595 Pg 1596 Pg 1597 Pg 1598 Pg 1599 Pg 1600 Pg 1601 Pg 1602 Pg 1603 Pg 1604
