
AllQuestion and Answers: Page 1599
Question Number 47883 Answers: 2 Comments: 1

Question Number 47881 Answers: 0 Comments: 1

Question Number 47874 Answers: 0 Comments: 0
Question Number 47863 Answers: 0 Comments: 0
$${find}\:\int\:\sqrt{\mathrm{1}−{x}^{\mathrm{4}} }{dx} \\ $$
Question Number 47860 Answers: 0 Comments: 1
Question Number 47857 Answers: 0 Comments: 1
Question Number 47852 Answers: 0 Comments: 1
Question Number 47851 Answers: 2 Comments: 3
Question Number 47848 Answers: 0 Comments: 2
Question Number 47837 Answers: 0 Comments: 0
Question Number 47836 Answers: 2 Comments: 0
$${prove}\:{cosec}^{\mathrm{2}} \theta−{cot}\theta{cosec}\theta=\mathrm{1} \\ $$
Question Number 47831 Answers: 2 Comments: 1
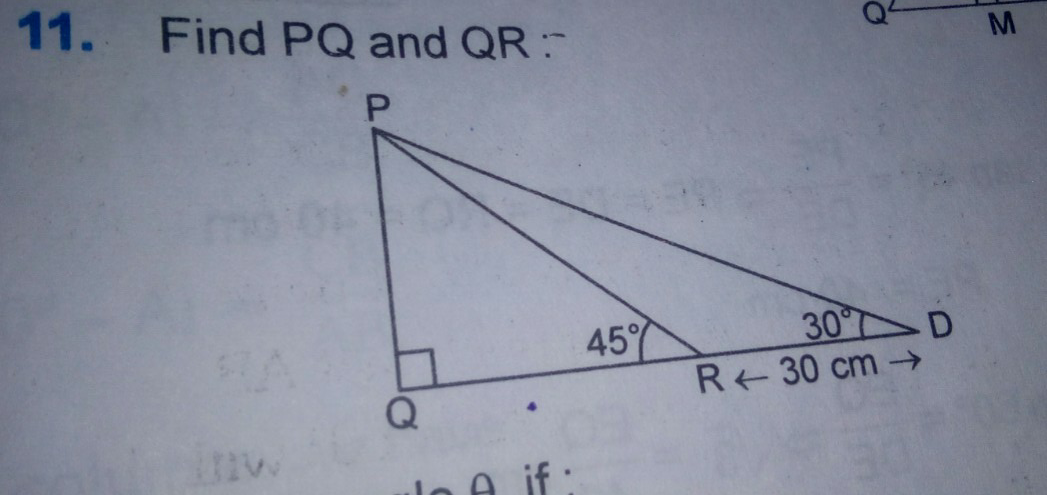
Question Number 47824 Answers: 2 Comments: 2

Question Number 47821 Answers: 1 Comments: 0
Question Number 47817 Answers: 1 Comments: 1

Question Number 47815 Answers: 1 Comments: 3

Question Number 47814 Answers: 1 Comments: 1
Question Number 47807 Answers: 1 Comments: 0
Question Number 47850 Answers: 1 Comments: 2
Question Number 47788 Answers: 0 Comments: 1

Question Number 47779 Answers: 0 Comments: 1

Question Number 47778 Answers: 1 Comments: 0
Question Number 47775 Answers: 2 Comments: 1

Question Number 47771 Answers: 0 Comments: 0
$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{x}}{{tanx}}{dx} \\ $$
Question Number 47770 Answers: 1 Comments: 3
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} −\mathrm{1}}{{lnx}}{dx} \\ $$
Question Number 47768 Answers: 0 Comments: 3
Pg 1594 Pg 1595 Pg 1596 Pg 1597 Pg 1598 Pg 1599 Pg 1600 Pg 1601 Pg 1602 Pg 1603
