Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1598

Question Number 49032 Answers: 2 Comments: 0

∫(1/(x^3 +1))dx=??

$$\int\frac{\mathrm{1}}{{x}^{\mathrm{3}} +\mathrm{1}}{dx}=?? \\ $$

Question Number 49028 Answers: 0 Comments: 0

n^2 −1 est olympique

$${n}^{\mathrm{2}} −\mathrm{1}\:{est}\:{olympique} \\ $$

Question Number 49020 Answers: 2 Comments: 2

Question Number 49008 Answers: 1 Comments: 0

Question Number 49007 Answers: 0 Comments: 0

Question Number 49006 Answers: 1 Comments: 0

Question Number 48993 Answers: 1 Comments: 0

Question Number 49014 Answers: 3 Comments: 1

Question Number 48984 Answers: 0 Comments: 1

Question Number 48983 Answers: 1 Comments: 0

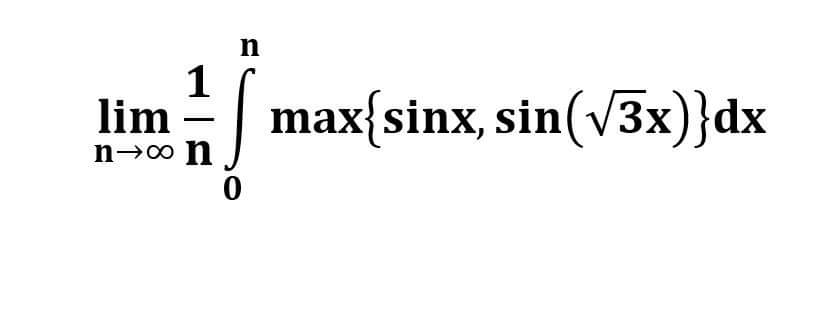
Question Number 48982 Answers: 2 Comments: 0

Question Number 48981 Answers: 1 Comments: 0

Question Number 48977 Answers: 2 Comments: 0

Question Number 48976 Answers: 1 Comments: 0

Question Number 48966 Answers: 1 Comments: 2

Question Number 48960 Answers: 1 Comments: 0

help me sir plz

$${help}\:{me}\:{sir}\:{plz} \\ $$

Question Number 49027 Answers: 0 Comments: 0

omoxnn dit qu un entier k est olympique s il existe 4 entiers a b c et d tous premiers avec k tel que k divise a^4 +b^4 +c^4 +d^4 .Soit n un entier naturel quelconque montrer que n^2 −1 divise a^4 +b^4 +c^4 +d^4

$${omoxnn}\:{dit}\:{qu}\:{un}\:{entier}\:{k}\:{est}\:{olympique}\:{s}\:{il}\:{existe}\:\mathrm{4}\:{entiers}\:{a}\:{b}\:{c}\:{et}\:{d}\:{tous}\:{premiers}\:{avec}\:{k}\:{tel}\:{que}\:{k}\:{divise}\:{a}^{\mathrm{4}} +{b}^{\mathrm{4}} +{c}^{\mathrm{4}} +{d}^{\mathrm{4}} .{Soit}\:{n}\:{un}\:{entier}\:{naturel}\:{quelconque} \\ $$$${montrer}\:{que}\:{n}^{\mathrm{2}} −\mathrm{1}\:{divise}\:{a}^{\mathrm{4}} +{b}^{\mathrm{4}} +{c}^{\mathrm{4}} +{d}^{\mathrm{4}} \\ $$

Question Number 48958 Answers: 1 Comments: 0

Given the position vectors v_1 = 2i−2j and v_2 =2j a) show that the unit vector in the direction of v_1 −v_2 = (1/(√5))(i−2j) b) Write down the equation of the line that contains the position vectors v_1 and v_2 c) Find the cosine of the angle between v_1 and v_2

$${Given}\:{the}\:{position}\:{vectors}\:{v}_{\mathrm{1}} =\:\mathrm{2}\boldsymbol{{i}}−\mathrm{2}\boldsymbol{{j}}\:{and}\:{v}_{\mathrm{2}} =\mathrm{2}\boldsymbol{{j}} \\ $$$$\left.{a}\right)\:{show}\:{that}\:{the}\:{unit}\:{vector}\:{in}\:{the}\:{direction}\:{of}\:{v}_{\mathrm{1}} −{v}_{\mathrm{2}} =\:\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left({i}−\mathrm{2}{j}\right) \\ $$$$\left.{b}\right)\:{Write}\:{down}\:{the}\:{equation}\:{of}\:{the}\:{line}\:{that}\:{contains} \\ $$$${the}\:{position}\:{vectors}\:{v}_{\mathrm{1}} \:{and}\:{v}_{\mathrm{2}} \\ $$$$\left.{c}\right)\:{Find}\:{the}\:{cosine}\:{of}\:{the}\:{angle}\:{between}\:{v}_{\mathrm{1}} \:{and}\:{v}_{\mathrm{2}} \\ $$

Question Number 48956 Answers: 3 Comments: 0

((x+0.2)/(x−0.2))=((1.2)/(2.2)) sir plz help me

$$\frac{{x}+\mathrm{0}.\mathrm{2}}{{x}−\mathrm{0}.\mathrm{2}}=\frac{\mathrm{1}.\mathrm{2}}{\mathrm{2}.\mathrm{2}} \\ $$$${sir}\:{plz}\:{help}\:{me} \\ $$

Question Number 48955 Answers: 3 Comments: 0

Evaluate ∫_0 ^((√3)/4) ((2xsin^(−1) (2x))/(√(1−4x^2 ))) dx

$${Evaluate}\:\underset{\mathrm{0}} {\overset{\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}} {\int}}\:\frac{\mathrm{2}{x}\mathrm{sin}^{−\mathrm{1}} \left(\mathrm{2}{x}\right)}{\sqrt{\mathrm{1}−\mathrm{4}{x}^{\mathrm{2}} }}\:{dx} \\ $$

Question Number 48953 Answers: 0 Comments: 1

Question Number 48952 Answers: 1 Comments: 0

Question Number 48921 Answers: 3 Comments: 1

Question Number 48920 Answers: 1 Comments: 0

Question Number 48919 Answers: 0 Comments: 1

if p and q are lengrhs of the line segment of any focal chord of parabola y^2 =4ax. where p,q are the roots of the equation (5+^ (√)2 )x^2 −(4+(√5))x+(4+(√)5)=0 then the length of the semi letusrectum of the parabola is ans:2

$$\mathrm{if}\:\mathrm{p}\:\mathrm{and}\:\mathrm{q}\:\mathrm{are}\:\mathrm{lengrhs}\:\mathrm{of}\:\mathrm{the}\:\mathrm{line}\:\mathrm{segment} \\ $$$$\mathrm{of}\:\mathrm{any}\:\mathrm{focal}\:\mathrm{chord}\:\mathrm{of}\:\mathrm{parabola}\:\mathrm{y}^{\mathrm{2}} =\mathrm{4ax}. \\ $$$$\mathrm{where}\:\mathrm{p},\mathrm{q}\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\left(\mathrm{5}+^{} \sqrt{}\mathrm{2}\:\right)\mathrm{x}^{\mathrm{2}} −\left(\mathrm{4}+\sqrt{\mathrm{5}}\right)\mathrm{x}+\left(\mathrm{4}+\sqrt{}\mathrm{5}\right)=\mathrm{0}\:\mathrm{then}\:\mathrm{the} \\ $$$$\mathrm{length}\:\mathrm{of}\:\mathrm{the}\:\mathrm{semi}\:\mathrm{letusrectum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{parabola} \\ $$$$\mathrm{is} \\ $$$$\mathrm{ans}:\mathrm{2} \\ $$

Question Number 48918 Answers: 0 Comments: 0

if r_1 andr_(2 ) are the radii of smallest and largest circles which passes through (5,6)and touches the circle x^2 +y^2 −4x=0 then r_1 r_2 = ans:((41)/4)

$$\mathrm{if}\:\mathrm{r}_{\mathrm{1}} \mathrm{andr}_{\mathrm{2}\:} \mathrm{are}\:\mathrm{the}\:\mathrm{radii}\:\mathrm{of}\:\mathrm{smallest}\:\mathrm{and}\: \\ $$$$\mathrm{largest}\:\mathrm{circles}\:\mathrm{which}\:\mathrm{passes}\:\mathrm{through}\: \\ $$$$\left(\mathrm{5},\mathrm{6}\right)\mathrm{and}\:\mathrm{touches}\:\mathrm{the}\:\mathrm{circle}\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} −\mathrm{4x}=\mathrm{0} \\ $$$$\mathrm{then}\:\mathrm{r}_{\mathrm{1}} \mathrm{r}_{\mathrm{2}} = \\ $$$$\mathrm{ans}:\frac{\mathrm{41}}{\mathrm{4}} \\ $$

Pg 1593 Pg 1594 Pg 1595 Pg 1596 Pg 1597 Pg 1598 Pg 1599 Pg 1600 Pg 1601 Pg 1602

Contact: info@tinkutara.com